Erik D. Demaine, Martin L. Demaine, Anna Lubiw, Arlo Shallit, Jonah L. Shallit
Artists
Erik Demaine and Martin Demaine
Professor and Artist-in-Residence
MIT
Cambridge, MA
Statement
This work is a collaboration involving Erik D. Demaine (MIT), Martin Demaine (MIT), Anna Lubiw (Waterloo), Arlo Shallit, and Jonah Shallit. The goal was to do math with family members: Erik is Martin's son, and Arlo and Jonah are Anna's sons. We were exploring what 3-dimensional shapes can be made from a planar polygon and one zipper. Or, to put it another way, what 3-dimensional shapes can be unzipped to lie flat in the plane without overlap. Felt models with real zippers are a satisfyingly tangible way to appreciate the problem.
Artworks

zipper unfolding of a double pyramid
5" x 5" x 5" (3d); 9" x 15" (2d)
felt with zipper, plus colour photocopy
2010
On the outside, this model is an octahedron. However, it has an extra square face on the inside, so it is really two pyramids glued together on their bases. This two-celled polyhedral complex has a zipper unfolding.
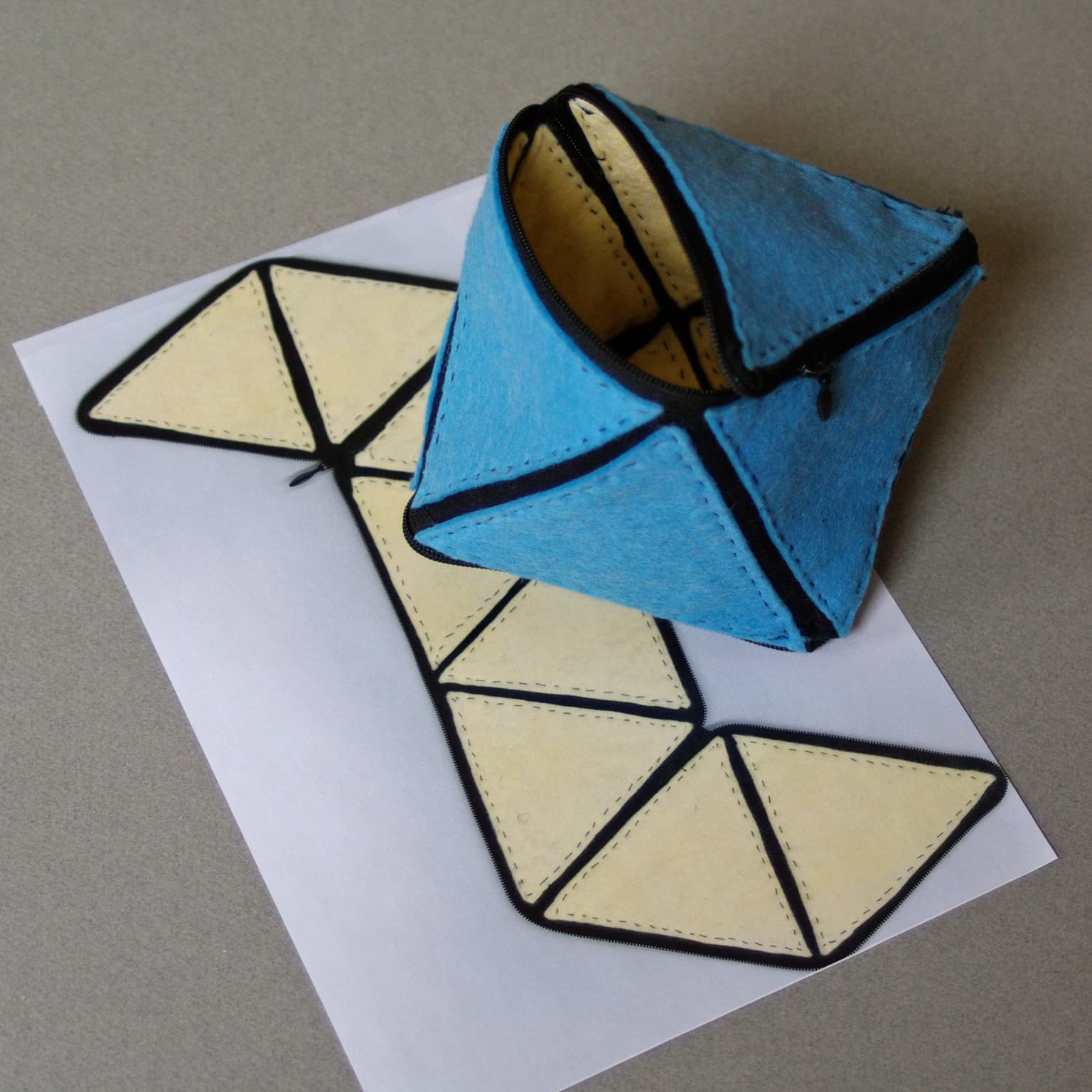
zipper unfolding of an octahedron
5" x 5" x 5" (3d); 12" x 16" (2d)
felt with zipper, plus colour photocopy
2010
An octahedron can be unzipped to a planar polygon.
In fact, all the Platonic and Archimedean solids have zipper unfoldings (http://erikdemaine.org/zippers/).
Can every convex polyhedron be unzipped to a planar shape? If the zipper is restricted to travel along the edges of the polyhedron, then a Hamiltonian path is necessary (though not sufficient) and the answer is "no". The general question is open.