2011 Joint Mathematics Meetings
Juan G. Escudero
Artists
Juan G. Escudero
Statement
Tiling problems have appeared in many branches of mathematics and physics, and during the last few decades there has been much progress in understanding their nature. Research on aperiodic tilings has been very intensive in connection with the field of quasicrystals. In the visual and sound arts, they have potential interest as a system of reference in constrictive preforming for channeling the expressive energies.
Artworks
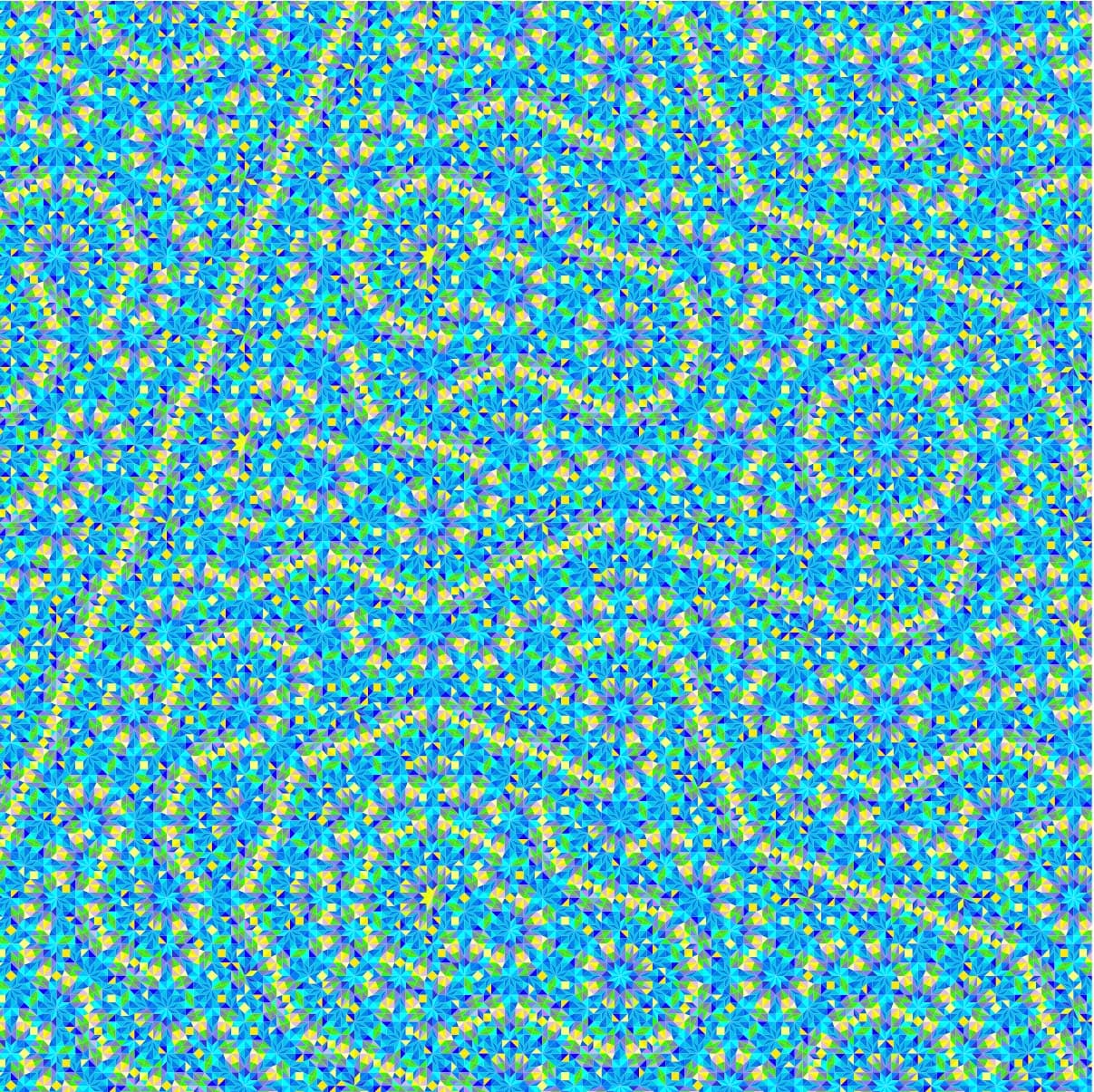
Branched Manifold
20"x20"
Digital Print
2010
A cell complex is defined in the analysis of the cohomology of tiling spaces. It contains a copy of every kind of tile that is allowed, with some edges identified for the 2D case, and the result is a branched surface. When the tiling does not force the border, collared tiles can be used. Here the triangles with the same shape, color and orientation represent the same tile in the complex. The manifold appears in the cohomology computations of an octagonal pattern belonging to a random tiling ensemble introduced by the author in the context of mathematical quasicrystals.