Markus Rissanen
Artists
Statement
Inspired by the famous aperiodic Penrose tiling I started some years ago to study non-periodic rhombic tilings with rotational symmetric properties. I asked myself if there is a simple way to create non-periodic rhombic tilings with infinite number of locally n-fold rotational symmetric areas for other values than n = 5. I managed to construct such a system for all integers n > 4 and my drawings for Bridges 2013 Math Art Exhibition are examples of such tilings.
Artworks
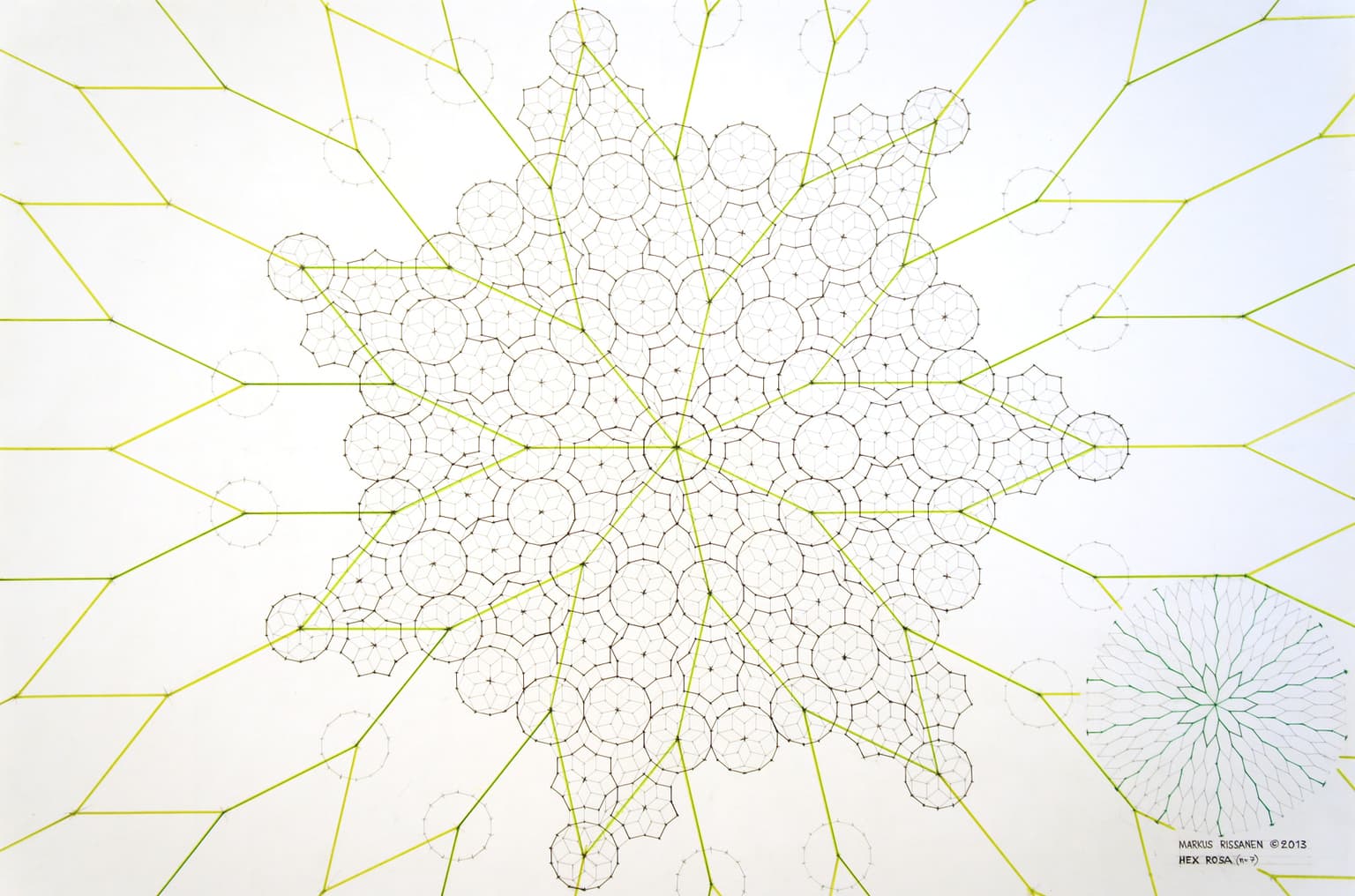
Hex Rosa (n = 7) Rhombic Tiling
60 x 90 cm
pencil and colored pen on paper
2013
Tilings presented here are non-periodic (but not aperiodic), globally n-fold rotational symmetric around one central point and contain infinite number of separate, locally n-fold rotational symmetric circular areas (drawn in black) resembling roses. These “roses” are located around the middle point along curvy circular rings touching each other. As the system utilizes Hexagonal superstructures (drawn in green) and Rose looking structures it is named Hex Rosa. Mere hexagons shown here are able to tile the plane in infinite number of different ways as such, but here they are positioned to allow globally n-fold rotational symmetric tiling for the rhombs. There is a convex “closed” rose in every vertex of every hexagon and a concave “open” rose near the middle point of every edge (drawn in green) of every hexagon. All the edges are identical.
I believe this system of rhombic tilings is previously unknown for mathematicians in the field.