Horst Schaefer
Artists
Statement
I am trying to apply formal concepts from mathematics, logic or science in my work. One of my goals is to reach a balance between these formal aspects, artistic freedom and the resulting aesthetic appearance. Some time ago I discovered the so called Squaring the Square problem. Basically it asks how can one divide a given square into smaller squares. The most demanding case has the additional requirement that all smaller squares are different. The first solution was found 1938 by Roland Sprague. For more information see www.squaring.net. The site is run by Stuart Anderson, it contains a wealth of further information about this topic.
Artworks
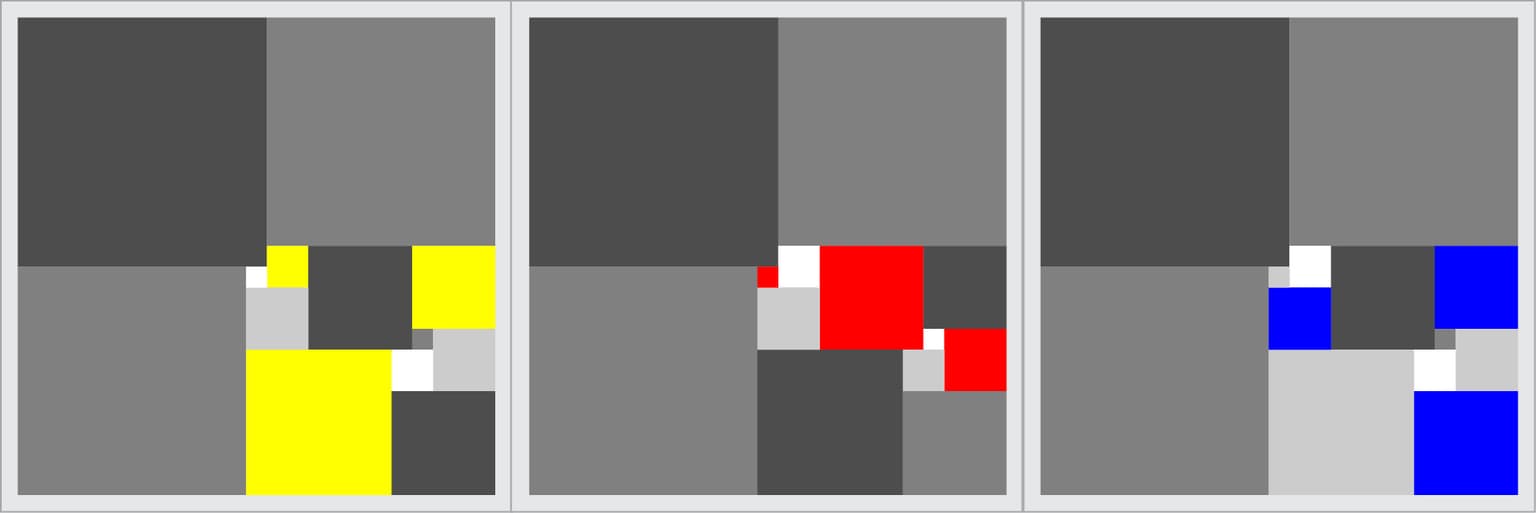
SISS order 13 – Yellow-Red-Blue Triptych
90 cm x 30 cm
Acryl on cancas
2012
SISS stands for Simple Imperfect Squared Square. The tiling is called imperfect because smaller squares may be equal, however they are not allowed to form sub-rectangles. The solution with 13 squares is the smallest solution. It was probably found in 1946 by C.J. Bouwkamp (a mathematician born 1915 in Netherlands and living until 2003).

CPSS order 24 – Yellow-Red-Blue Mondrian Style
90 cm x 90 cm
Acryl on cancas
2013
CPSS stands for Compound Perfect Squared Square. The smaller square are all unequal, however a subset of the squares can form a rectangle or a square (therefore compound). The solution with 24 squares is the minimal solution for this type. It was found 1948 by T.H. Willcocks, (an amateur mathematician born 1912 in England)
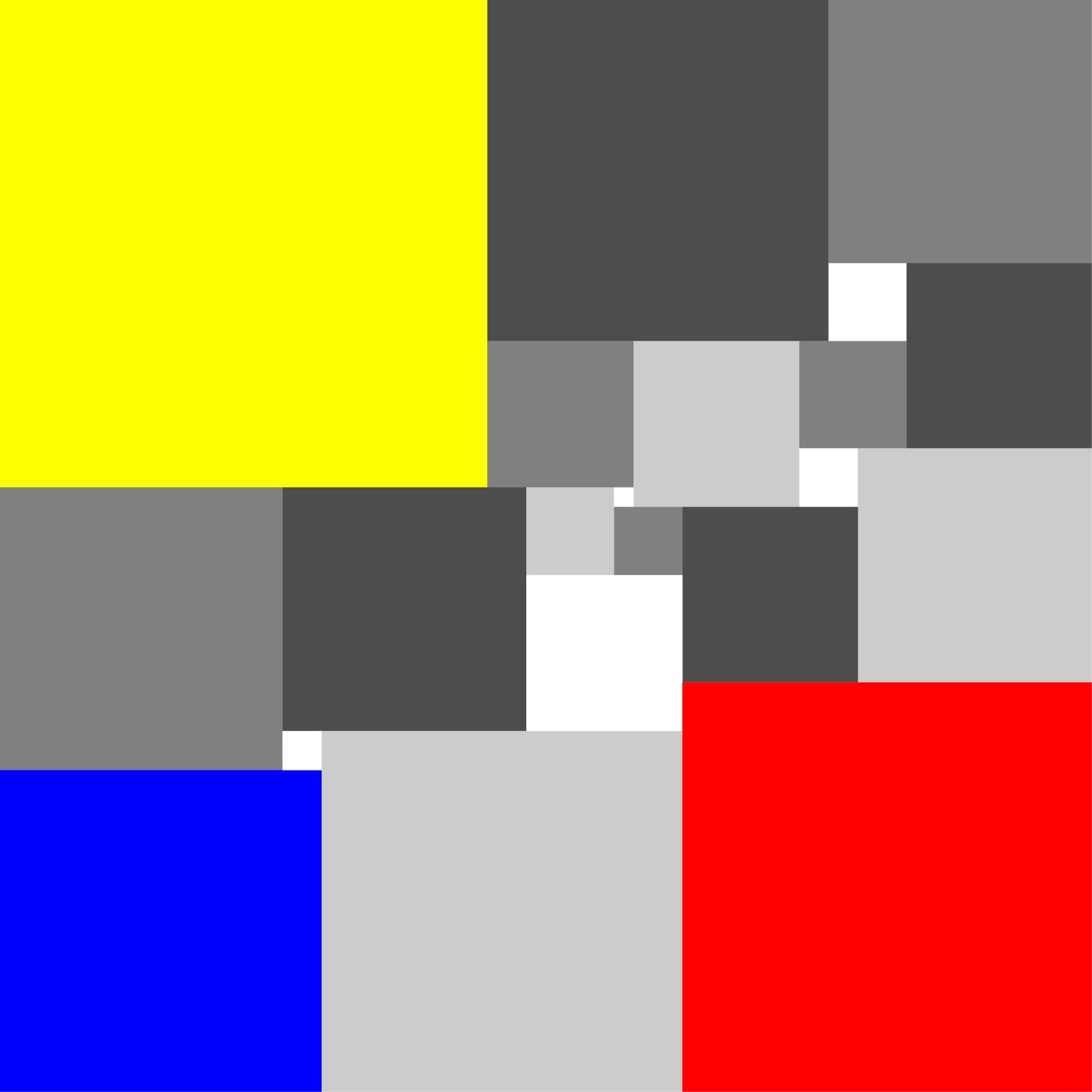
SPSS order 21 – Yellow-Red-Blue Mondrian Style
60 cm x 60 cm
Acryl on canvas
2013
SPSS stands for Simple Perfect Squared Square. The square shown is divided into 21 smaller squares, such that all smaller squares are unequal. This solution was found in 1978 by A.J.W. Duijvestijn (a mathematician born 1927 in the Netherlands and living until 1998). He also proved that this solution is unique and that it is the minimal solution.