2013 Joint Mathematics Meetings
Anne Burns
Artists
Anne Burns
Professor Emerita (Mathematics)
Long Island University
Huntington, New York, USA
Statement
For many years I have been fascinated by the connections between art and mathematics. I teach mathematics and always search for a visual interpretation of each topic. My students are always amazed and pleased at how a visual representation of a concept results in a better understanding and sometimes a path to discovery.
Artworks
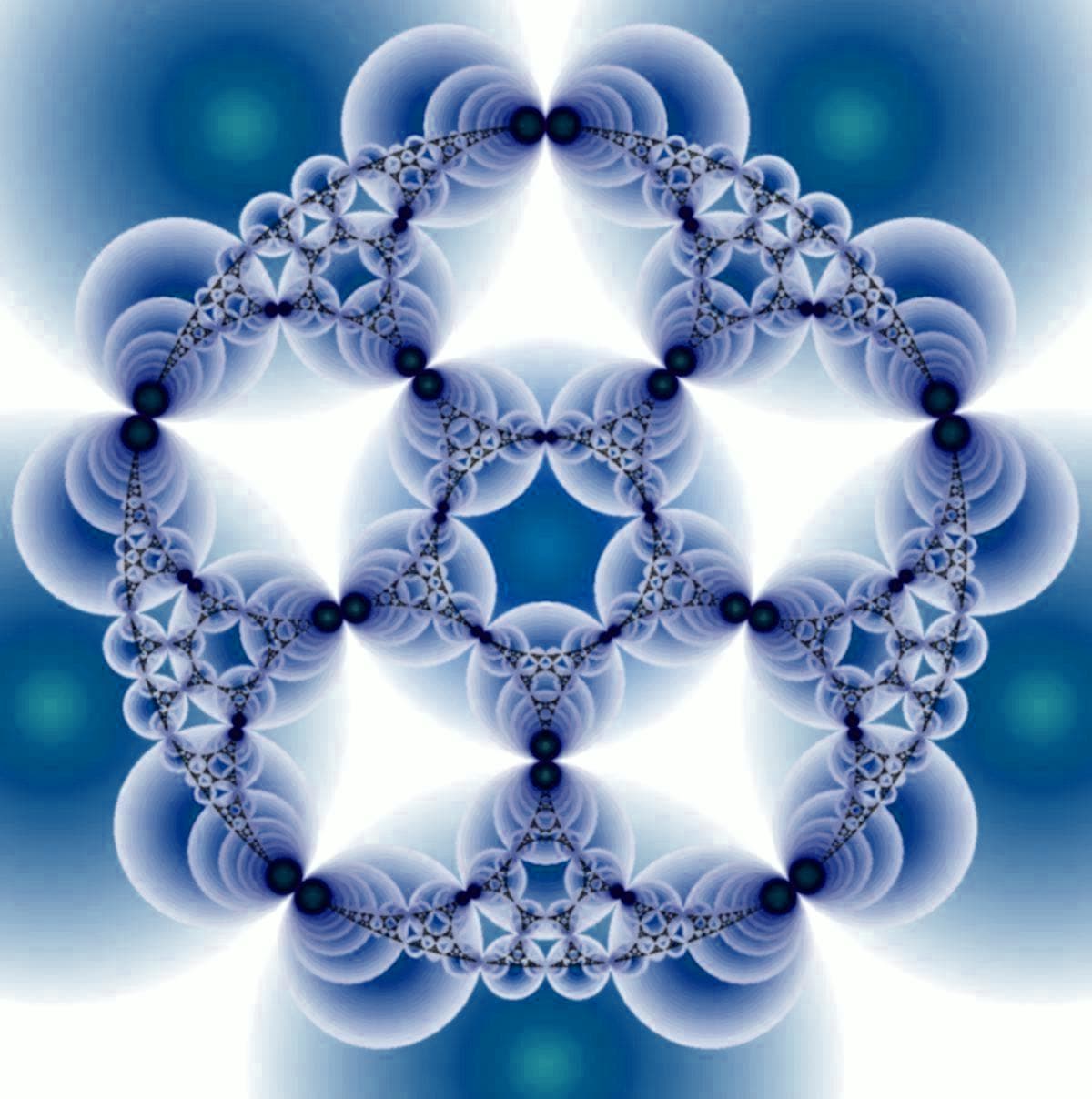
InversionsFive
12"x12"
Digital Print
2012
Five pairwise tangent circles are all tangent to a sixth circle centered at the origin. The discs bounded by these six circles are colored in blue-green. An iterated function system is made up of repeated inversions in the six circles.
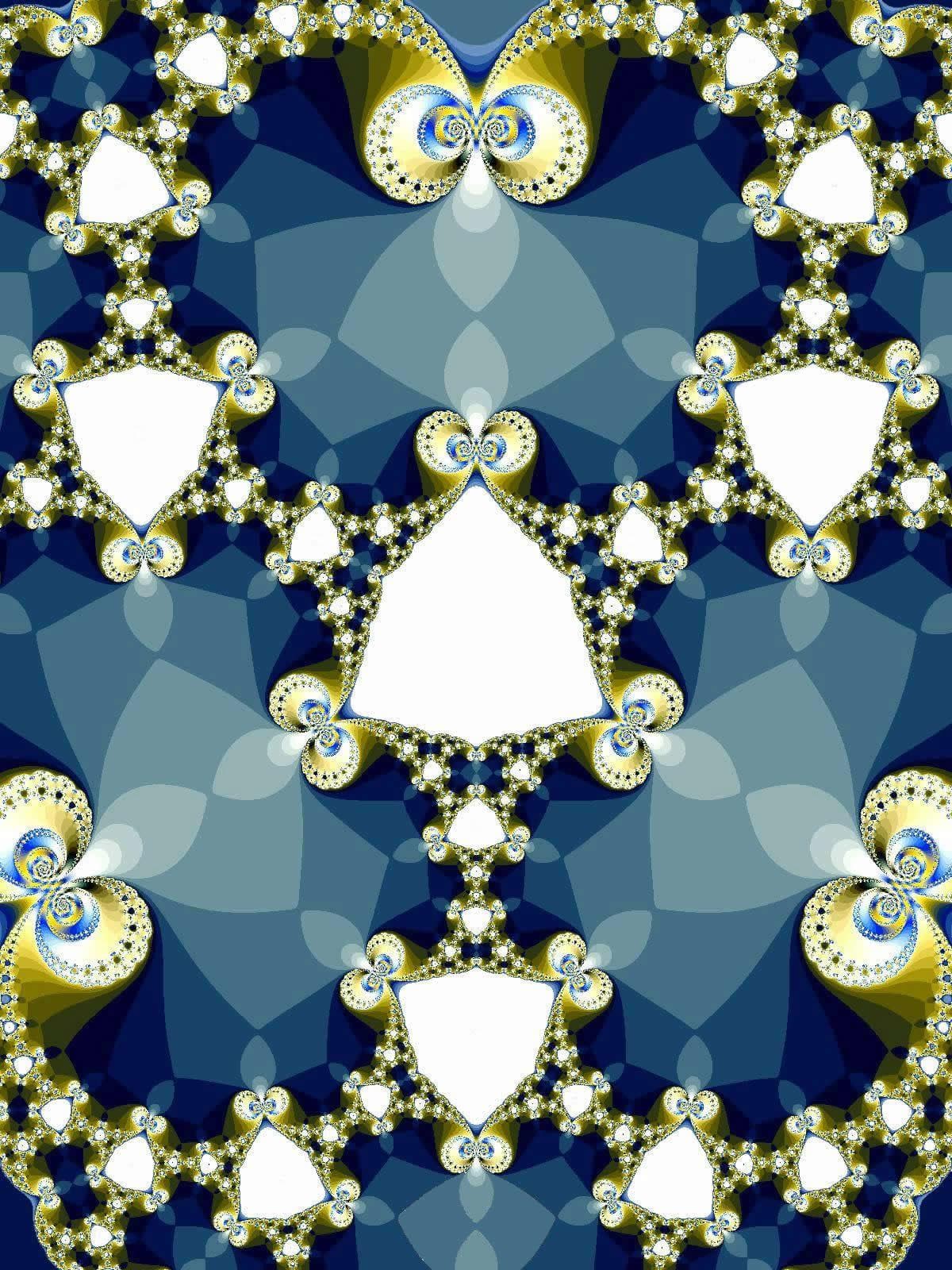
Blue Julia
19" by 13"
Digital print
2012
This graphic is a rendering of the Julia Set obtained by iterating the rational function, f(z) = z^2+c/z. An open set containing the origin maps to the immediate basin of attraction of ∞. This set is the white area in the center of the picture. Why does the picture exhibit a “three-ness”? By writing the function as (z^3+c)/z it is easy to see that this white region has three pre-images, and each of those has three pre-images, etc.; these regions are colored white. This function also has three fixed points, and the backward iterates of those points never leave the region; those points are the centers of the 3 largest spirals and the smaller spirals are pre-images of those. The picture has been rotated by 90 degrees to render the symmetry more attractive.