2013 Joint Mathematics Meetings

Chern Chuang, Bih-Yaw Jin and Chia-Chin Tsoo

Artists

Bih-Yaw Jin

Professor

Department of Chemistry, National Taiwan University

Taipei, Taiwan

byjin@ntu.edu.tw

Statement

Using the beaded realization of three canonical triply periodic minimal surfaces which are frequently encountered in material and biological sciences, we demonstrated at last year's exhibition that mathematical beading applies to the simulation of chemical structures. We show here a beaded sculpture of Hilbert's space-filling curve, a purely mathematical object, made with the same methodology. The so called right-angled weaving technique proves to be a reliable way of constructing three-dimensional objects provided that appropriate designs of the structures are given. The resulting artworks are of considerable interest with regard to both mechanical stability and aesthetics.

Artworks

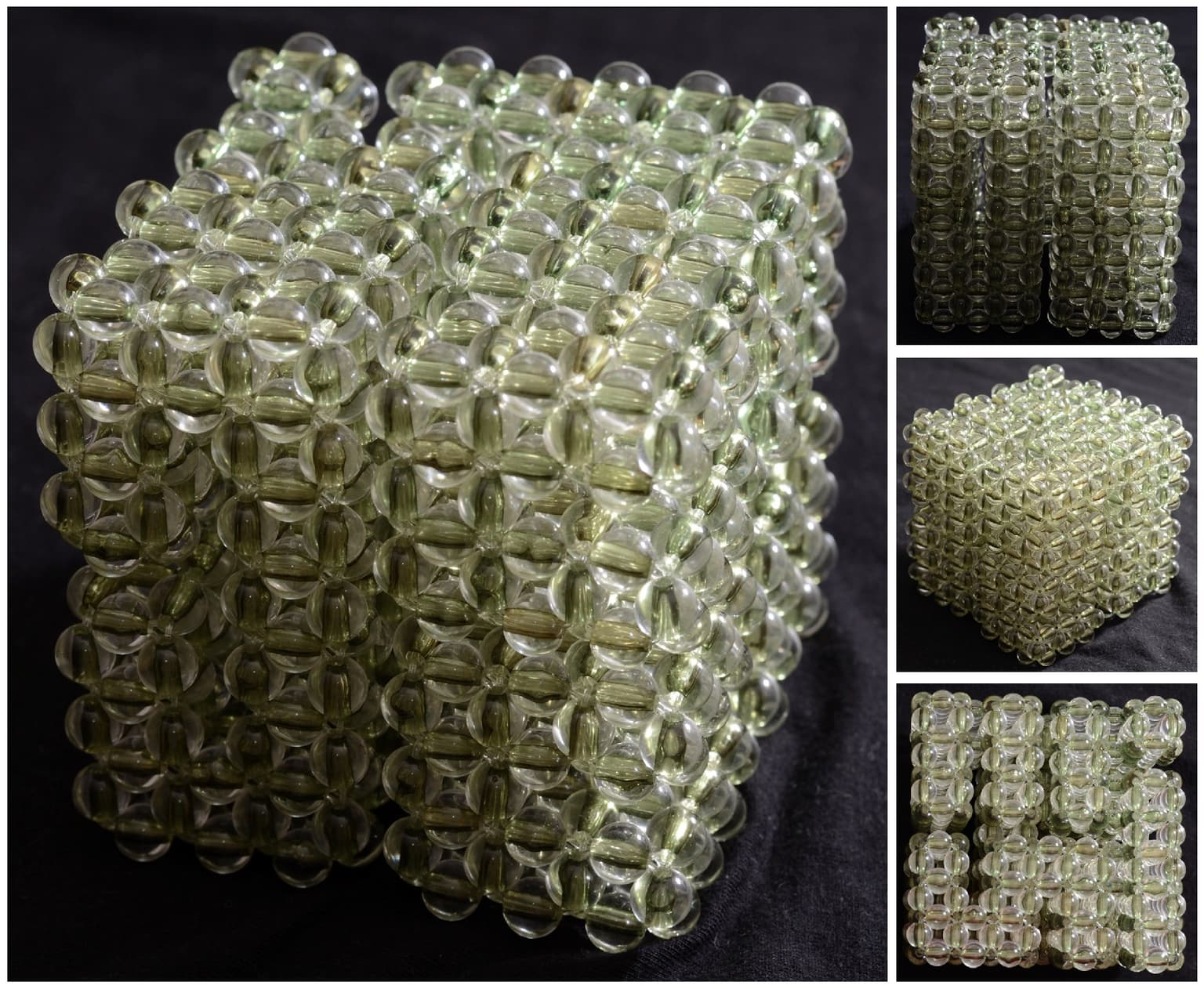
Image for entry 'Beaded Hilbert Curve, Step Two'

Beaded Hilbert Curve, Step Two

12cm x 12cm x 12cm

Double layered plastic beads with fish lines.

2012

Hilbert curve is an example of space-filling curves, a fascinating family of curves that pass through every point in space. In particular, because of its locality preserving property, the Hilbert curve finds wide application in the field of computer science. Here we demonstrate that the right-angled weaving technique of beading can be suitably used to construct models of these curves. The particular example we choose is the second step of a three dimensional Hilbert curve composed of 127 beaded cubes. Although the structure has weak connectivity, since essentially every segment is only connected to its two neighbors as compared to that of the simple cubic lattice (six), the mechanical stability of the physical model is considerably strong. This serves to illustrate beading as a systematic tool of understanding and creating mathematical figures, which features a hands-on and sequential construction scheme.