2013 Joint Mathematics Meetings
Hartmut F. W. Höft
Artists
Hartmut F. W. Höft
Professor of Computer Science, emeritus
Eastern Michigan University
Ann Arbor, Michigan, USA
Statement
The sickle of the moon can be drawn with two ellipses in the plane and a pair of sickles with two orthogonal ellipses. I asked myself what other designs I might be able to create with such pairs as the only geometric element in the plane. With my images I want to demonstrate to the viewer the conundrum of the easy and the difficult, the simple and the complex. The image is based on two fundamental geometric elements: a curve in the plane that provides the "spine" of a figure in an image and pairs of orthogonal ellipses that are placed at regular intervals along the spine and that create the shape in the image. The spine is not drawn, only the ellipses are drawn. I render my images in Mathematica. What surprised me most, however, is that many of the resulting images appear three-dimensional. Our visual system apparently gets fooled into seeing a three-dimensional object when we draw many ever smaller slices after larger ones in a single plane. All images are mathematically planar.
Artworks
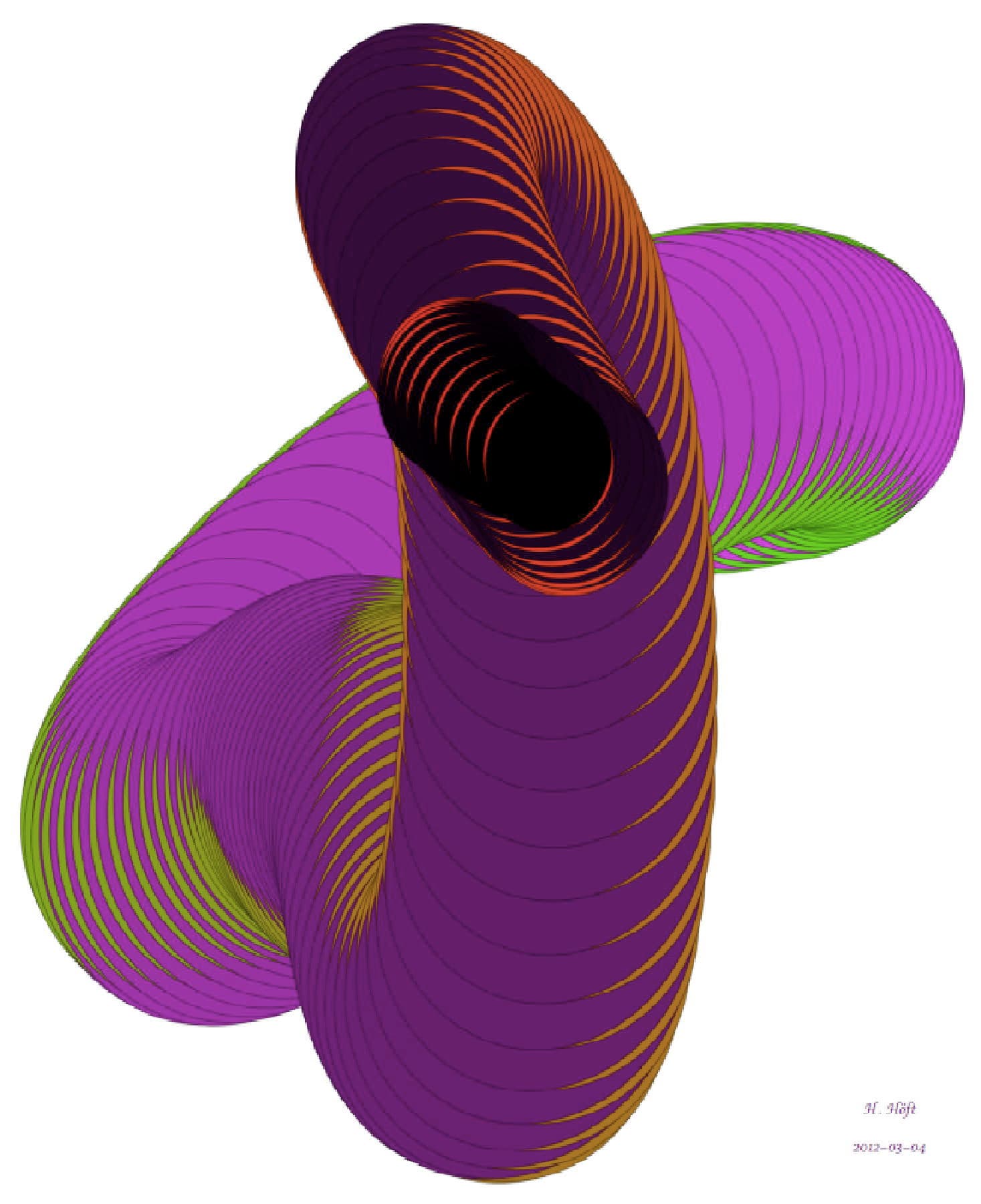
Purple Tubeworm Rearing Up
8.5 x 11 (16 x 17.25 framed)
color print on archival paper
2012