2013 Joint Mathematics Meetings
Harry Rubin-Falcone
Artists
Harry Rubin-Falcone
Student
Math Department, Oberlin College
Oberlin, OH
Statement
My work takes simple mathematical ideas and uses them to create complex figures, which I then present in a simple way. I'm fascinated by how mathematical figures are often strongly reminiscent of nature and try to showcase that in my work. While some of my pieces are fractal models of natural objects, others do not resemble anything found in nature at all, but I try to instill an organic feel in them all the same.
Artworks
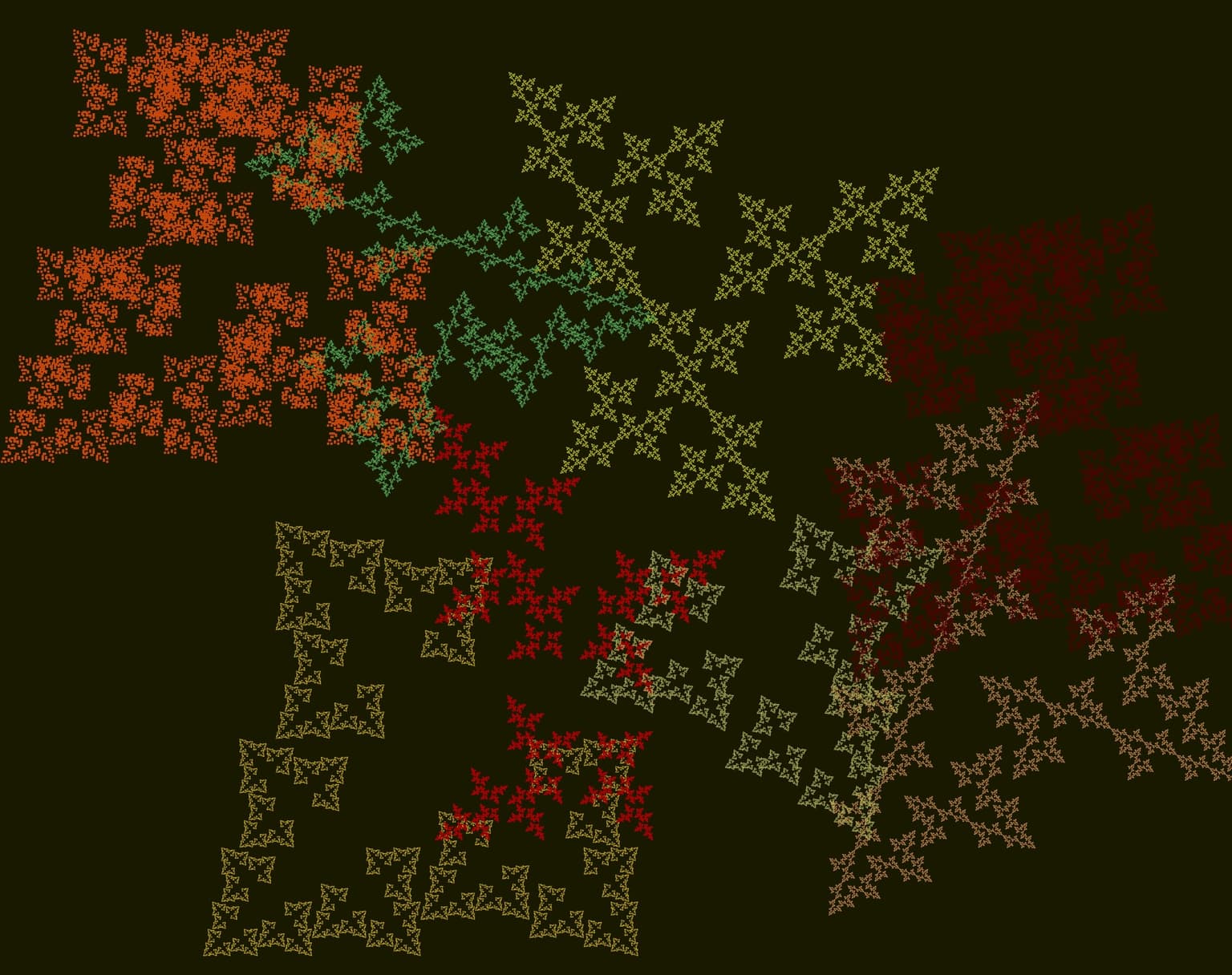
Affine Autumn
12" x 15"
Digital Print
2012
A few different simple iterated function systems, positioned and colored to resemble fallen leaves. Each leaf is created with a series of affine transformations.
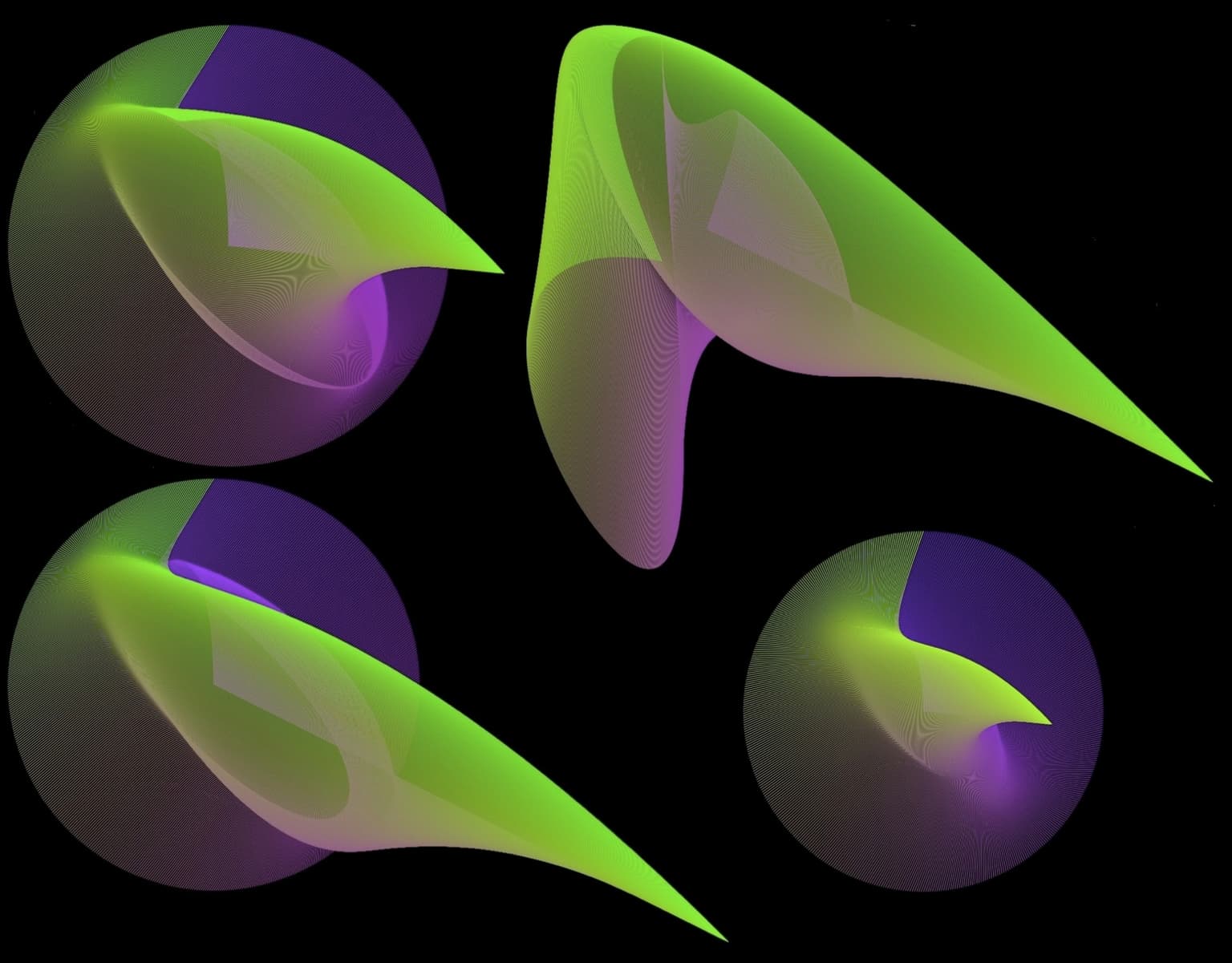
Pointed Planes and Bézier Beaks
15.5"x20"
Digital Print
2012
Each figure is created with a series of Bézier curves. Because there is space between each curve, you can see some parts of the curves behind the others, which gives each figure a translucent and three dimensional look. The curves can be thought of as lying on a plane, which means each figure is a representation of a folded-over plane that comes to a point.