2013 Joint Mathematics Meetings
Robert Spann
Artists
Statement
I have always been fascinated with the behavior of dynamical systems -- be they mathematical, physical, or financial. Where do different points end up and how do they get there? Do they reach an equilibrium or do they continue moving randomly forever? Computer graphics allows one to see both the numerical and aesthetic properties of these systems. Recently I became interested in the properties of complex functions in which a complex variable is raised to a complex, rather than an integer exponent. I have also been analyzing complex polynomials that have no attracting fixed points.
Artworks
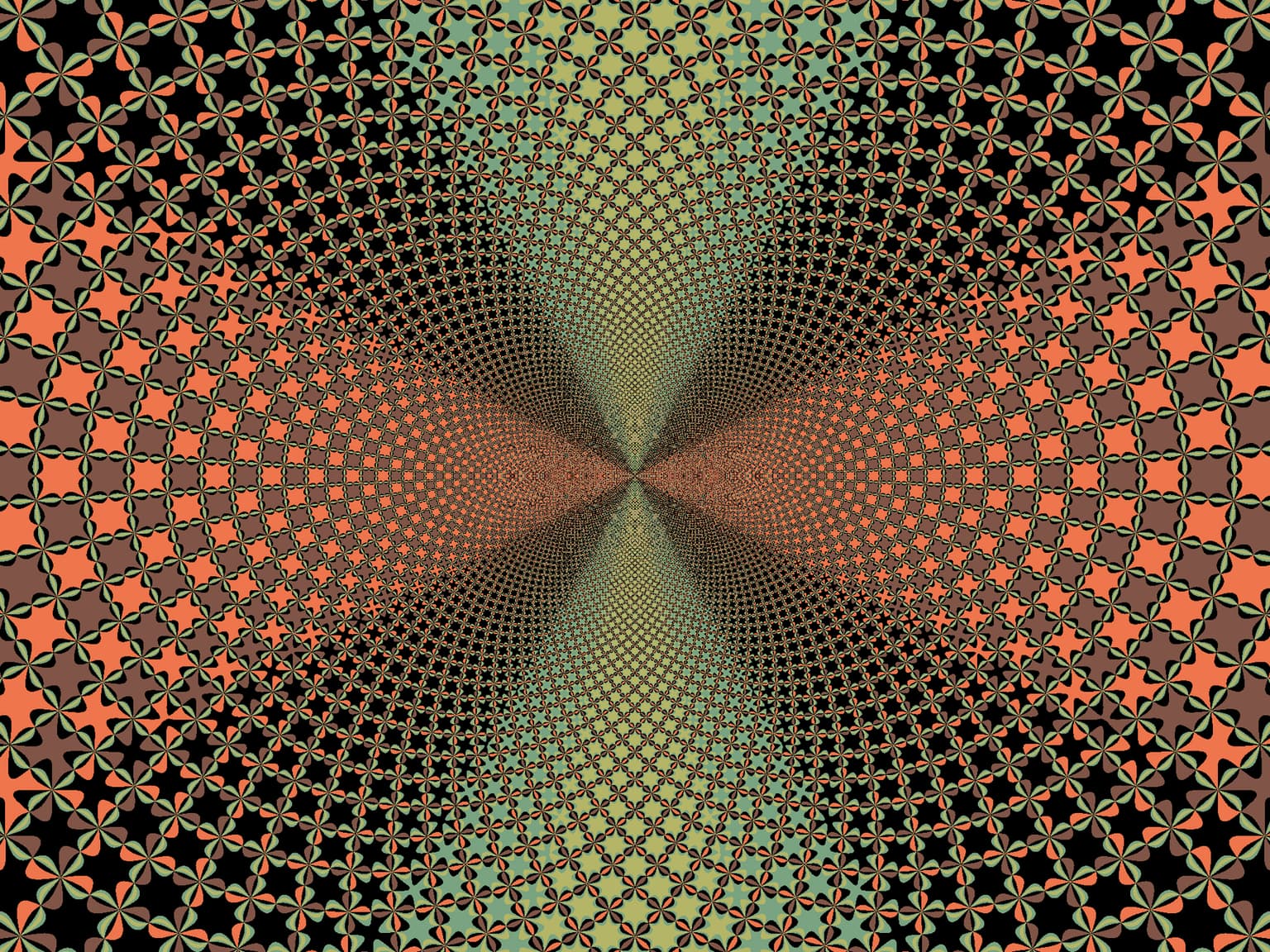
Star Wheel
11" x 14" including frame
Digital Print
2009
This image is produced by iterating the complex polynomial (z^2-2)/z^2. This complex polynomial has no attracting fixed points in the complex plane.

Convergence?
11" x 14" including frame
Digital Print
2012
This image is produced by applying Newton's method for root finding to the complex function (z^(2+3i)-.09)*(z^(2-3i) -.09).The white areas are points in the complex plane where this function does not converge to any root. The background is produced using Perlin noise functions.