2014 Bridges Conference Short Movie Festival

Russell Lyons

Filmmakers

Russell Lyons

Professor of Mathematics

Dept. of Math., Indiana University

United States

rdlyons@indiana.edu

http://pages.iu.edu/~rdlyons

http://pages.iu.edu/~rdlyons/rw/rw.html

Statement

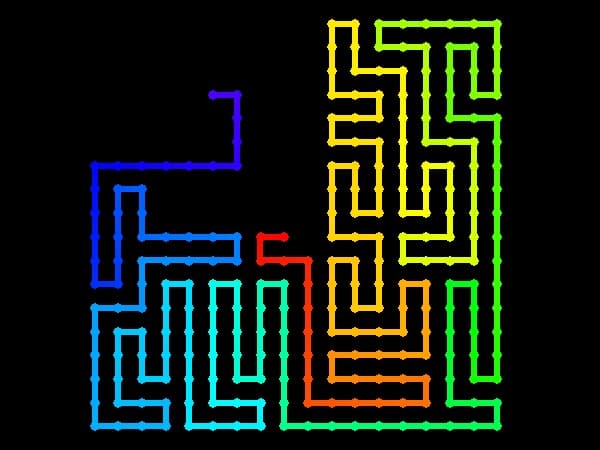
The video "UST Peano Curve" gives pleasure by musical, visual, and intellectual means. A fascinating interaction of high randomness with simple constraints yields surprisingly satisfying music. In June 2010, I used Mathematica to give a uniformly random spanning tree (UST) of a 9-by-9 grid in the plane. There are 8,326,627,661,691,818,545,121,844,900,397,056 spanning trees of the 9-by-9 grid. Surrounding the spanning tree by a curve, one gets a random Peano-like curve that visits each lattice point exactly once in an 18-by-18 region before returning to its starting point. The coordinates of the curve were converted to pairs of notes in the A major scale. The music and the color simply trace the curve. USTs and their Peano curves are elements of contemporary mathematical research. Mathematica was used to make the video and to convert the coordinates to notes, which were turned into Midi by Midge. The video and audio were combined by ffmpeg.

Films

Image for entry 'UST Peano Curve'

UST Peano Curve

00:01:23

Russell Lyons

2010

Watch

Originally inspired by amazing graphics and videos at a math conference I attended, I decided to illustrate some basic concepts in my research area, both for the book I am writing and in order to increase interest in mathematics among students. After making some such graphics and small animated gifs, I realized that music could help make vivid the motion of random walks. I chose a very simple and natural way to turn walks into music. Surprisingly, no one had done this before. Even more astonishing was that the music was pleasing as music. Thus, I extended this idea and turned the Peano curve surrounding a UST into music in the same way. Hue, a circular color scale, was used to illustrate visually the progress along the closed curve, matching the musical progress. A score is at http://pages.iu.edu/~rdlyons/rw/peano9.pdf. A limit of the Peano curve as the mesh tends to 0 is a random space-filling curve that is described by the Schramm-Loewner Evolution, a major part of probability.