David Chappell
Artists
David Chappell
Professor of Physics
University of La Verne
La Verne, California, USA
Statement
Mathematical art gives me the opportunity to create my own universe. I design mathematical and computational rules to explore the production of complex spatiotemporal patterns. In some sense, I consider my art form to be the act of creating rules. Sometimes I adopt a “hands-off” approach and let my universe unfold undisturbed once the rules have been set in motion. Other times I meddle: tweaking, reordering, organizing. In either case, the aim of these explorations is to both generate aesthetically compelling compositions and to better understand the process of pattern formation in dynamical systems.
Artworks
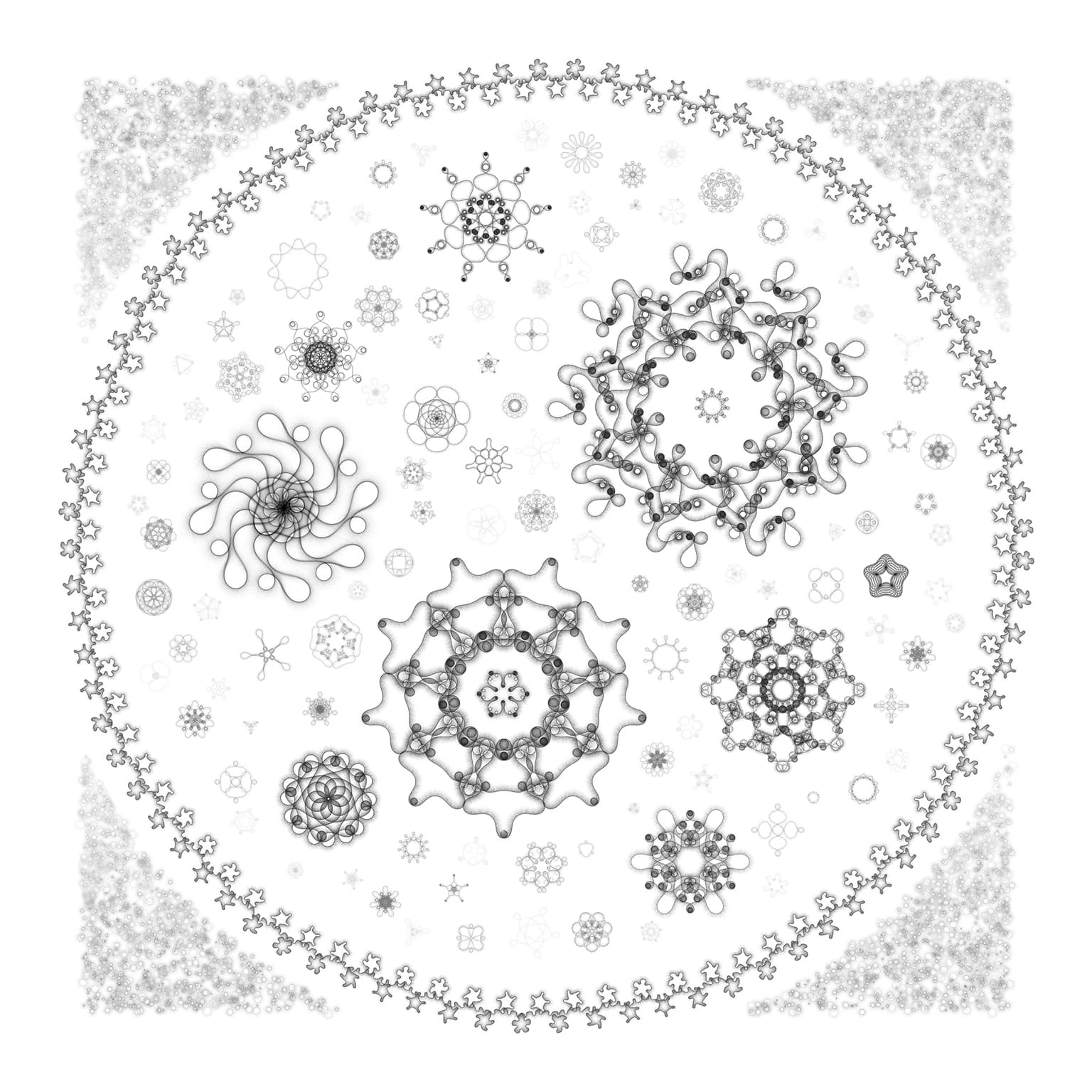
Topos Hyperuranios
20" x 20"
Archival Inkjet Print
2014
“Topos Hyperuranios” (the place beyond the heavens), is a star chart in an imagined universe of pure form. Each “galaxy” is constructed from a single closed curve in which the tangential angle of the curve is expressed as the sum of two sine terms that are functions of arc length. I refer to such curves as sinuous meander patterns. An additional linear term is included to wrap the curves into rotationally symmetric designs. Each structure has between 3- and 11-fold rotational symmetry. Most have reflection symmetry as well. I find it remarkable that a single underlying equation can produce such richly diverse forms.