Bih-Yaw Jin & Chia-Chin Tsoo
Artists
Bih-Yaw Jin
Professor
Department of Chemistry, National Taiwan University
Taipei, Taiwan
Statement
Previously, we have applied the angle weave technique with spherical beads to the construction of physical models for arbitrary fullerenes and graphitic structures. Spherical beads are more suitable for trivalent systems, which are exactly molecular systems with sp2 hybridization. Here, we show that beaded sculptures consisting of higher valencies through linking rigid polyhedral units, such as tetrahedra and octahedra, can be achieved by using tubular beads and the same beading technique.
Artworks

Beaded Mackay icosahedron with chiral vector (4,0)
18x18x18 (cm)
3cm tubular Glass beads
2014
Mackay icosahedron is a type of building units of the quasi-crystal, a structure that is ordered but not periodic due to the incompatibility between the five-fold rotational symmetry and the translational symmetry. We built this icosahedron by linking tetrahedra, octahedra, and pentagonal bipyramids together using the angle weave technique. In this sculpture, tetrahedra and octahedra fill its 20 faces and pentagonal bipyramids become its 12 apexes.
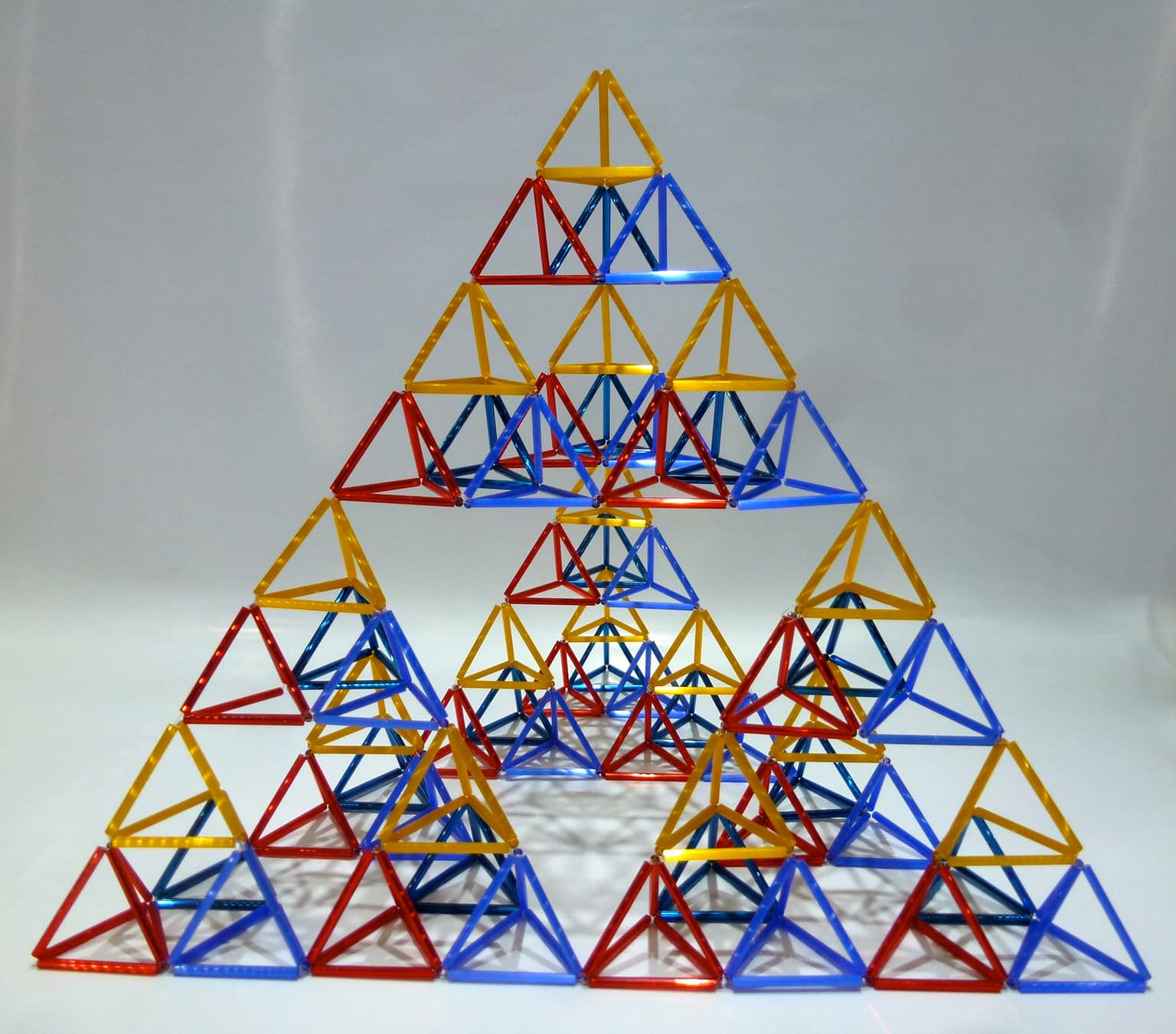
Beaded Sierpinski tetrahedron
20x20x20 (cm)
3cm tubular Glass beads
2014
The third-order bead model of Sierpinski tetrahedron consists of 64 small tetrahedra and is about 24 cm along its edges. It is made from 256 3cm tubular beads using the angle weave technique repeatedly.