Samuel Monnier
Artists
Samuel Monnier
Postdoc
Institut für Mathematik, Zürich University
Zürich
Statement
My recent work explore the visual richness of patterns obtained from conformal maps of the sphere admitting a dense set of chaotic orbits. When the orbits are colored according to certain of their properties, mesmerizingly complex patterns appear, characterized by dense fractal structures. My work explores this inexhaustible source of beauty. The works displayed here are associated to a Lattès map, obtained from an affine map of a branched covering of the sphere by a torus. These types of maps, studied by the french mathematician Samuel Lattès in 1918, were the first to be recognized to have dense chaotic orbits.
Artworks

The Lattès map z -> (z^3+a)/(az^3+1), with "a" a cubic root of the identity, is a conformal map of the Riemann sphere to itself. It has the property that the orbit of almost every point is chaotic. The pattern displayed in this work is created by coloring each point according to the mean distance of its orbit to given points of the Riemann sphere. The sphere is projected on the complex plan stereographically, and only part of it is shown in the picture.
The pattern has the interesting property that it display dense fractal structure: no matter where one looks structures, appear at all scales.
Edition: unique museum-quality print.
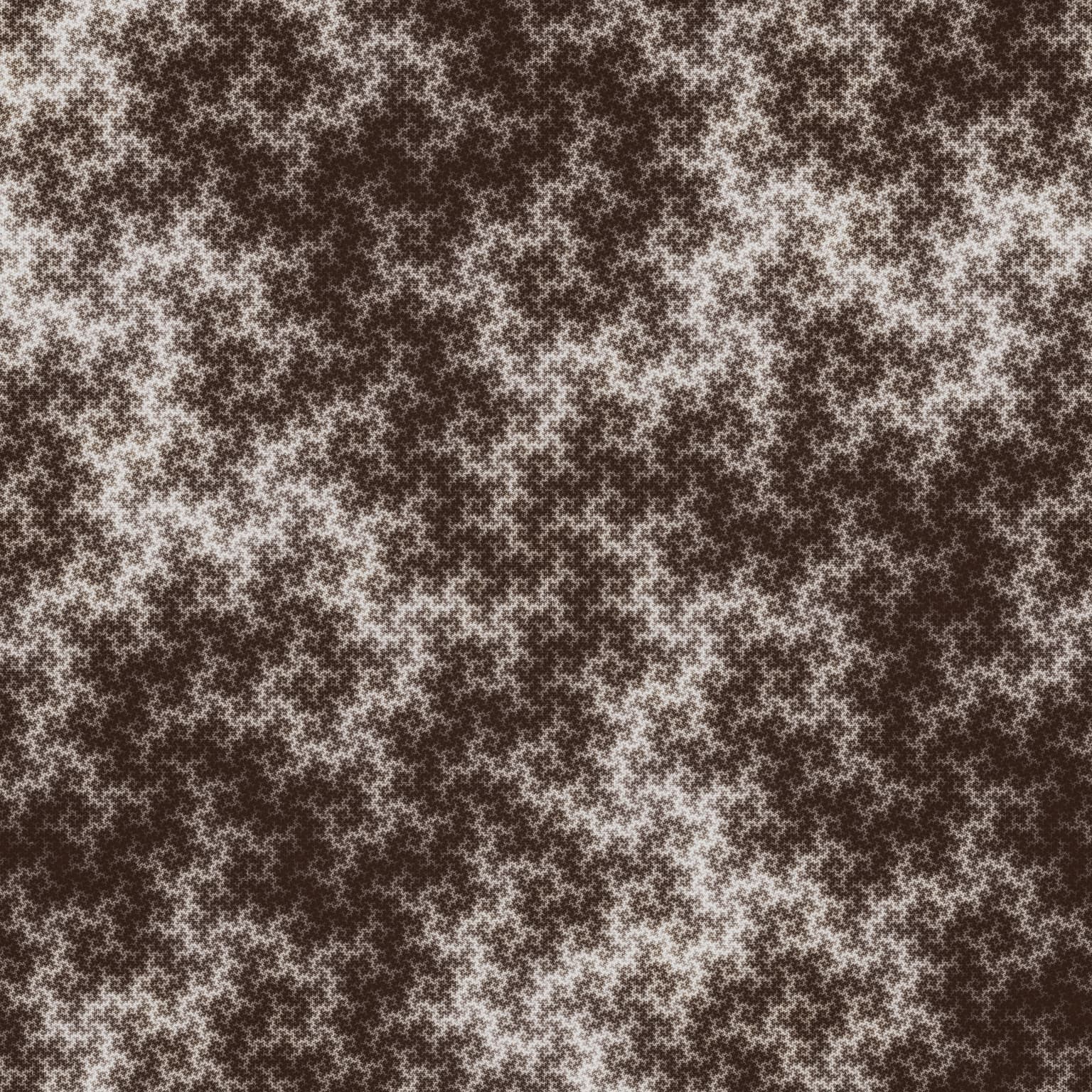
The Lattès map z -> (z^3+a)/(az^3+1), with "a" a cubic root of the identity, is a conformal map of the Riemann sphere to itself. It has the property that the orbit of almost every point is chaotic. The pattern displayed in this work is created by coloring each point according to the mean distance of its orbit to given points of the Riemann sphere. The sphere is projected on the complex plan stereographically, and only part of it is shown in the picture.
The pattern has the interesting property that it display dense fractal structure: no matter where one looks, structures appear at all scales.
Edition: unique museum-quality print.

The Lattès map z -> (z^3+a)/(az^3+1), with "a" a cubic root of the identity, is a conformal map of the Riemann sphere to itself. It has the property that the orbit of almost every point is chaotic. The pattern displayed in this work is created by coloring each point according to the mean distance of its orbit to given points of the Riemann sphere. The sphere is projected on the complex plan stereographically, and only part of it is shown in the picture.
The pattern has the interesting property that it display dense fractal structure: no matter where one looks, structures appear at all scales.
Edition: unique museum-quality print.