Vincent J. Matsko
Artists
Statement
Color, texture, geometry: these drive my work. Pure structure and color are clean, beautiful; playing with structure and color engages the eye and activates the mind. My computer-generated work is inspired by two questions: How would I have developed older ideas if I had a computer rather than just paper, pencil, pen? What is computer art? (That is, what effects can be created with a computer which would be virtually impossible to create otherwise?) Randomness is key: tens or hundreds of thousands of closely related objects combine to create textures difficult to produce otherwise. The randomness is tightly controlled: structured chaos. The challenge? Art as structured randomness.
Artworks

Four to One is based on a dissection of four squares to one. Squares of sizes 15, 36, 48, and 64 may be dissected into pieces and arranged to form a square of size 89, illustrating that 15^2+36^2+48^2+64^2=89^2. Rather than creating five different squares, suggestions of the four component squares are created by overlaying smaller circles (with random radii and colors) on top of the larger circles. As a result, the original dissection on which the image is based can be found within the square of size 89. A close examination of the image reveals 89 small circles on each side, with dark green (size 15), yellow (size 36), brown (size 48), and light green (size 64) squares easily discernible. The image was created using Mathematica.
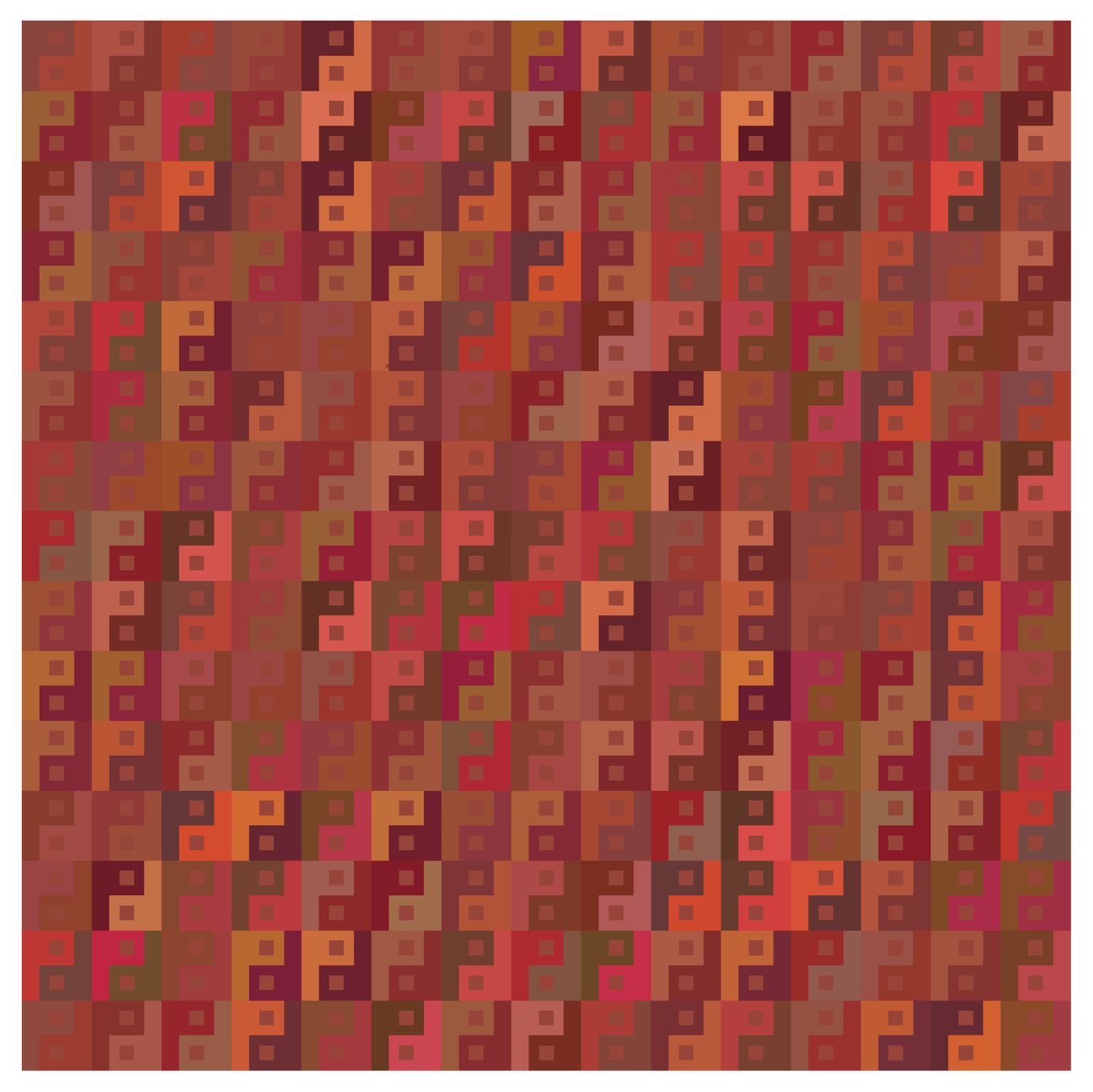
In Yin Yang IV, the use of color was influenced by the color studies of Josef Albers. The smallest squares are all the same color. In the smaller squares (each containing a "p'' and "d'' figure), when the CMYK values for the "p'' and "d'' are numerically averaged, the common color of the smallest squares is obtained. Use of a random number generator determines how far the colors of the "p'' and "d'' are from that of the smallest squares. For example, suppose the smallest squares have CMYK values c=(0.4,0.5,0.6,0.2). A vector of random numbers between 0 and 0.2 is generated, say r=(0.03,0.15,0.1,0.08). Then in one of the smaller squares, the "p'' figure may be colored with c-r, while the "d'' figure may be colored with c+r.