2014 Joint Mathematics Meetings
Robert Fathauer
Artists
Robert Fathauer
Owner
Tessellations
Apache Junction, Arizona, USA
Statement
I'm endlessly fascinated by certain aspects of our world, including symmetry, chaos, and infinity. Mathematics allows me to explore these topics in distinctive artworks that I feel are an intriguing blend of complexity and beauty. The laws that govern our physical universe can be succinctly expressed by mathematical equations. As a result, mathematics can be seen throughout the natural world, and much of my work plays on mathematical forms in nature.
Artworks
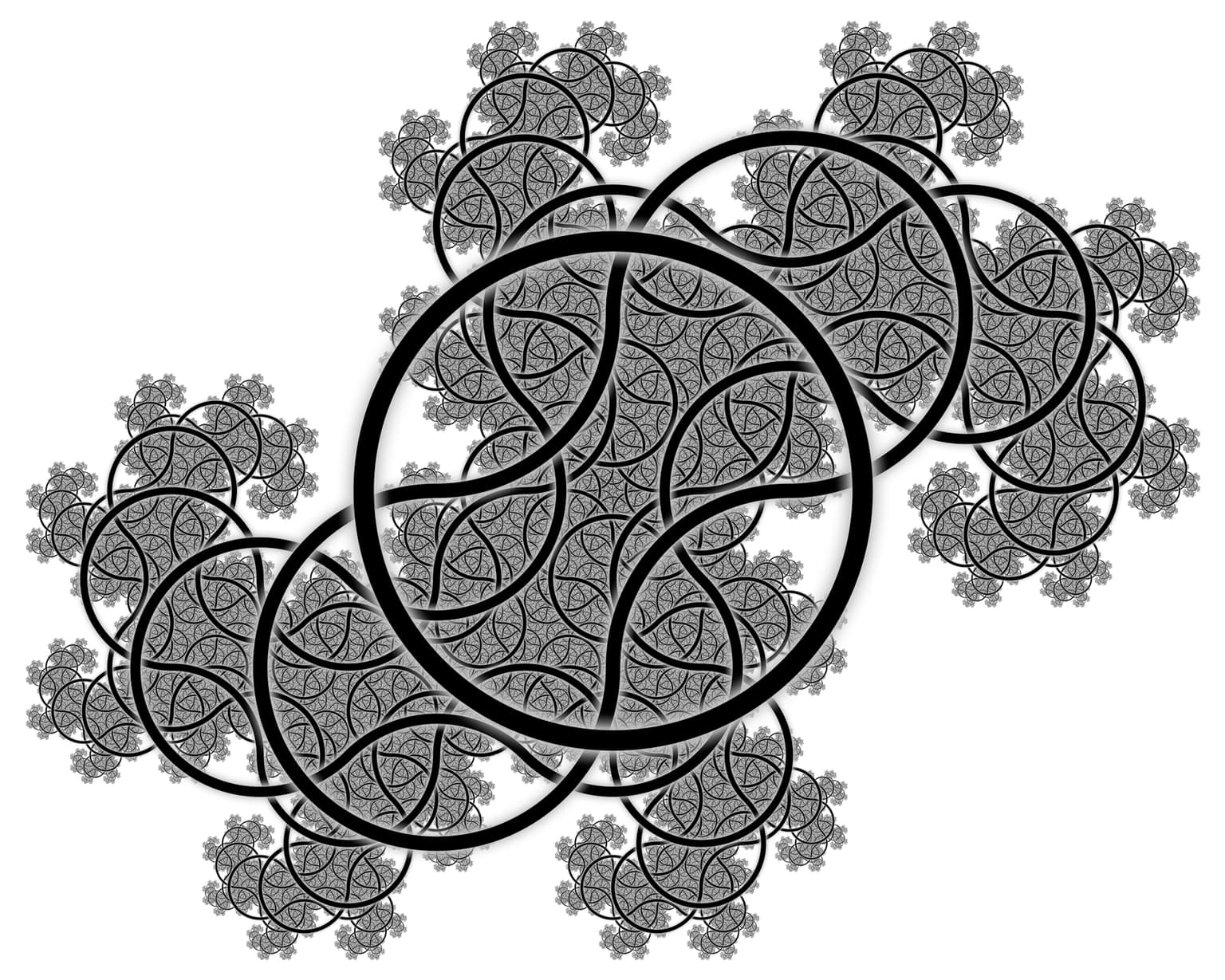
A curvilinear closed form of the dragon curve is depicted (a "twindragon"). The first generation is a circle, and each iteration possesses two-fold rotational symmetry. Curves are shown through 19 iterations.

This sculpture is based on the first five generations of a fractal curve. The starting point is a circle, and the first iteration produces a three-lobed form. With each iteration, the number of lobes is tripled. The spacing between features is essentially constant throughout a layer, while the three-fold symmetric boundary of the curve becomes increasingly complex. A hexagonal version of this curve is found in Benoit Mandelbrot's book "The Fractal Geometry of Nature". This hyperbolic surface is reminiscent of naturally-occurring corals. It was inspired in part by a 3D-printed model created by Henry Segerman.