2014 Joint Mathematics Meetings
Samantha Pezzimenti
Artists
Samantha Pezzimenti
Graduate Student of Mathematics
Mathematics Department, Bryn Mawr College
Bryn Mawr, Pennsylvania
Statement
As a mathematics graduate student and future educator, I am interested in the ways artistic representations and physical models can help students understand abstract mathematical concepts. Legendrian knots are usually represented by their front projection and the corresponding three-dimensional object can be difficult to visualize. This sculpture provides a clearer visualization of the Legendrian unknot and its underlying contact structure. The image shown of the front view reveals how the three dimensional knot is projected onto the xz-plane.
Artworks
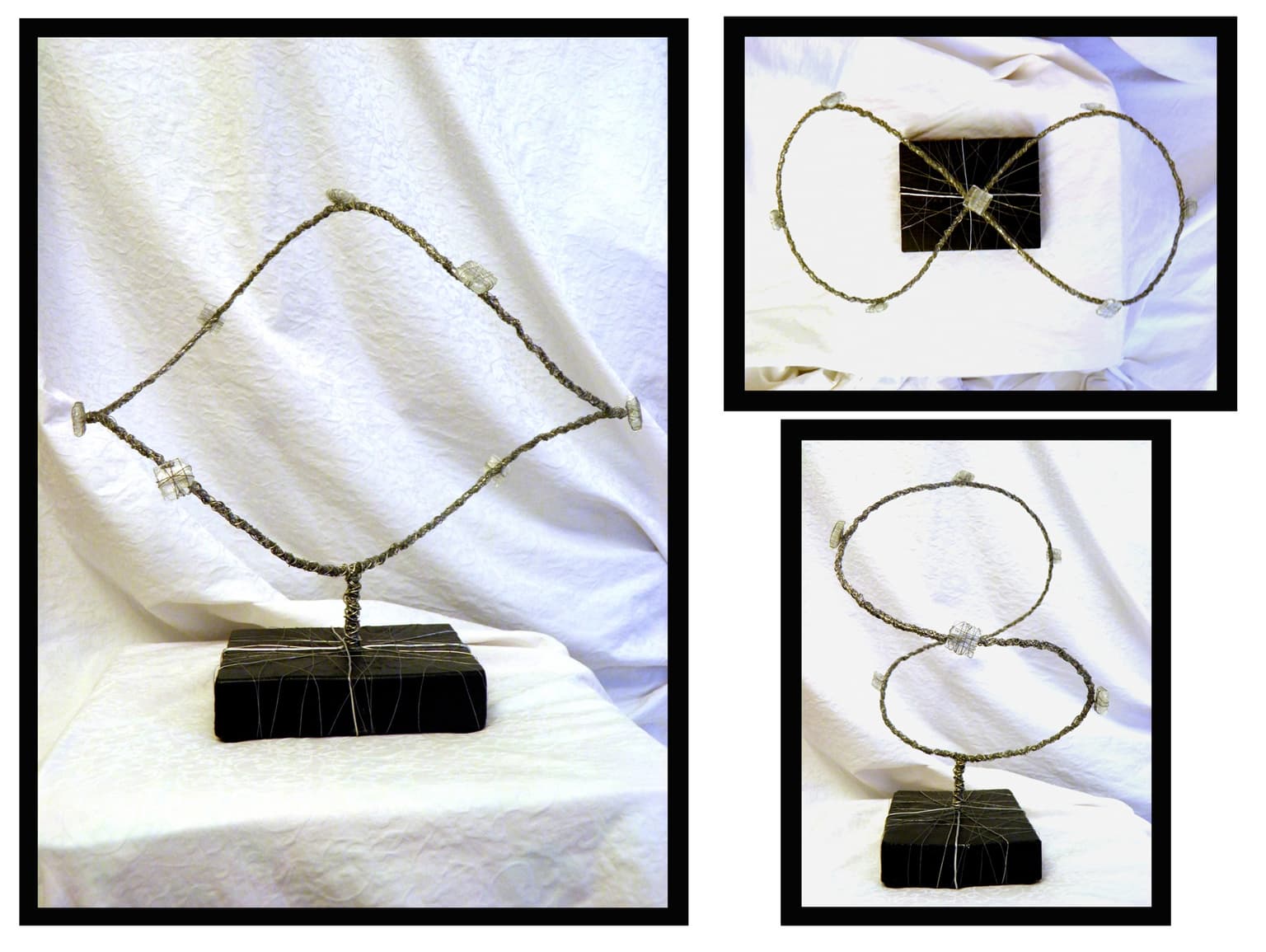
Legendrian Unknot
15"x15"x10"
Wire, glass beads, wood
2013
An unknot is any smooth deformation of a circle. The Legendrian unknot has the added geometric condition of being embedded in a contact structure. The standard contact structure in 3-space is a plane field that twists as it traverses the y-axis. A Legendrian knot is a knot whose tangent planes lie in this plane field. The glass beads along the wire sculpture represent the tangent planes at selected points. The twisting of the contact structure forms "cusps" on the knot. When viewed from the front (as shown) this Legendrian unknot appears lemon-shaped with two cusps. Notice that as the slope becomes more positive, the position of the strand is closer to the viewer. From the top or side view, the Legendrian unknot has a figure eight shape.