Abdalla G. M. Ahmed
Artists
Abdalla G. M. Ahmed
Independent
Khartoum, Sudan
Statement
Originally I am a telecommunications engineer. Accidentally discovering AA-patterns 15 years ago eventually lead me to know Bridges and its community. These patterns are so simple yet so rich, and they do not cease to inspire me. So far I found applications for them in pixel art (still and animated), weaving design, and most recently sampling (in computer graphics). My artworks this year are all inspired by AA patterns.
Artworks
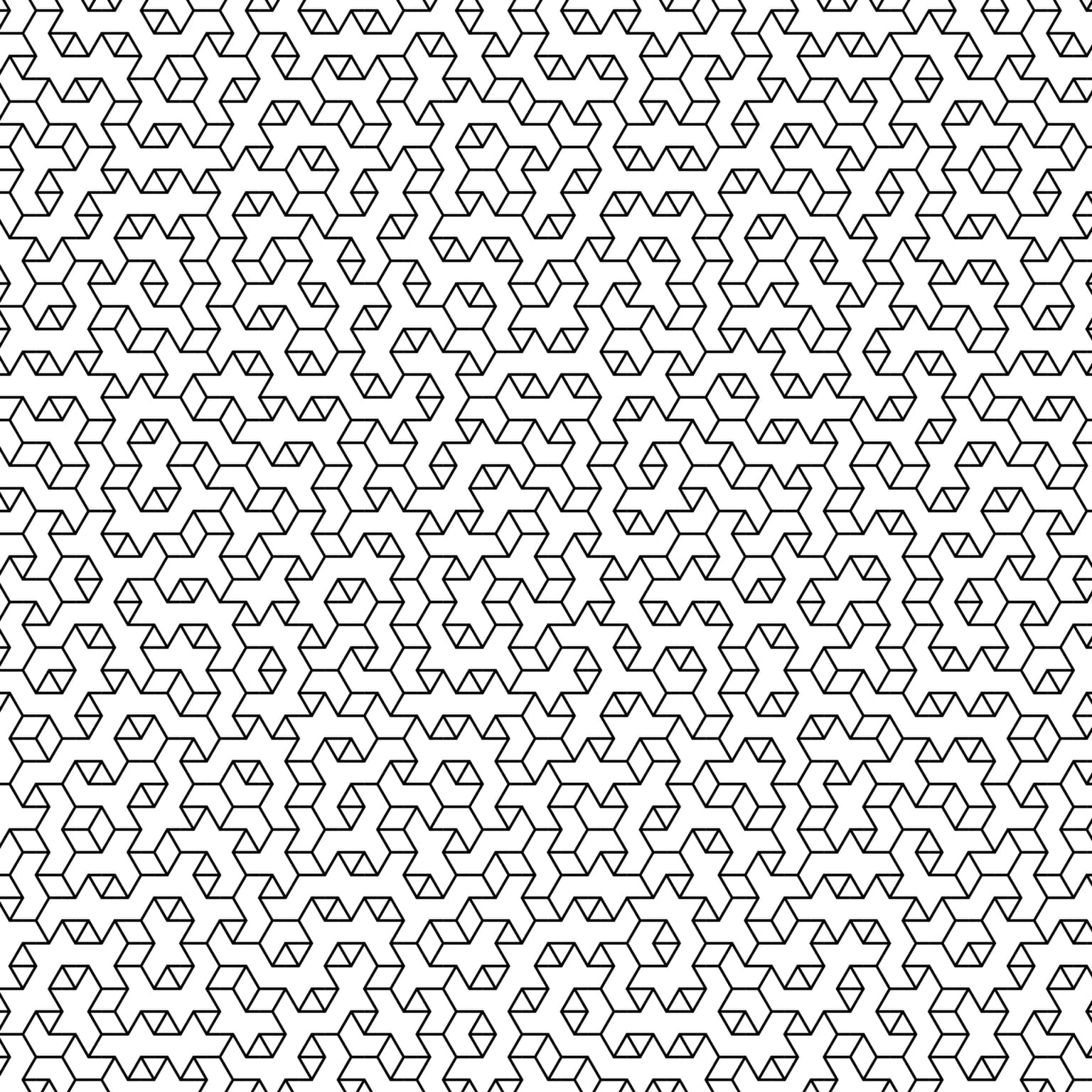
"Are these cubes projecting out or engraved in" is a common optical illusion.
Here we present a probably richer 3D illusion.
As I showed in a previous Bridges paper¹, interesting patterns are obtained by connecting every seconds pair of a regular grid of points (in rows and columns).
In this artwork we also connect every second pair of grid points, but we use a triangular gird. In each row we start randomly with a dash or gap. The resulting pattern looks complex, but a careful inspection reveals that every vertex is trivalent. Since edges meet at 120°, the angles of isometric projection, it gives the illusion of cubic corners, sometimes in, sometimes out, sometimes solid, and sometimes hollow.
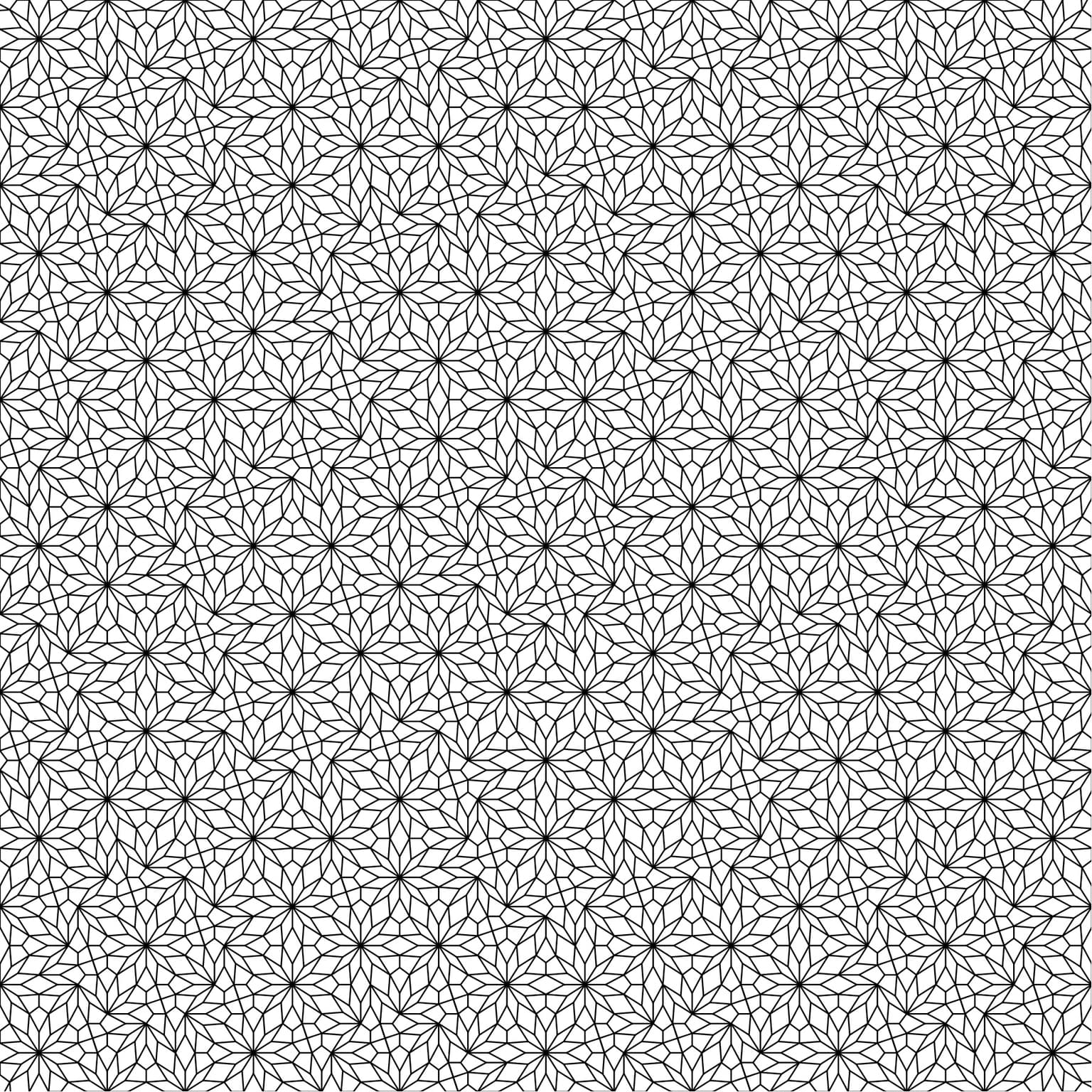
AA Flowers
42 x 30 x 1 cm
Digital print (A3)
2015
This pattern is produced from AA(sqrt(3)) by connecting each point to vertices of its Voronoi cell. This seemingly simple layout in fact embodies a lot of math from different areas; e.g:
- Geometry: tiles are quadrilaterals with perpendicular diagonals
- Tessellation: the few distinct tiles are an example set which can tile the plane periodically or aperiodically
- Topology: pattern points can be distinguished by valence ( > 3)
- Symmetry: (partial) translational symmetries (high auto-correlation) are evident at steps reflecting best rational approximations of the pattern parameter
12-fold rotational symmetries are also visible thanks to the parameter connection to angle π/6
Finally, it resembles Islamic arts, but is aperiodic!
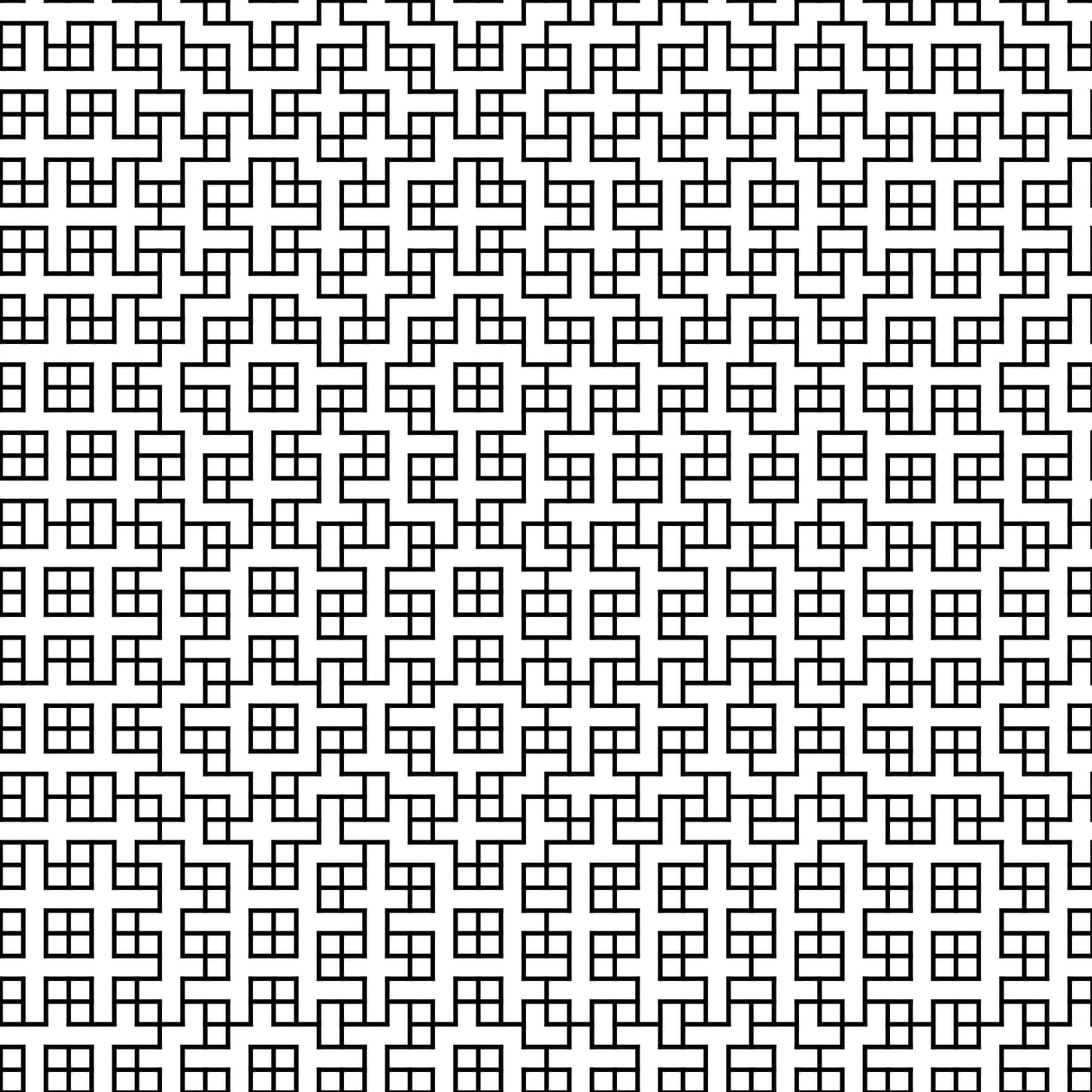
Boxes
42 x 30 x 1 cm
Digital print (A3)
2015
This is similar to previous one, but instead of changing the grid layout of AA outlines, we use a regular grid but make the dashes 2 steps long. The result is a puzzling layout of boxes. An artistic application which comes to mind is carpets and wallpapers.
Besides its aesthetic appeal this structure can be the subject for challenging problems in probability. For example, (easy) what is the probability of having a block of 4 boxes (which are always isolated), or (hard) what's the probability density function of contiguous areas.
A possibly challenging problem in topology is to prove (or disprove) that 4-boxes are the only possible disjoint blocks.