Brendan Kinnell
Artists
Brendan Kinnell
Math Teacher
Packer Collegiate Institute
Brooklyn, New York, USA
Statement
Most of my work is result of play: What would happen if we started with some visual structure and allowed it to be altered in some systematic way? I tend to explore how patterns and form are generated through transformation and randomization. The content of the work generally begins with simple principles -- tessellations, duals, geometric puzzles (such as Sangaku), or the graphs of functions. Through a mix of programming and a bit of luck, I sometimes find new and interesting visual structures.
Artworks
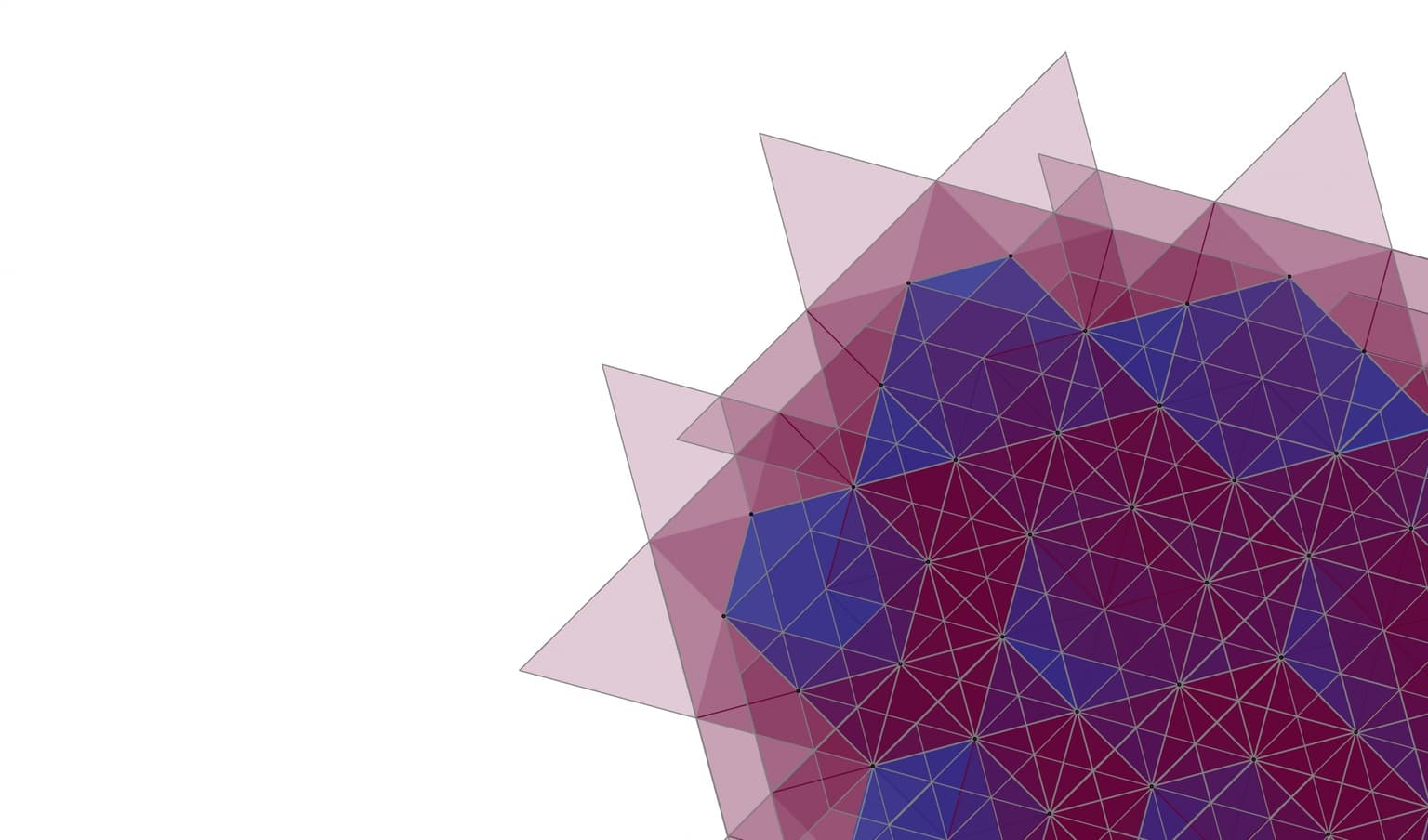
Extended Dual
44 x 26 cm
Giclée print on paper
2014
What could the transition between geometric duals look like? By programming growing quadrilaterals from the vertices of the polygons (in this case, hexagons and triangles), the dual of this tessellation can be achieved. By allowing the growth to continue beyond the dual, the figures extend beyond the boundaries of the original tessellation, bleeding into adjacent regions, blending colors. This a is a detail of the extended dual at its outer boundary.
Unfolding Triangles
90 x 90 cm
Giclée print on paper
2014
The question explored in this piece is, "What does it look like when a triangle is divided and unhinged?" By cutting along the medians and unfolding using segment midpoints as pivots, a trapezoid can be formed. To me, as a teacher of geometry, this makes for an interesting animation. This piece documents the traces of this transition, being composed of the intermediate figures as this unfolding occurs. The tight patterns (and deeper colors) near the top relate to the smaller pieces unhinging, while the bottom portion's lighter feel is the result of the accumulated larger pieces as they approach the trapezoidal shape.

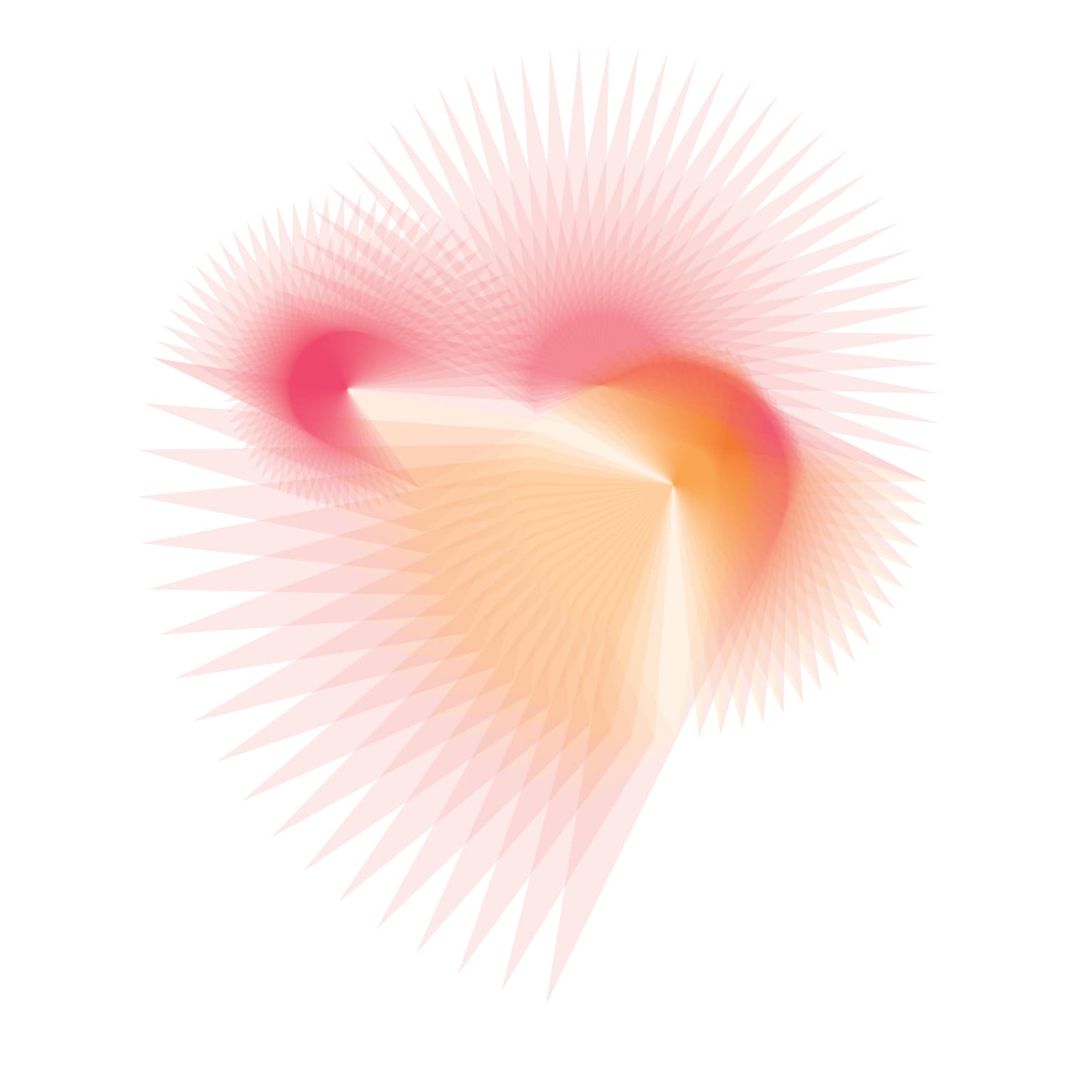
Splat
100 x 70 cm
Giclée print on paper
2014
Randomly chosen angles and lengths are used to construct segments radiating from an origin. The midpoints of these segments form the vertices of these polygons, and the color is a function of the polygon's area. I like to call these "starburst polygons."