2015 Joint Mathematics Meetings
Anne Burns
Artists
Anne Burns
Professor Emerita (Mathematics)
Long Island University
Huntington, New York, USA
Statement
I began as an art major and then switched to mathematics. When I bought my first computer in the 1980's I immediately started making stick figures of plants using Basic. As computers became better, so did my programming skills and my interest in visualizing mathematical concepts. In addition to botanical simulations I am interested in complex dynamical systems and I eagerly await Robert Devaney's latest explorations.
Artworks
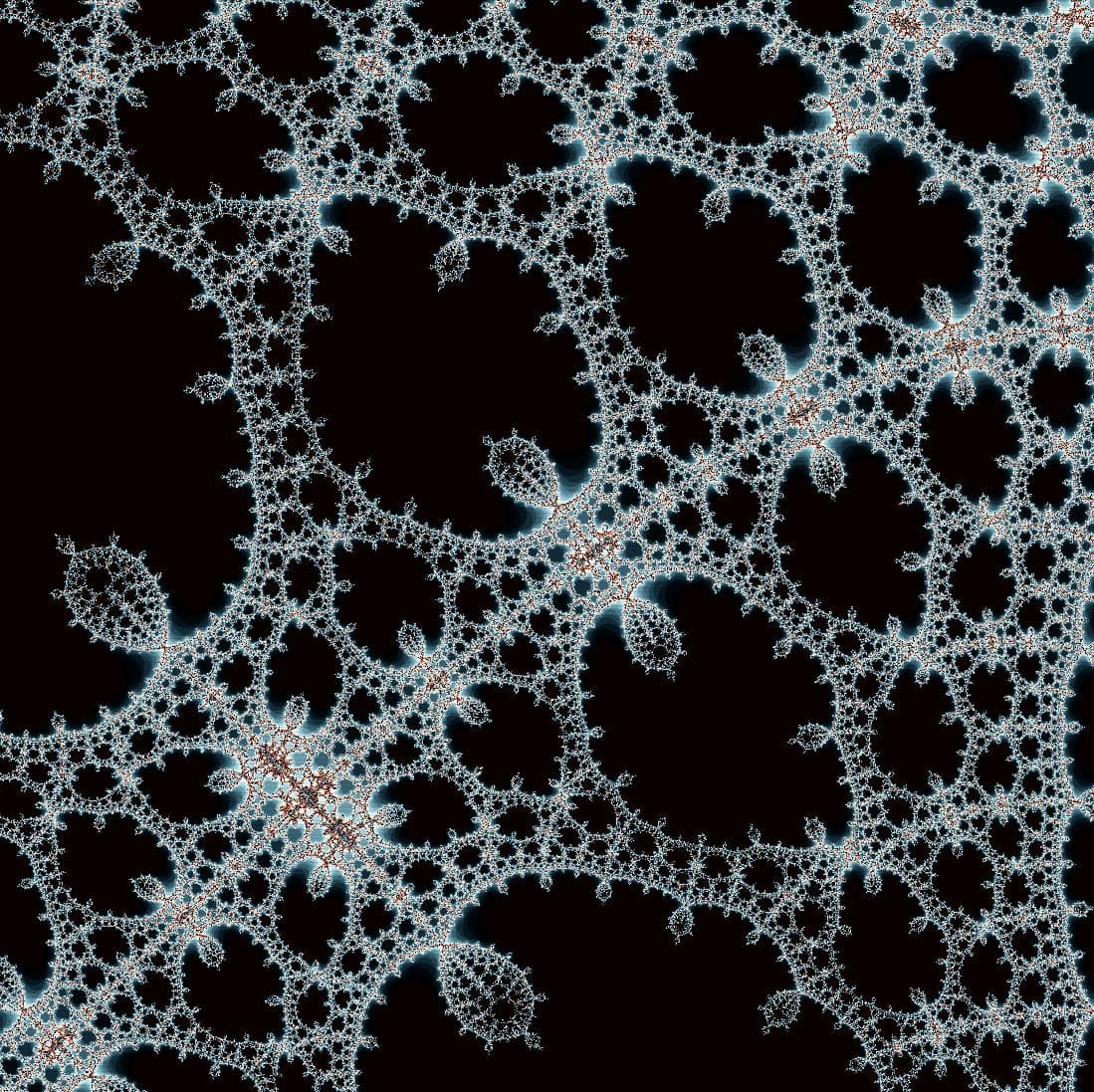
Basilica Zoom
13" X 13"
Digital print
2014
"Basilica Zoom" is a zooming in on a portion of the image "Basilica"; here the color values are reversed, with the set of points whose orbits escape colored black.
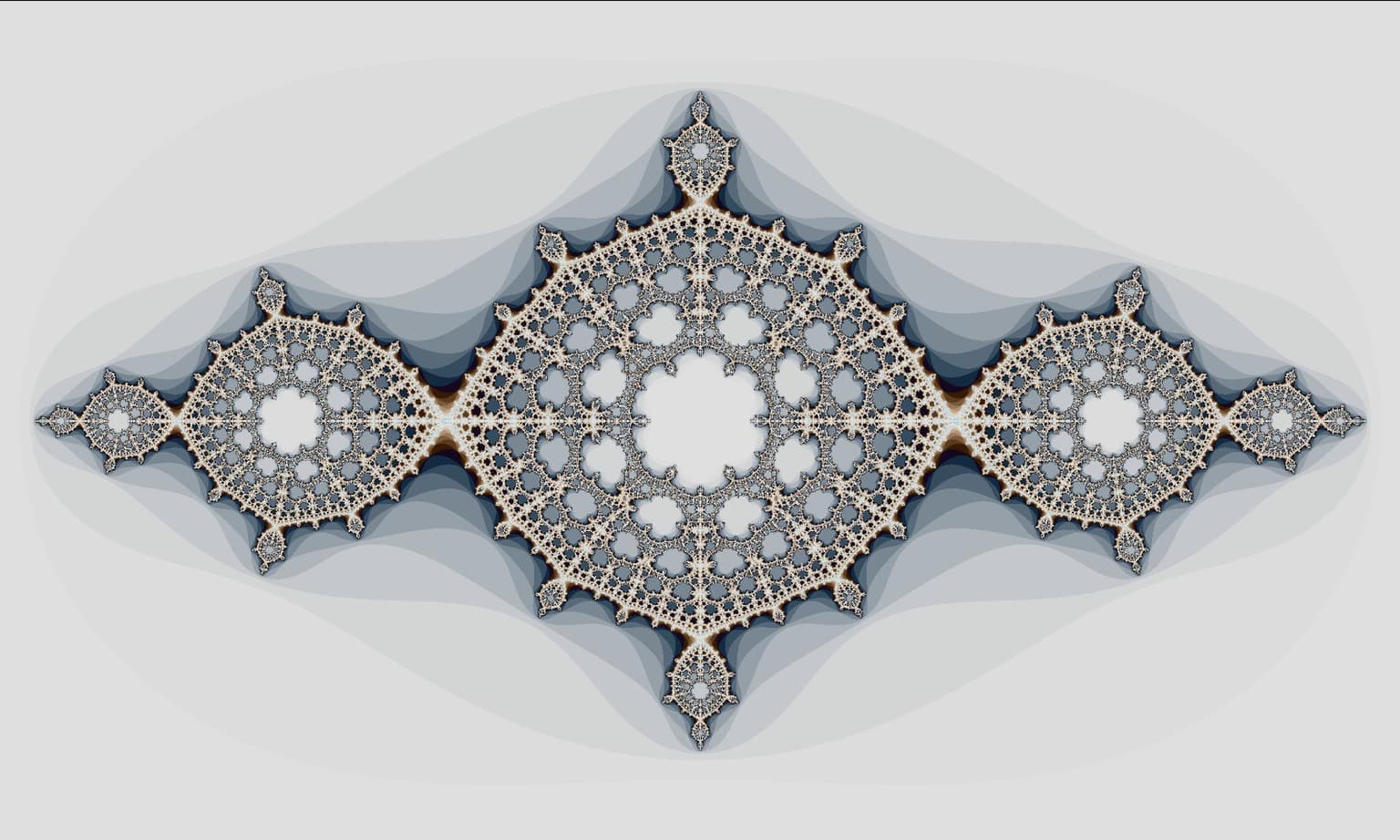
Basilica
12" X 18"
Digital print
2014
The Julia Sets of z^n + c are familiar objects to mathematicians. In particular c=-1 yields the well-known "Basilica". Adding a term d/z(z^2-1) introduces three poles: z=0, z=-1, z=1. The orbits of initial points near the poles rapidly diverge to ∞; for very "small" (real, positive) d, amazingly, the boundary of the set of points whose orbit escapes (the Julia Set) contains an infinite number of tiny decorations resembling the decorations on the original "Basilica".