2015 Joint Mathematics Meetings
David Bachman, Robert Fathauer, and Henry Segerman
Artists
David Bachman
Professor of Mathematics; Associate Professor of Mathematics
Pitzer College
Claremont, CA
Statement
[DB] My design process starts with translating a physical form to a mathematical model, which can be endlessly modified. Truly new objects are then produced from these models by modern digital fabrication techniques. [RF] I'm endlessly fascinated by certain aspects of our world, including symmetry, chaos, and infinity. Mathematics allows me to explore these topics in distinctive artworks that I feel are an intriguing blend of complexity and beauty. [HS] My mathematical research is in 3-dimensional geometry and topology, and concepts from those areas often appear in my work. Other artistic interests involve procedural generation, self reference, ambigrams and puzzles.
Artworks
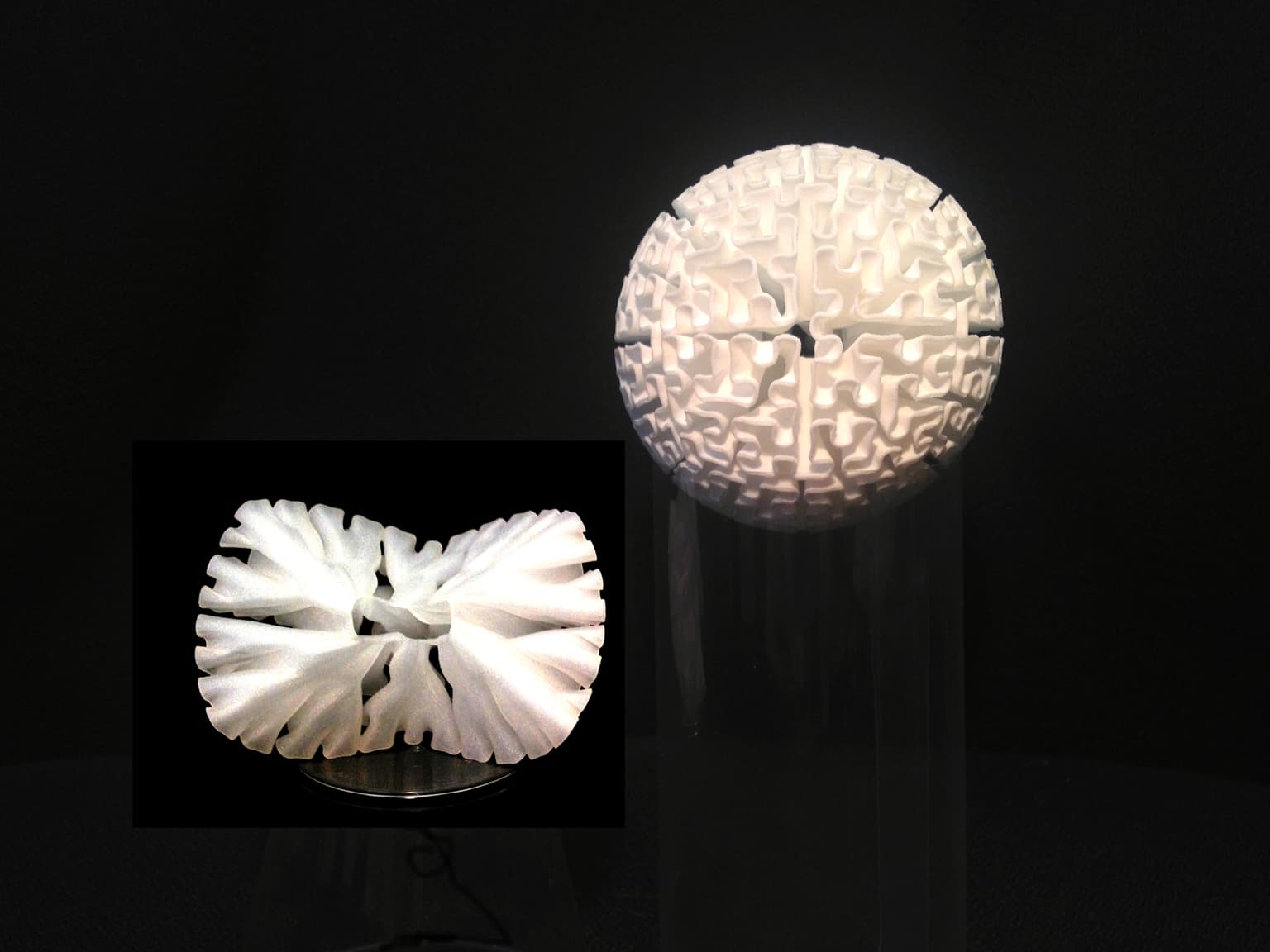
Hilbert Sphere
3.15" diameter for the full sphere
3D printed plastic, resin
2014