Renate Quehenberger
Artists
Renate Quehenberger
Art researcher
Independent
Vienna
Statement
I am a captive of Plato's Ideal World -- focusing on the visualization of higher-dimensional spaces and their physical significances I am exploring different geometries and their application through the history of art and science. Currently I am editing the book on the visualization of quantum phenomena by means of digital arts. Besides pursuing my thesis in philosophy 'On the Hermeneutics of the Penrose Pattern' I'm promoting my contribution to mathematics: the 3D representation of the Penrose Kites and Darts tiling through various publications, screenings and exhibitions in the art and science contexts.
Artworks
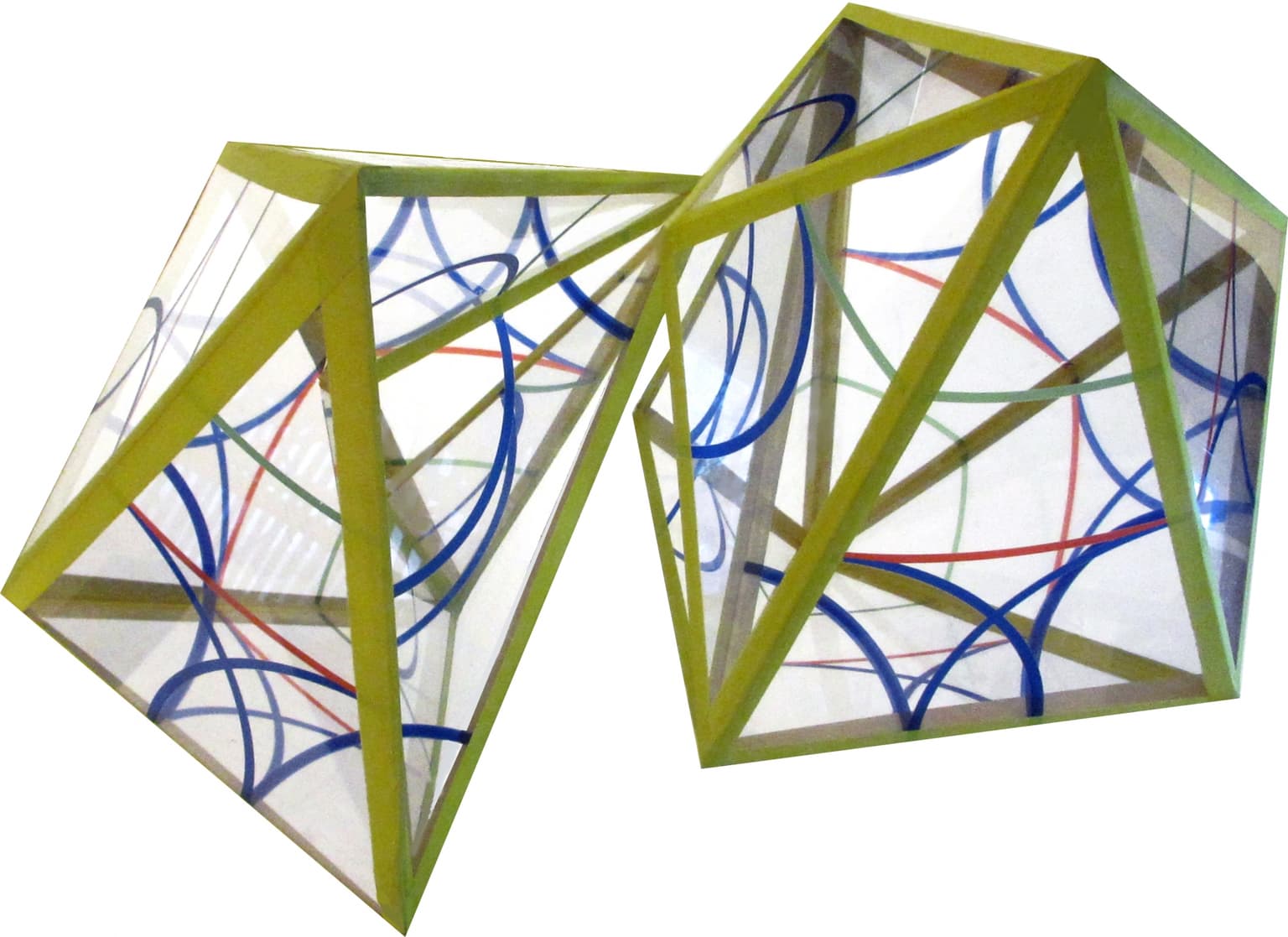
Epitahedron (E±)
35 x 50 x 45 cm
Acrylic sheets, printed
2015
Epitahedra are the 3D representation of the Penrose Kites and Darts tiling.
The volume of the convex epitahedron E+ (E "plus") demeans 1/6 (0,666667) and the concave E- (E "minus") φ /6 (0,1030057).
Thus the volumes of E+ and E- are in the Golden Ratio as well as the lengths of the edges. the volumes of the concave epitahedron (E-) and the convex (E+) and both together (EE ) are conform to the golden ratio:
E- : E+ = E+ : EE = φ (≅ 1.618).
Dividing EE into E+ and E- therefore means dividing space in the golden ratio
analogous to the tessellation of the plane by means of Penrose patterns.