2016 Bridges Conference

Taneli Luotoniemi

Artists

Taneli Luotoniemi

Doctoral candidate of art

Dept. of Mathematics and Systems Analysis, Aalto University

Helsinki

taneli.luotoniemi@aalto.fi

http://therearemorethings.org

Statement

The geometric concept of the fourth spatial dimension has had a lasting effect on Western culture and science. My doctoral work in the context of art education – particularly in the interdisciplinary context of mathematics and art, studies the possibilities of making 3-dimensional objects that represent hyperspatial content and have visual quality of an art piece. Constructive experiments in graphic, plastic and virtual media are used, informed by theoretical background of low-dimensional topology and projective geometry. Even if a full understanding of the artifacts requires some mathematical knowledge of 4D space, I hope that the pieces will also evoke immediate visual attraction, even for the lay audience.

Artworks

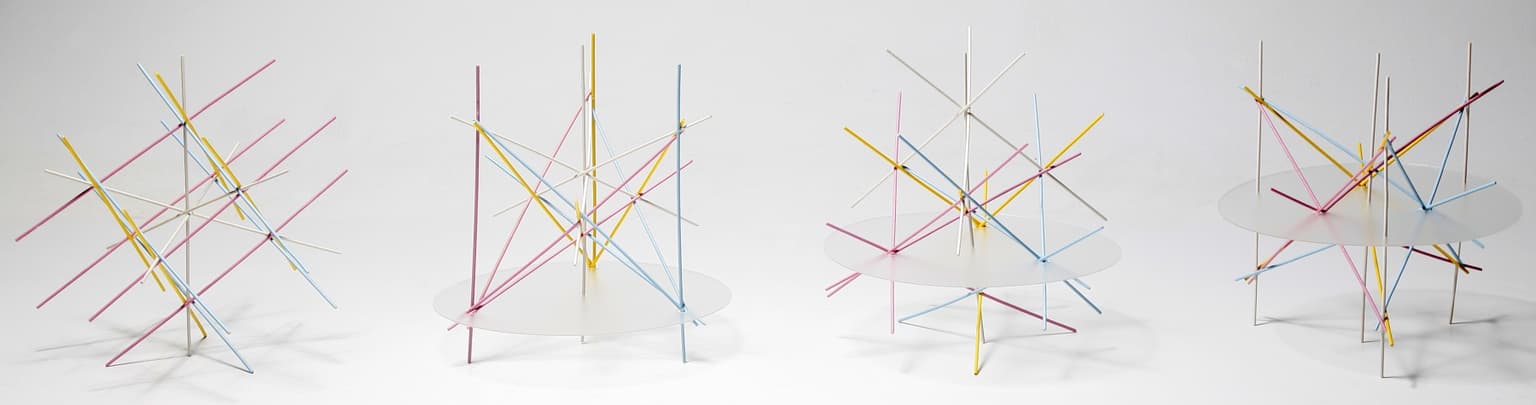
Image for entry 'Ideal Plane of a Cube'

Ideal Plane of a Cube

30 x 30 x 30 cm

Painted wooden rods, acrylic sheets

2016

According to classic geometry, parallel lines do not meet. In projective geometry however, parallel lines are thought of as meeting at an 'ideal point'. In perspective drawing these points are called vanishing points, and in 3-dimensional space they lie on an 'ideal plane'. The sequence of four models depict a cube in a projective setting, and show how the ideal plane, and the vanishing points on it, can be brought into view. In the final stage, the three ideal points are positioned around the center of the model, and the center of the original cube is at infinity. This transition corresponds to the rotation of a 4-dimensional polytope called the 24-cell, and the subsequent change in appearance of its projections in Euclidean 3-space.