Zachary Abel
Artists
Zachary Abel
Graduate Student
Applied Math Department, Massachusetts Institute of Technology
Massachusetts, USA
Statement
I enjoy discovering mathematical wonder and beauty in familiar, overlooked places. This endeavor often manifests as geometric sculptural forms made from office supplies and their kin. Veering slightly from this tradition, in these works I use the prime numbers themselves as an unlikely visual medium, finding prime numbers that, at first, seem like they couldn't possibly exist.
Artworks
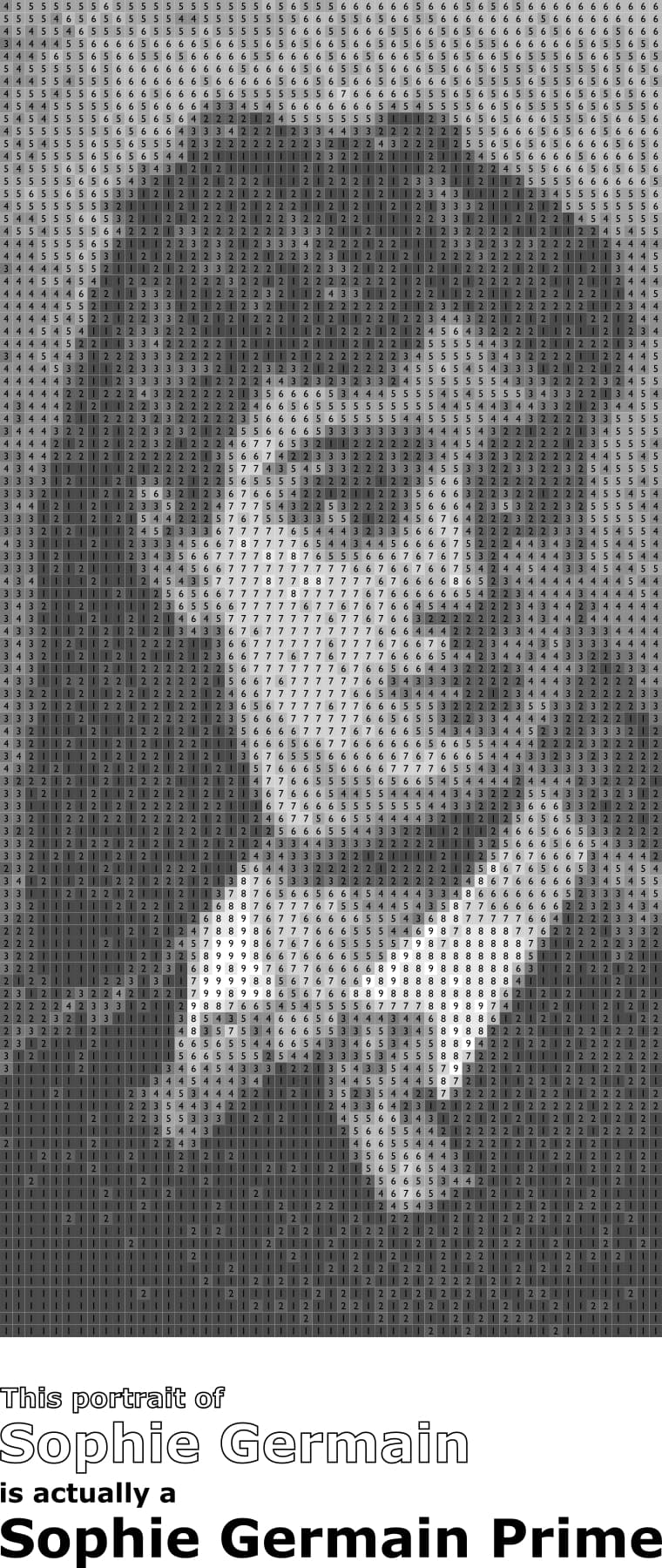
Sophie Germain Sophie Germain Prime
32 x 14 cm
Digital Print
2016
In this piece, a prime number P was carefully chosen so that its 53x107=5671 digits, when arranged in a grid (in order) and shaded by value, reveal a portrait of Sophie Germain. In fact, this prime P was even more carefully chosen so that 2P+1 is also prime, thus making P a Sophie Germain prime in the mathematical sense (like 53, since 2x53+1=107 is also prime). The existence of a Sophie Germain prime bearing Sophie Germain's appearance highlights the conjectured high density of Sophie Germain primes: even though they are rarer than regular primes, they are believed to possess a similar ubiquity. This is unproved---we still don't even know if there are infinitely many!

Gaussian Gaussian Prime
42 x 37 cm
Digital Print
2016
In "Gaussian Gaussian Prime", two integers A and B are drawn in separate 145x72 grids (A is drawn in red on the left, and B is drawn in blue on the right), where A and B are chosen so that the Gaussian integer A+Bi cannot be (nontrivially) factored into smaller Gaussian integers, i.e., A+Bi is mathematically a Gaussian Prime. These are known to possess density properties in the complex plane that are similar to those of integer primes on the real line.