2016 Joint Mathematics Meetings
S. Louise Gould/ Franklin Gould
Artists
S. Louise Gould
Professor Emerita
Department of Mathematical Sciences, Central Connecticut State University
Connecticut USA
Statement
My mathematical art grows out of my experiences with my students and my explorations of mathematics, textiles, paper, and technology. I enjoy working with computer controlled machines such as the computerized embroidery sewing machine and the Craft Robo (plotter cutter) as well as traditional looms and knitting machines.
Artworks
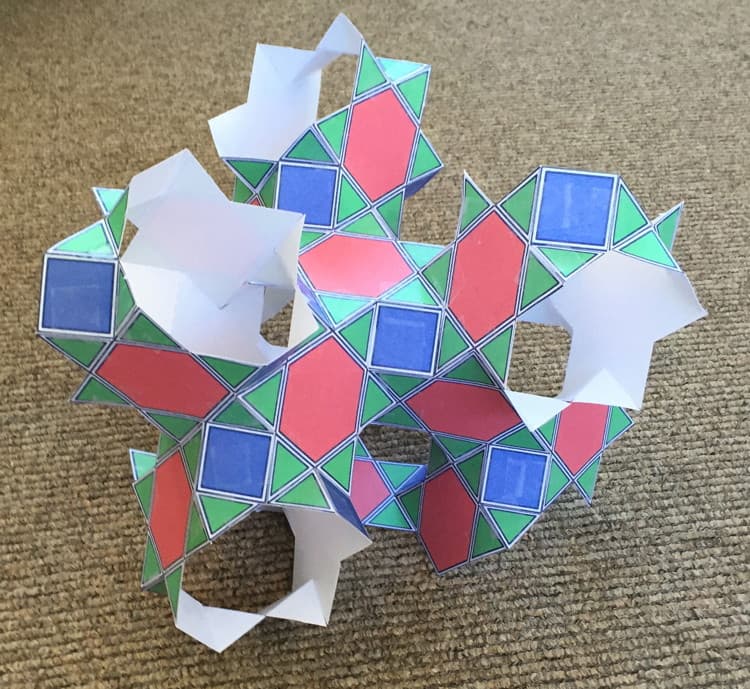
Multiplied Snub Cube II
23 x 28 x 28 cm
Printed card stock
2015
This multiplied snub cube illustrates the geometry of the infinite polyhedron 6.3.4.3.3. Conway et al. in “The Symmetry of Things” point out how this surface can be derived from the “mu cube” or from the “mu octahedron” surfaces using the same process by which the snub cube is derived from the cube or from the octahedron. When we “snub” the mu octahedron, we see that as the four hexagons rotate and separate, their planes no longer meet at one vertex but meet, instead, in the 6 edges of a tetrahedron. That gap is then bridged by a square bounded by four triangles. Each of the four triangles shares an edge with one of the hexaons and is also co-planar with it. The resulting surface looks as if it is tiled by six-pointed stars and squares.

Multiplied Snub Cube I
19 x 26 x 26 cm
Wool Felt and Embroidery Thread
2015
This multiplied snub cube illustrates the organic or topological features of this Archimedean-like infinite polyhedron. It tiles the hyperbolic plane with regular hexagons, squares and triangles that meet at each vertex in the order 6.3.4.3.3 going clockwise (or alternatively, counterclockwise). Its triply-periodic surface separates Euclidean three-space into two connected congruent parts where the “negative space” is a mirror image of the “positive space.” In this infinite polyhedron the triangles lie in the same planes as the hexagons making them appear to be six-pointed stars connected to each other directly and by squares.