Vivian Wang
Artists
Statement
As both a math-lover and artist, I am curious about geometry, color, and symmetry. I enjoy learning and playing around with different art forms and their applications to the realm of mathematical artwork, particularly those that reside in three dimensions. Currently, I am interested in the transformation of traditional 2D digital prints on paper and canvas into 3D forms.
Artworks

Faces of Hilbert and Sierpinski
21 x 35 x 21 cm
canvas
2017
This piece consists of two prints on canvas that are replicated, cut, and assembled together. The designs are made by generating a space-filling curve and coloring the pixel values based on the values of the underlying images (of the mathematicians that created these two curves). I tweaked the curves slightly to add embellishment to the straight line curves for aesthetic reasons. The structure is two-sided: on the left, Hilbert space-filling curves reveal Hilbert's face; on the right, Sierpinski space-filling curves reveal Sierpinski's face. The prints are cut out linearly to create a sliceform of a hyperbolic paraboloid. However, due to rolling of the prints at the edges, the surface exhibits an interesting curvature.
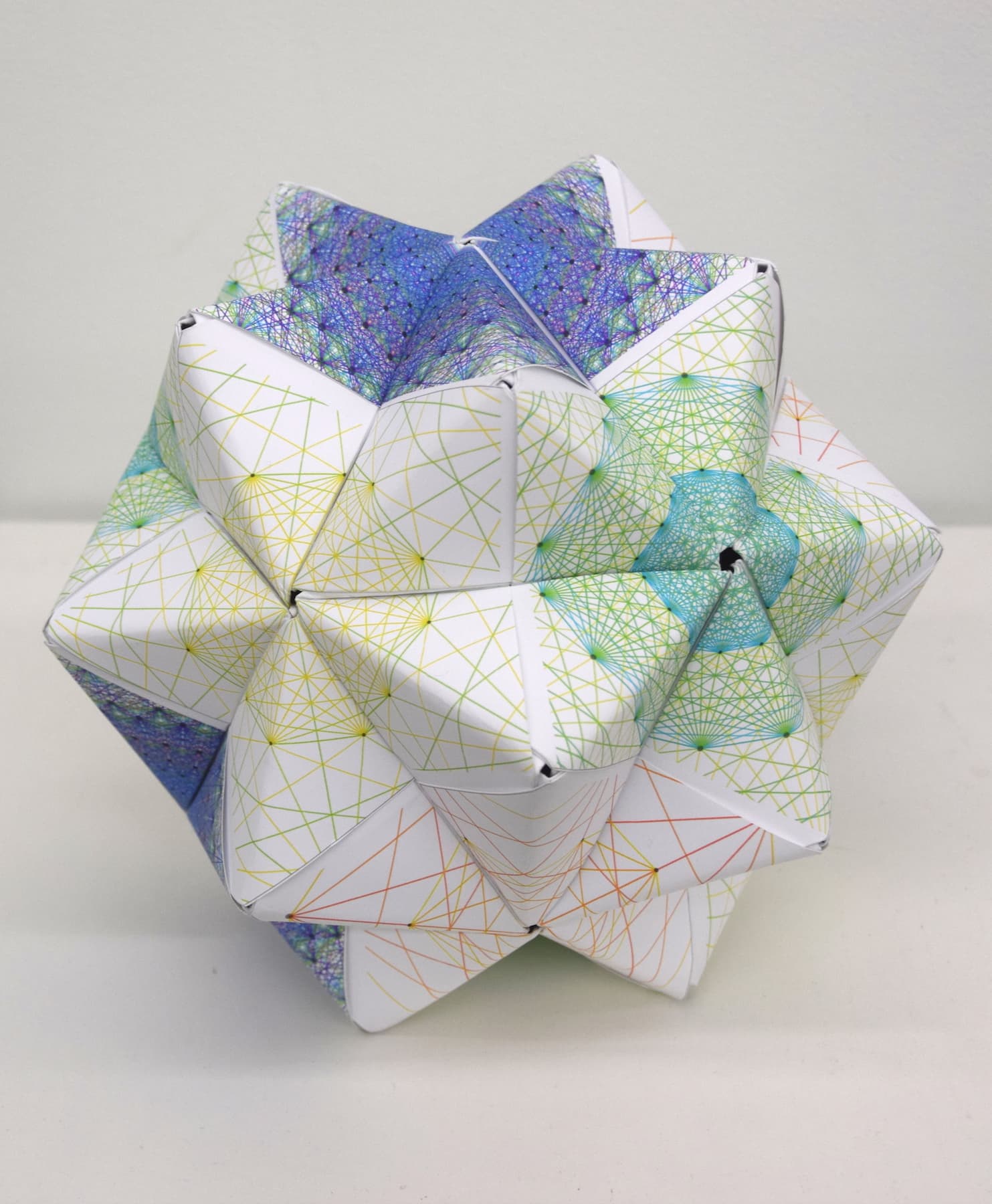
Polytopic Polyhedron
30 x 30 x 30 cm
paper
2017
This triakis icosahedral polyhedron consists of 30 Sonobe units. There are four different patterns (in varying color schemes) patterned across the larger "faces" of the polyhedron, each of which represents Coxeter plane projections of different polytopes of increasing complexity (up to E8). The colored patterns are aligned in a rotationally symmetric manner across the polyhedron. The challenge in constructing such a piece resided in (1) transforming the 2D geometric designs to lie on folded 3D surfaces and (2) designing the individual origami squares to contain the images in the correct positions, so as to create the desired patterns when folded.