Vincent J. Matsko

Statement

Computer-generated art involves ideas not conceivable before the advent of modern technology. My recent work involves randomness on a large scale, experimentation with color, and integrating thousands, sometimes millions, of individual elements in a single composition. Work in this exhibition explores creating images consisting of line segments by varying the angles in the well-known recursive algorithm for producing the Koch snowflake. Because the geometry of the resulting images is so intricate, a very limited color palette is used, thereby allowing the geometry of the images to generate their aesthetic appeal.

Artworks

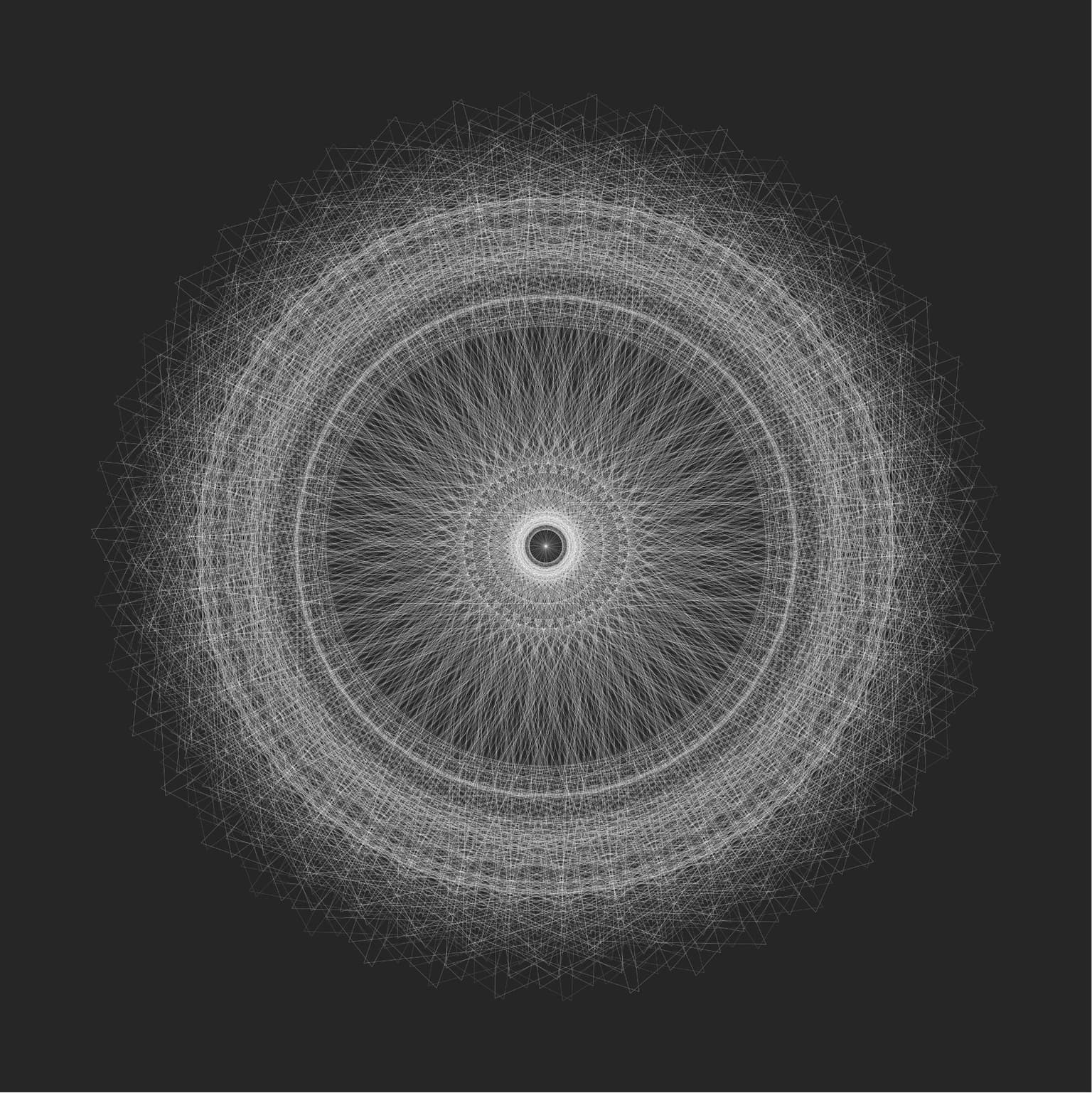
The algorithm used to create this image is the same as that used to recursively define the Koch curve. In this case, the circle is divided into 336 parts (rather than 360 degrees), and the angles used in the algorithm are 19 and 107 of these parts (rather than 60 and 240 degrees). In this case, the curve is bounded, consists of 10,752 segments, and possesses 42-fold symmetry.

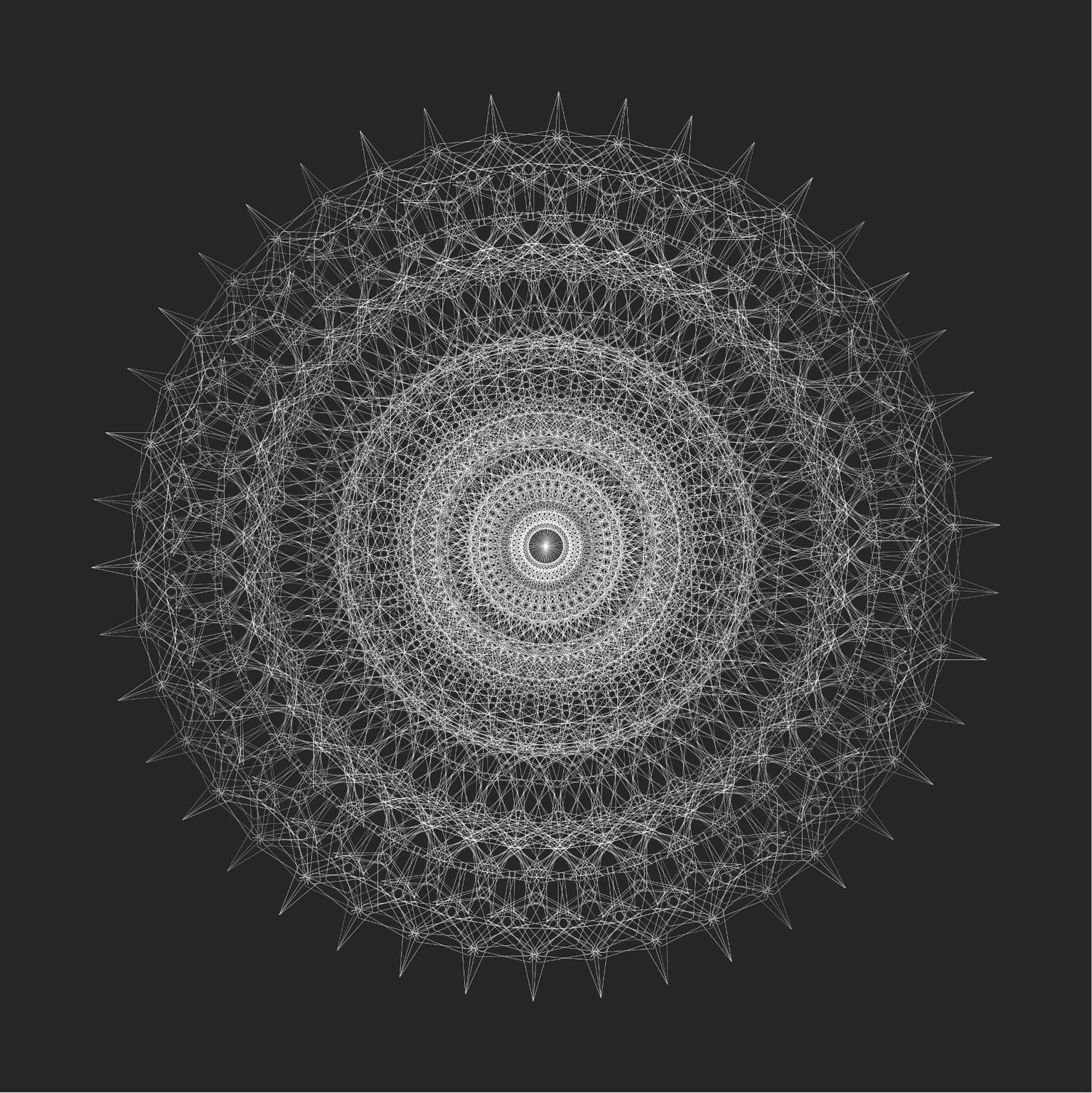
The algorithm used to create this image is the same as that used to recursively define the Koch curve. In this case, the circle is divided into 336 parts (rather than 360 degrees), and the angles used in the algorithm are 283 and 179 of these parts (rather than 60 and 240 degrees). In this case, the curve is bounded, consists of 10,752 segments, and possesses 42-fold symmetry.