2018 Joint Mathematics Meetings
Andrew Smith
Artists
Andrew James Smith
Artist
Cambridge, Ontario, Canada
Statement
There are two distinct, discrete spirals. Equal-length, straight lines compose both. One has equal distance between its windings. Plato’s math teacher, Theodorus of Cyrene, created that one 2400 years ago. In 2005, I created the other, the Protogon spiral, whose windings grow farther apart. The “Time Hook” is a metaphysical concoction contrasting the two spirals. My supposition is, in a quantum world where only straight lines exist, there are only two spirals. I imagine they ornament either side of the beak of a creature which rules time. It feasts by piercing the veil of space in a quantum manner, parting pixels where no curves are available. Space flow reflects one spiral while the wayward side exudes an eddy of the opposing spiral.
Artworks
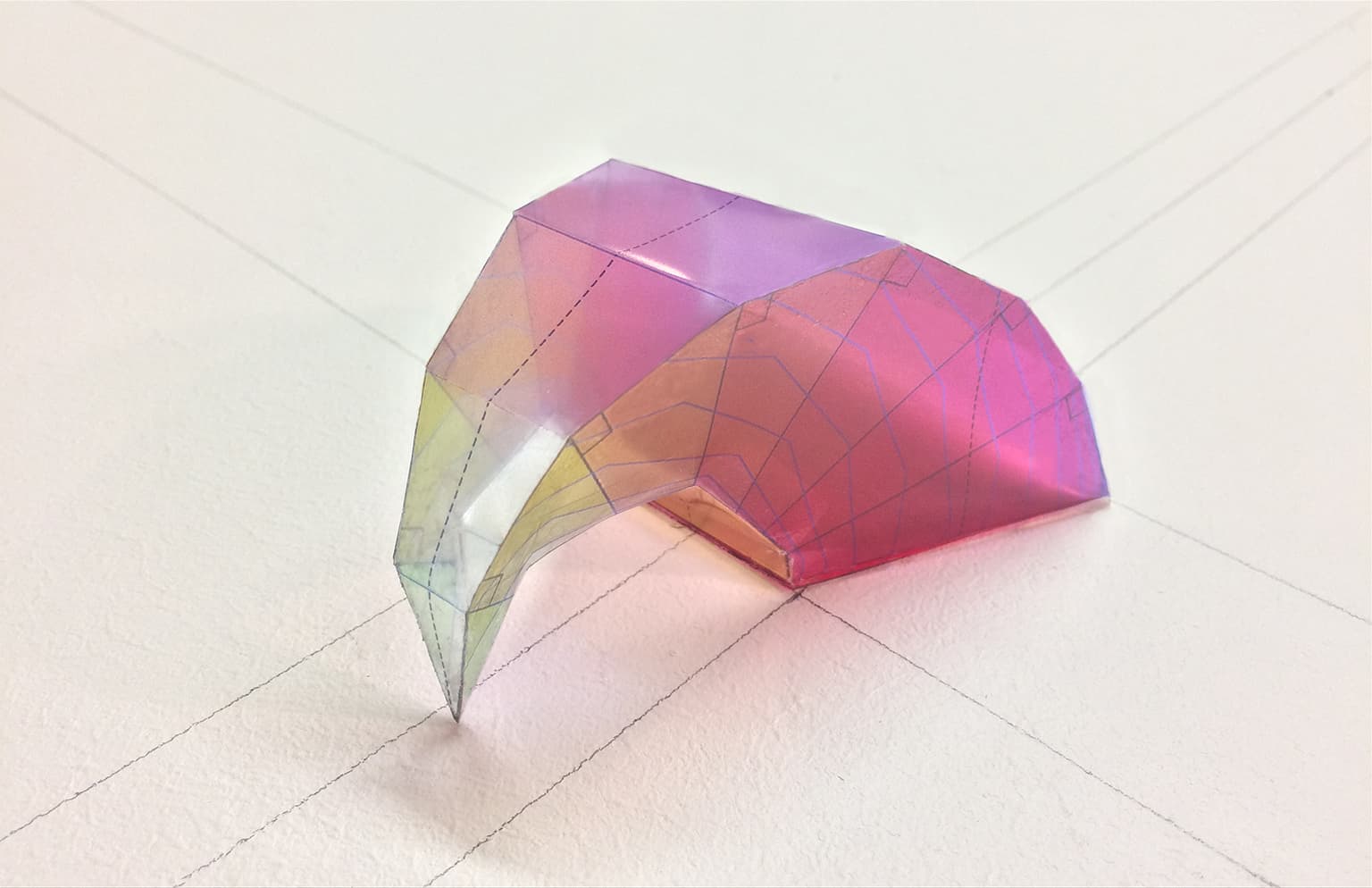
Beak 2
28 x 48 cm
Pigment print on handmade paper.
2017