2018 Joint Mathematics Meetings
Ethan Bolker
Artists
Ethan Bolker
Professor of Mathematics, Emeritus
UMass Boston
Boston, MA
Statement
I've built mathematical models ever since I read Hugo Steinhaus's "Mathematical Snapshots" more than 60 years ago. Over time I graduated from crude cardboard platonic polyhedra and soma cubes to some constructions friends have graciously called art.
Artworks
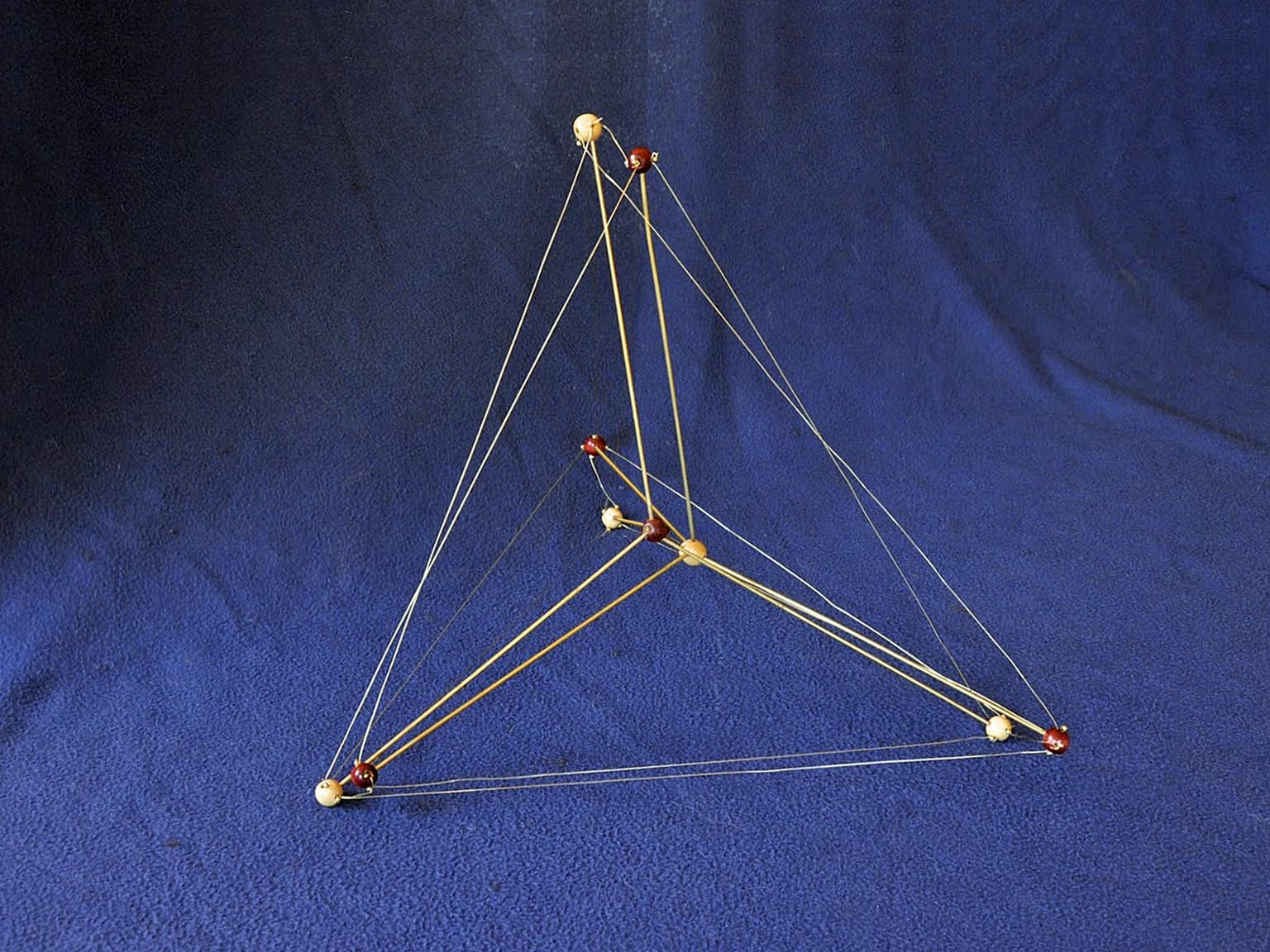
K(5,5)
44 x 44 x 44 cm
Wood and brass
2017
Counting suggests that the complete bipartite graph K(5,5) might be rigid even though it has no triangles. Ben Roth and I proved that in "When is a bipartite graph a rigid framework?" (Pacific Journal of Mathematics, 90, 1981). The graph has one more edge than the number of degrees of freedom, so can be built as a tensegrity structure. In this realization four of the five vertices from each set form a tetrahedron with the fifth vertex near its center. The tetrahedra overlap so that the center vertex of each is inside the other. Eight struts join each center vertex to the four vertices of the other tetrahedron. The remaining 17 edges are cables.