2018 Joint Mathematics Meetings
Rona Gurkewitz and Bennett Arnstein
Artists
Rona Gurkewitz, Bennett Arnstein
Professor Emerita of Computer Science, retired mechanical engineer
Western Connecticut State University
Danbury, Connecticut, USA
Statement
Bennett and I experimented with Gyroscoping different regular polyhedra. We were motivated to find new forms and new origami models in a system or family of related polyhedra. The family was built on a common algorithm and functionality of modules with different regular polygon starting shapes. Gyroscoping a regular polyhedron involves putting a module with a point on each face and having the bases of the modules connect without glue. The gyroscoped model is an example of an unusual model in modular origami. It is made up of three different modules. Our third book has instructions for making these models and others. "Multimodular Origami Polyhedra: Archimedeans, Buckyballs and Duality", Dover Publications, 2003.
Artworks
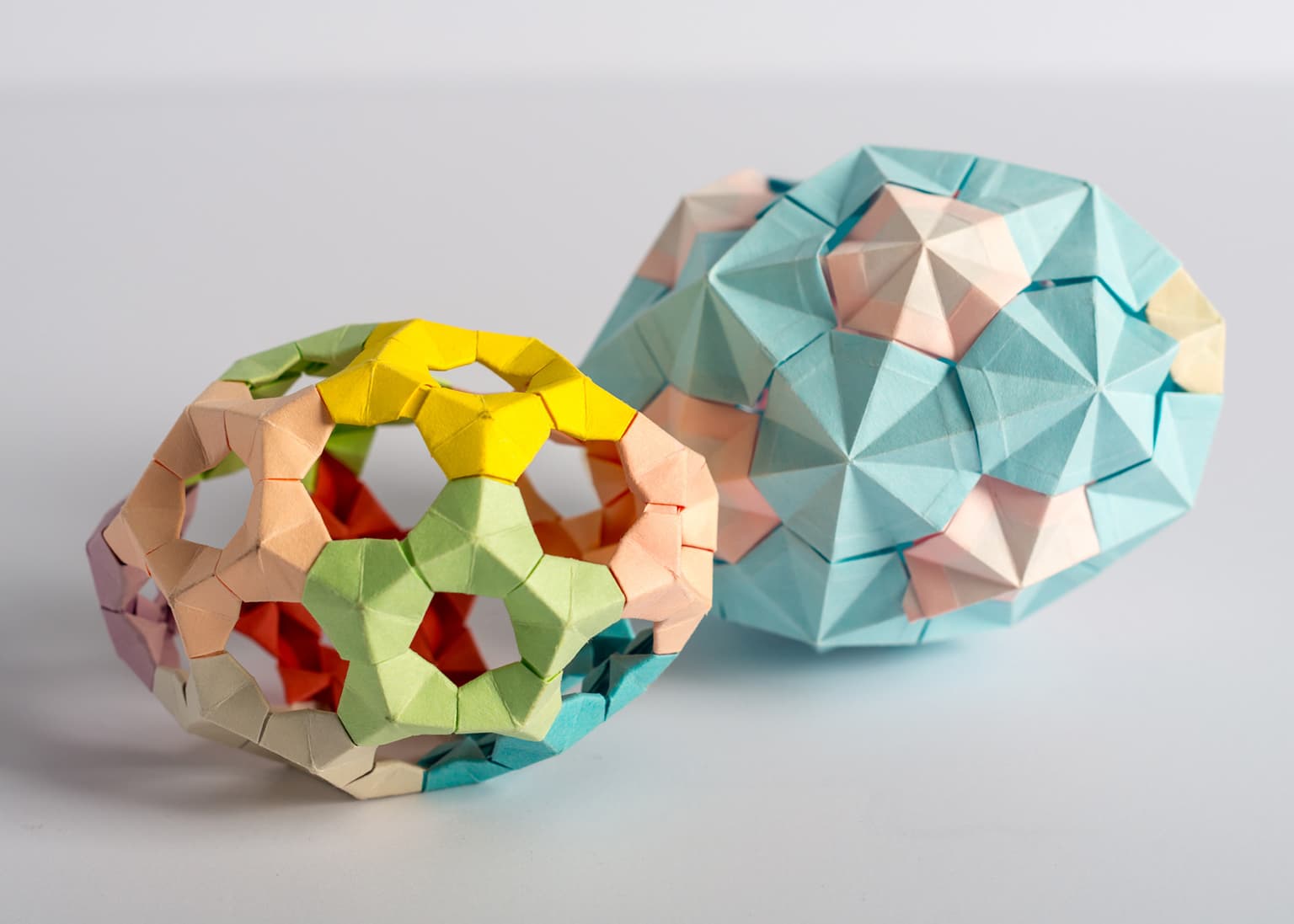
Egg and Gyroscoped Egg
6 x 15 x 6 cm
paper
1992 and 2000