2019 Joint Mathematics Meetings
David Plaxco
Artists
David Plaxco
Assistant Professor
Clayton State University
Morrow, Georgia, USA
Statement
The "Cubes Underscore Art" project (@cubes_art) is an investigation into the types of aesthetic patterns that can be rendered on nxnxn Rubik's cubes (and other twisty puzzles). This work has intrinsic connections to group theory and provides a rich example of how multiple group elements and their inverses can be composed and leveraged in real situations to generate patterns and designs.
Artworks

Single Thread, 2-Pattern Solve on 8x8x8
7 x 7 x 7 cm
Stickerless MoFang JiaoShi MF8 8x8x8 Puzzle Cube
2018
This design incorporates four of the algorithms that I have developed since beginning the Cubes Underscore Art project in the Fall of 2017. Tracing the path of the stripe all the way around the cube will lead one to traverse each face twice in a journey that alternates weaving over and under until arriving at the beginning again. There are two general patterns, each demonstrating 120 degree symmetry around a corner of the cube. I enjoy this piece because it seems at first like a straightforward pattern, but necessitates some of the most complex algorithms that I have developed using conjugates and commutators.
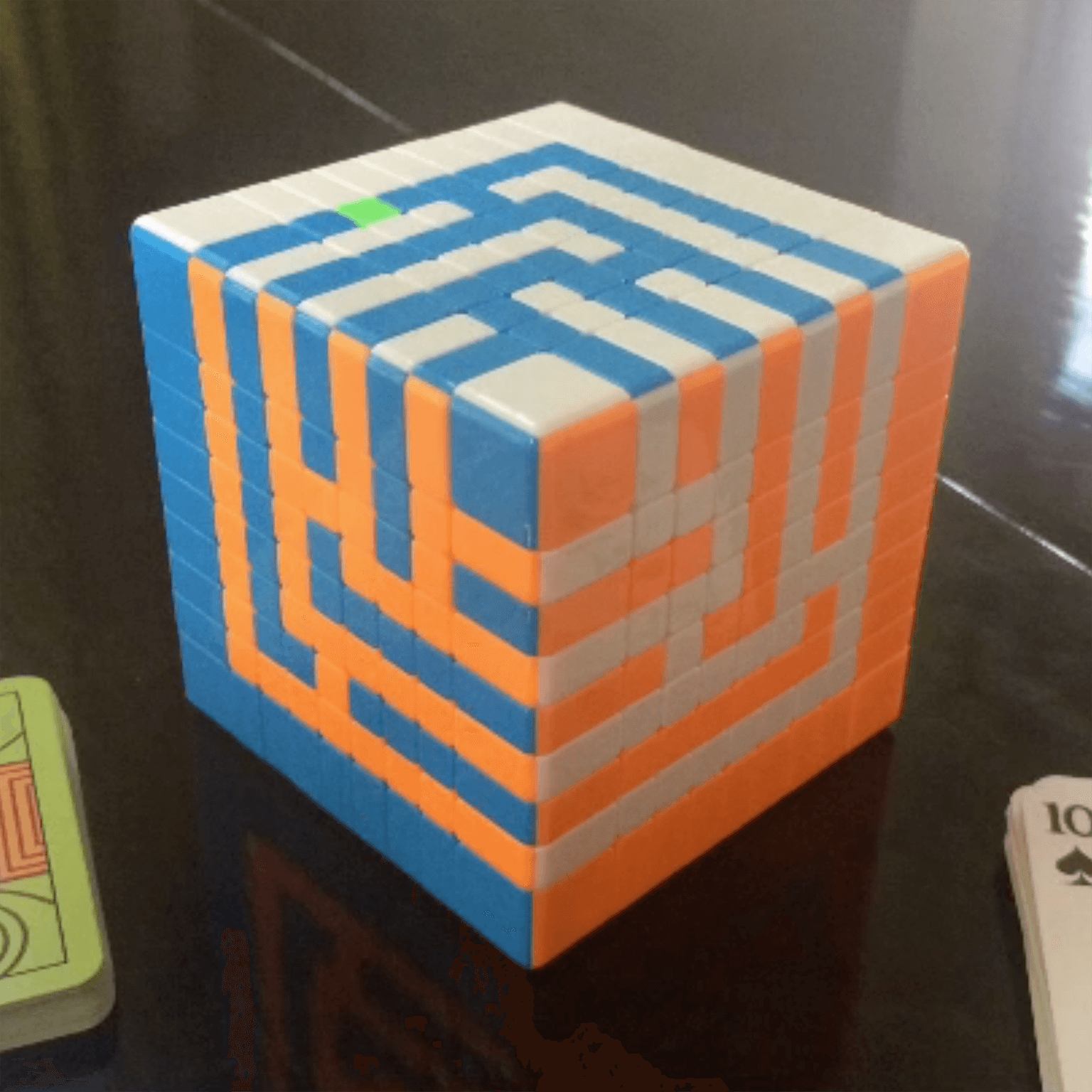
Traversing the Labyrinth
15 x 25 x 1 cm
Stickerless MoFang JiaoShi MF9 9x9x9 Puzzle Cube, Stop-motion software, iPad
2018
This piece is a looped stop-motion video of a 9x9x9 MoFang cube in which a labyrinth forms across three faces and a single pixel traverses from the perimeter, across the three faces, and to the center of the labyrinth where it then expands to fill the maze. As the stop-motion video elapses, a deck of cards is turned over (one card per frame) until revealing the joker card at the end of the video. I intend to present the piece looping on an iPad alongside other works from the project. The entire video can be seen at: www.instagram.com/p/BnSDSaAhpYD