2019 Joint Mathematics Meetings
Steve Trettel
Artists
Steve Trettel
Graduate Student
Math Department, UC Santa Barbara
Santa Barbara, California, USA
Statement
I use Mathematica to visualize low-dimensional objects which come up in my work in geometric topology. I am particularly interested in visualizing tilings, patterns and Cayley graphs associated to discrete groups in dimensions 2 and 3, as well as surfaces in the three sphere and hyperbolic space.
Artworks
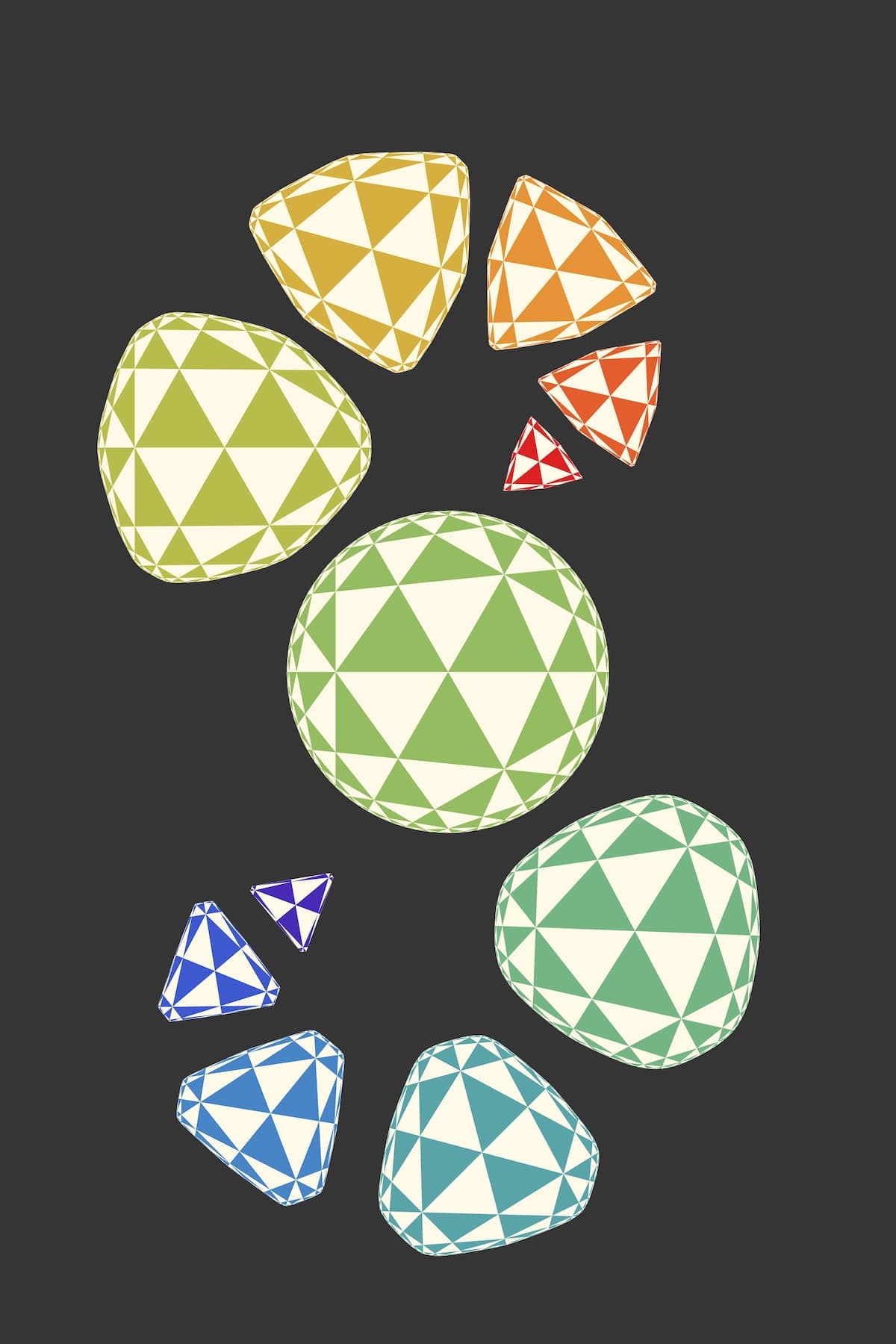
Projective Tilings
50 x 40 cm
Print on Metal
2018
Triangular tilings of the hyperbolic plane produce beautifully symmetric patterns. The theory of real convex projective structures provides a way to deform a triangle tiling of the hyperbolic plane (realized as a disk in projective space) to tilings of other convex domains. This artwork came out of my desire to concretely visualize some of these deformations, and displays eleven different projective domains with fundamental domains for an action of the (3,3,4) triangle group.
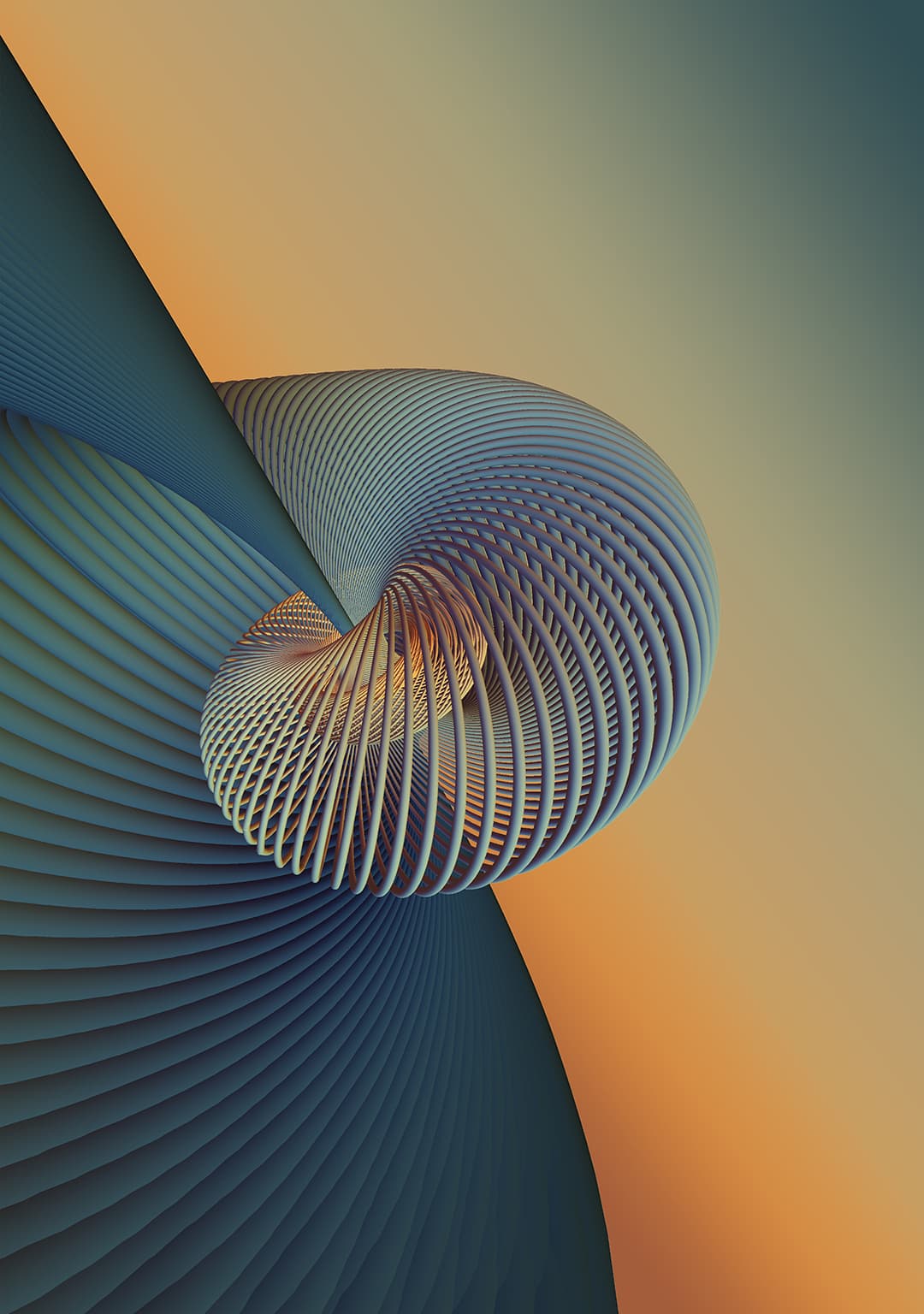
The Hopf Map
50 x 40 cm
Print on Metal
2017
The Hopf map from the three sphere onto the two sphere induces a fibration of S^3 by circles. These circles can be used to create many beautiful symmetric patterns inside the three dimensional sphere, which we can then visualize under stereographic projection. This piece visualizes some of the circles arising from the preimage of a loxodromic curve on the sphere.