2021 Joint Mathematics Meetings
John Nicholson
Artists
Statement
As a child, I was interested in mathematics and art. Eventually, I discovered computers and programming. Once I realized I could connect computer programs to both math and art, I spent many hours writing many small and large programs that could generate all types of images including fractals, 3d renderings, complex curves, and generative art. I continue to explore the intersection of math, art and computer science, both as a pastime and as a way to inspire the students in the computer science courses I teach.
Artworks
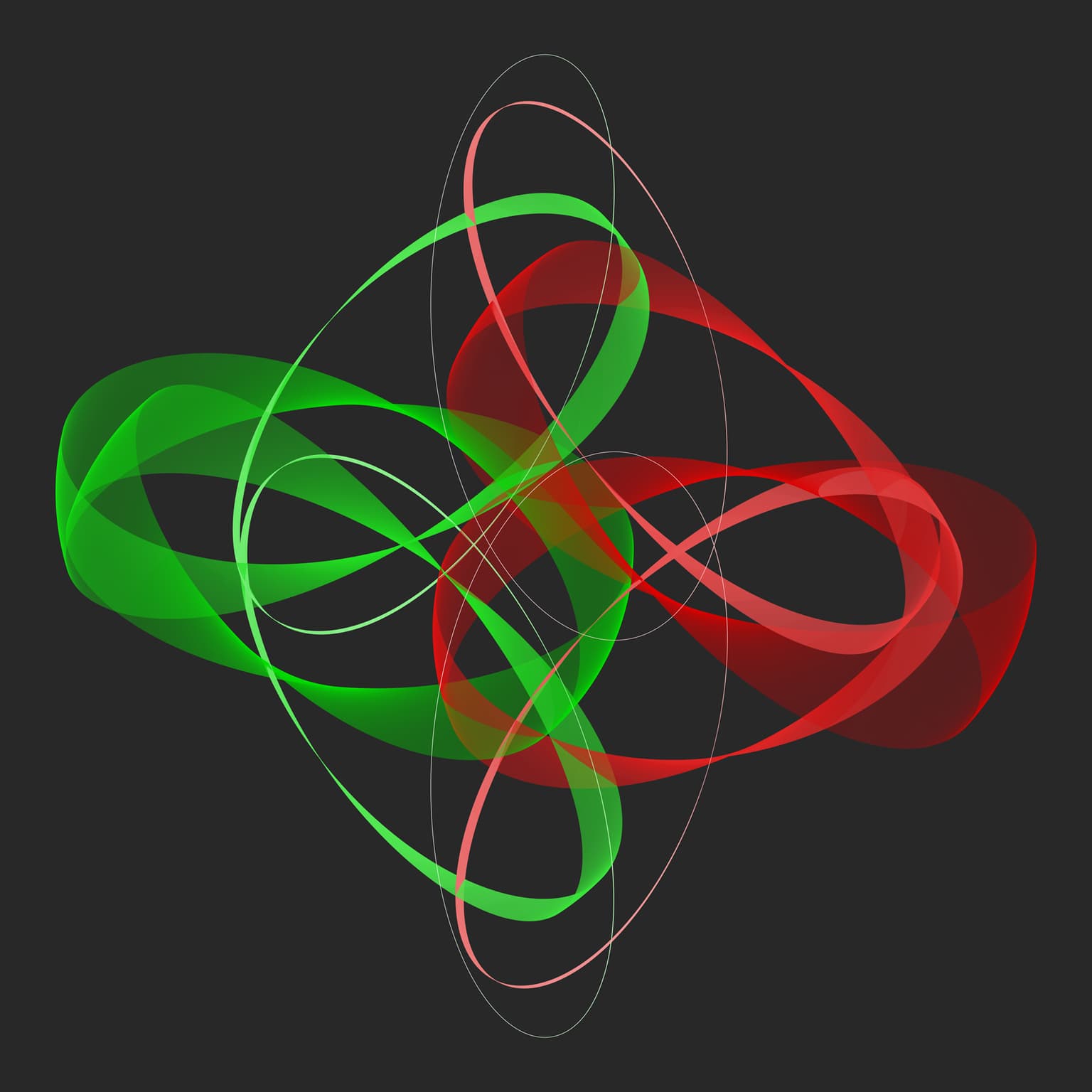
Epicycloid variation
41 x 41 cm
Inkjet on Aluminum
2020
This work is an artistic experiment in which I extend the idea of the epicycloid curve to create a flowing, non-symmetrical path. A traditional epicycloid is created by tracing the path of a point on the circumference of a circle that is itself rolling around the circumference of another circle. In this experiment, the number of circles is increased from two to four resulting in a path that is more complex than the traditional two-circle curve. A secondary modification to the process varies the rotation speed of one of the circles rather than keeping it constant as is normally done.