Dániel Erdély
Artists
Dániel Erdély
Freelance artist, anthropologist
Spidron Bt., Option Ltd
Budapest, hungary
Statement
My task is research and develop geometrical basis of thinking, establish human communities, link art and science.
Artworks

Sphidron deformation of a disc 2.
240 x 320 mm
pastel on paper
2010
The arms of the Sphidron disc are rotating around themselves. The longer distance from the center increases the measure of the rotation. Here the change of the rotation is continuous while in the case of Spidrons it is discrete. All of he spiral arms remain on their own plane, but these planes on which they lie on can be lifted up and pushed down alternately. This way you can make more and more dense surfaces.

Sphidron deformation of a disc
240 x 320 mm
Computer graphic
2010
This work of art is a common effort of Mr. János Erdős and me. János made excellent animations of the deforming sphidron discs. You can read more about our investigations here: www.spacecollective.org/edanet
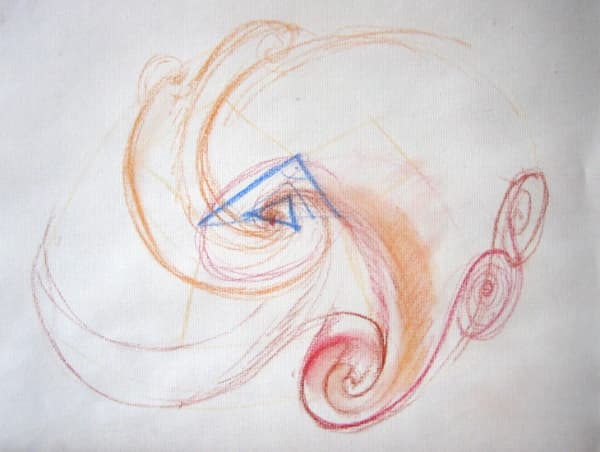
Sphidron deformation of a disc 1.
240 x 320 mm
pastel on paper
2010
I have found the only possible 3D deformation of the Euclidean plane which - regarding of the 9 vertices of the basic shapes of the Pythagorean Theorem, like the triangle and the squares - is preserving the validity of the theorem. The triangle in the 2D plane is rotating and shrinking, but its proportions and angles don't change. I tried to make some drawings to demonstrate this interesting swirling deformation.