2011 Joint Mathematics Meetings
Andrzej k Brodzik
Artists
Statement
One of the main goals of science, art and philosophy is to reveal fundamental principles, essential representations, and meaningful connections. This task can sometimes be facilitated by juxtaposing structure and randomness, intention and accident. Art that explores these relationships does not function merely as a pleasing illustration of a mathematical principle, but as an independent mode of intellectual interrogation. Indeed, many examples of such explorations can be found not only in contemporary arts, but also, among others, in the traditional designs of Berber veils, Javanese ikats and Asmat shield carvings.
Artworks
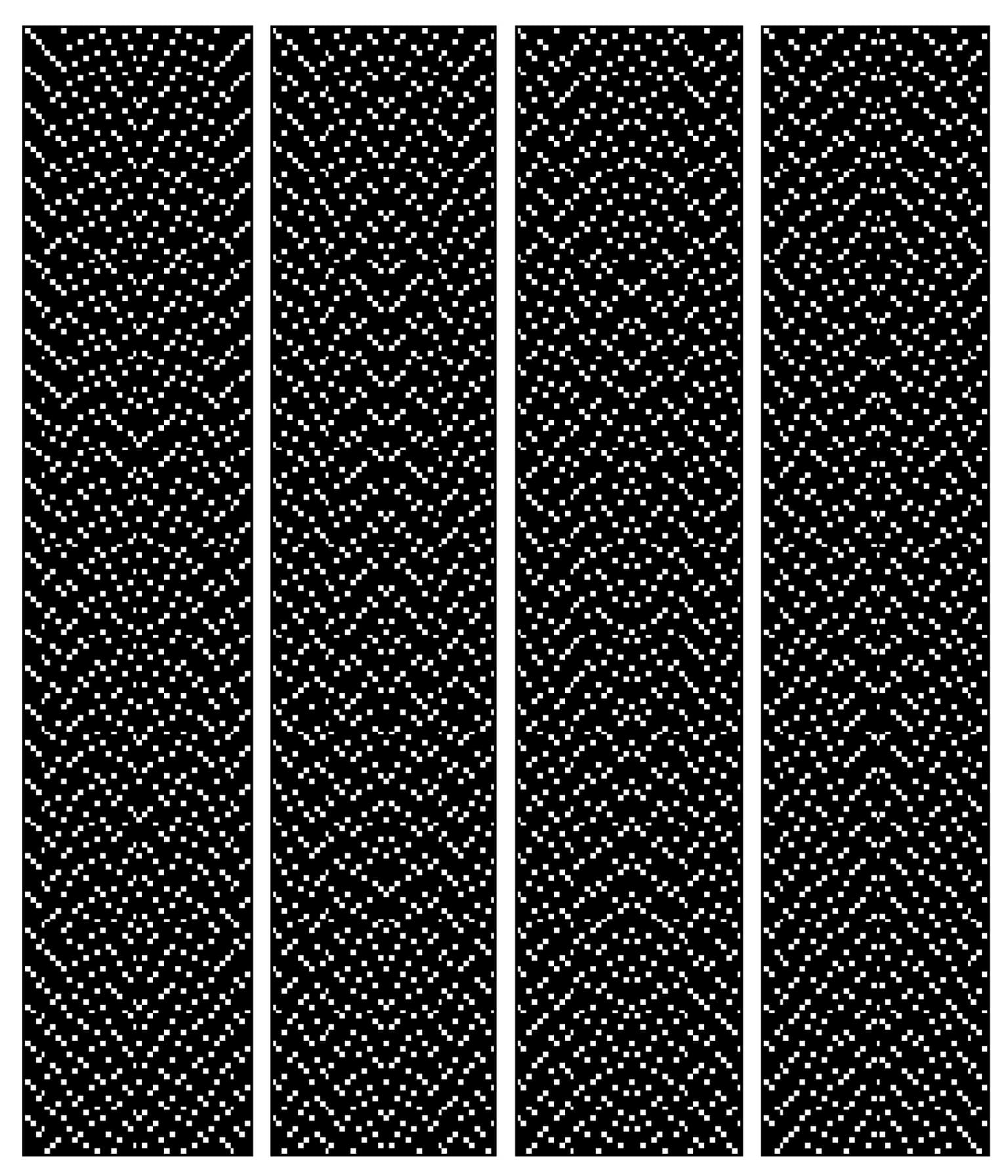
ideal quilt, slightly imperfect
24'' x 20''
digital print
2010
Ideal quilts are Zak space representations of families of ideal sequences. Ideal sequences are sequences with certain special group-theoretical properties. In particular, ideal sequences satisfy the Sarwate bound, having both zero out-of-phase autocorrelation and minimum cross-correlation sidelobes. Construction of ideal sequences was described in the recent book, Ideal sequence design in time-frequency space. Ideal quilts are (p-1)p by (p-2)!p images, where p is a prime. As these images tend to be long and narrow, to facilitate display, they are usually divided into columns. Geometrically, an ideal quilt is a sequence of distinct permutations of the canonical image of a diagonal line. Both the overall structure of the image and the association with ideal sequences convey a strong sense of symmetry, predictability, and uniqueness. To counter-balance these qualities, the corrupting effect of tiff data compression, manifested as pixel distortion, is embedded into the image.