2011 Joint Mathematics Meetings
Vladimir Bulatov
Artists
Vladimir Bulatov
Mathematics and Arts Explorer
Corvallis, Oregon
Statement
My artistic passions are purely mathematical images and sculptures, which express a certain vision of forms and shapes, my interpretations of distance, transformations and space. In my opinion, mathematics is a way of thinking, a way of life. My images and sculptures are like photographs of interesting mathematical ideas, which I try to dicover and to bring to the real world. I have always been intrigued by the possibility of showing the intrinsic richness of the mathematical world, whose charm and harmony can really be appreciated by everyone.
Artworks
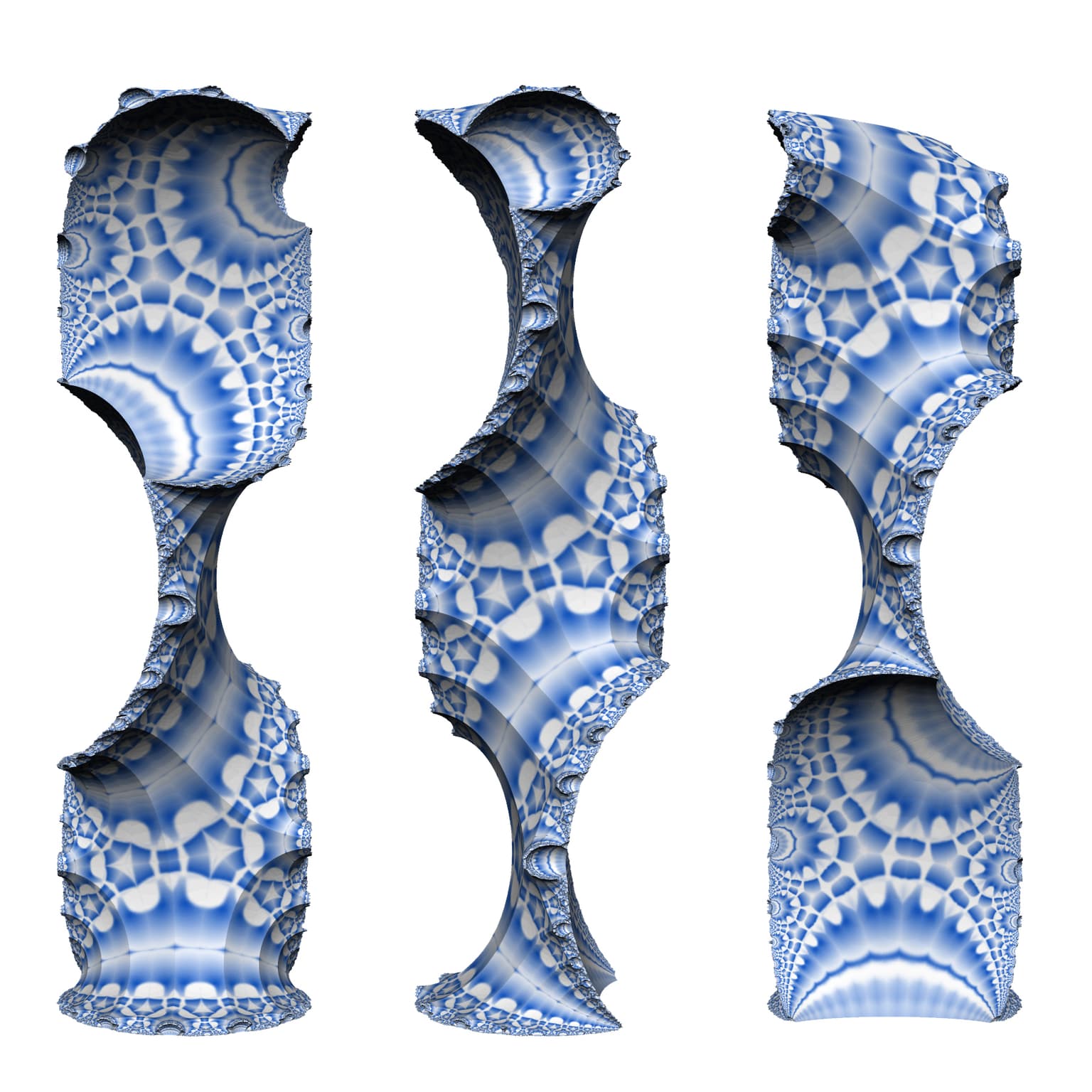
Hyperbolic Tiling I
200mm x 60mm x 60mm
Rapid prototyping sculpture
2010
This is a visualization of a tiling of the hyperbolic space. The tiling is generated by reflections in the faces of Lambert cube (Coxeter polyhedron) which becomes the fundamental polyhedron of the symmetry group of the tiling. Only 4 out of 6 sides are used, which results in sub-tiling (subgroup) filling only part of the space. It let us see the internal structure of the tiling.
We use cylinder model of the hyperbolic space - a 3D generalization of 2D band model. In this model the Poincare ball is stretched into infinite cylinder. Cylinder's axis becomes one of hyperbolic geodesics.
The tiling is oriented to make one it's plane to be orthogonal to the cylinder's axis to have a feet to stand on.
The cylinder's axis is close to the axis of a loxodromic transformation of the group, which gives the pieces its spiral twist. The sharp boundary of the piece corresponds to the limit set of the group. The limit set is fractal
Jordan curve at the infinity of the hyperbolic space.