Hongtaek Hwang, Sol Oh* and Ho-gul Park
Artists
Hong-taek Hwang
Artist, Emeritus Professor
Kumoh National Institute of Technology
Gumi, South Korea
Statement
I enjoy creative activities on the boundary between Math and Art. Sometimes I create artwork with geometrical tube design; at other times I enhance a math visual model to the point where it becomes a piece of art. I have designed “Stars over the Alhambra’s Palace”, “Islamic Tessellation”, and etc. with tubes. We are developing the spherical versions of tube designs according to the following scheme: First, we observe and analyze the designs of the soccer ball "Telstar" and the "Park's sphere". Second, by mathematical thinking, we get various generalized imagination of the geometrical model of the Telstar. Last, through a series of tube design experiments, we get the creative realities about the mathematically generalized imagination.
Artworks

A spherical harmony of horizontality and verticality
7.9''x7.9''x7.9''
Geometric Tube Design
2011
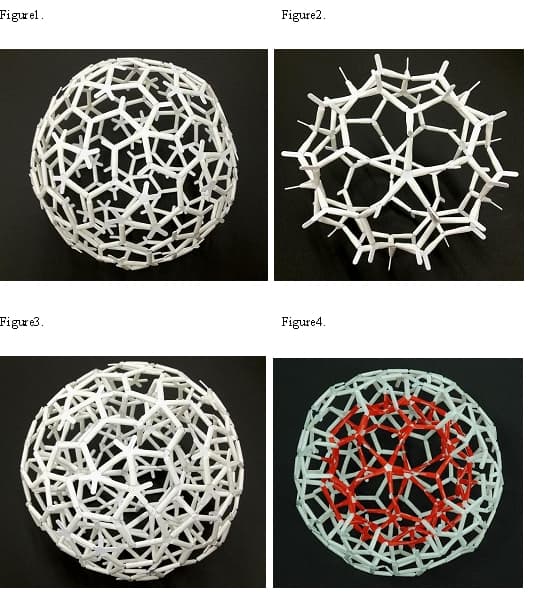
Explanation of “A spherical harmony of horizontality and verticality”
24''x24''
Geometric Tube Design
2011