2012 Joint Mathematics Meetings
Chern Chuang, Bih-Yaw Jin, Wei-Chi Wei
Artists
Bih-Yaw Jin
Professor
Department of Chemistry, National Taiwan University
Taipei, Taiwan
Statement
Geometry is an essential ingredient of chemistry. The functionality of molecules depends heavily on their geometries. As chemists, we are interested in the connection of one type of molecules, the fullerenes composing of graphitic carbon, and its beaded counterpart. As one of the authors (BYJ) demonstrated in the Bridges conference last year, fullerene molecules are particularly suited to be models of making bead crafts. And the resulting beaded molecular models are both faithful representations of the molecules themselves and artistically appealing. Here we present two beaded molecules that correspond to the classic triply periodic minimal surfaces (TPMS), Schwarz's D and Schoen's G surfaces. These TPMSs are widely known to the chemical, material and mathematical societies, and are ubiquitous in Nature. By suitably inserting octagons into regular hexagonal honeycomb and introducing periodic boundary condition in all three dimensions, one obtains the fullerene correspondences of the TPMSs. In particular, octagons are represented by colored beads while hexagons are white in these beaded models.
Artworks

Beaded Fullerene of Schwarz's D Surface
23cm x 21cm x 18 cm
Faceted plastic beads and fish thread
2008
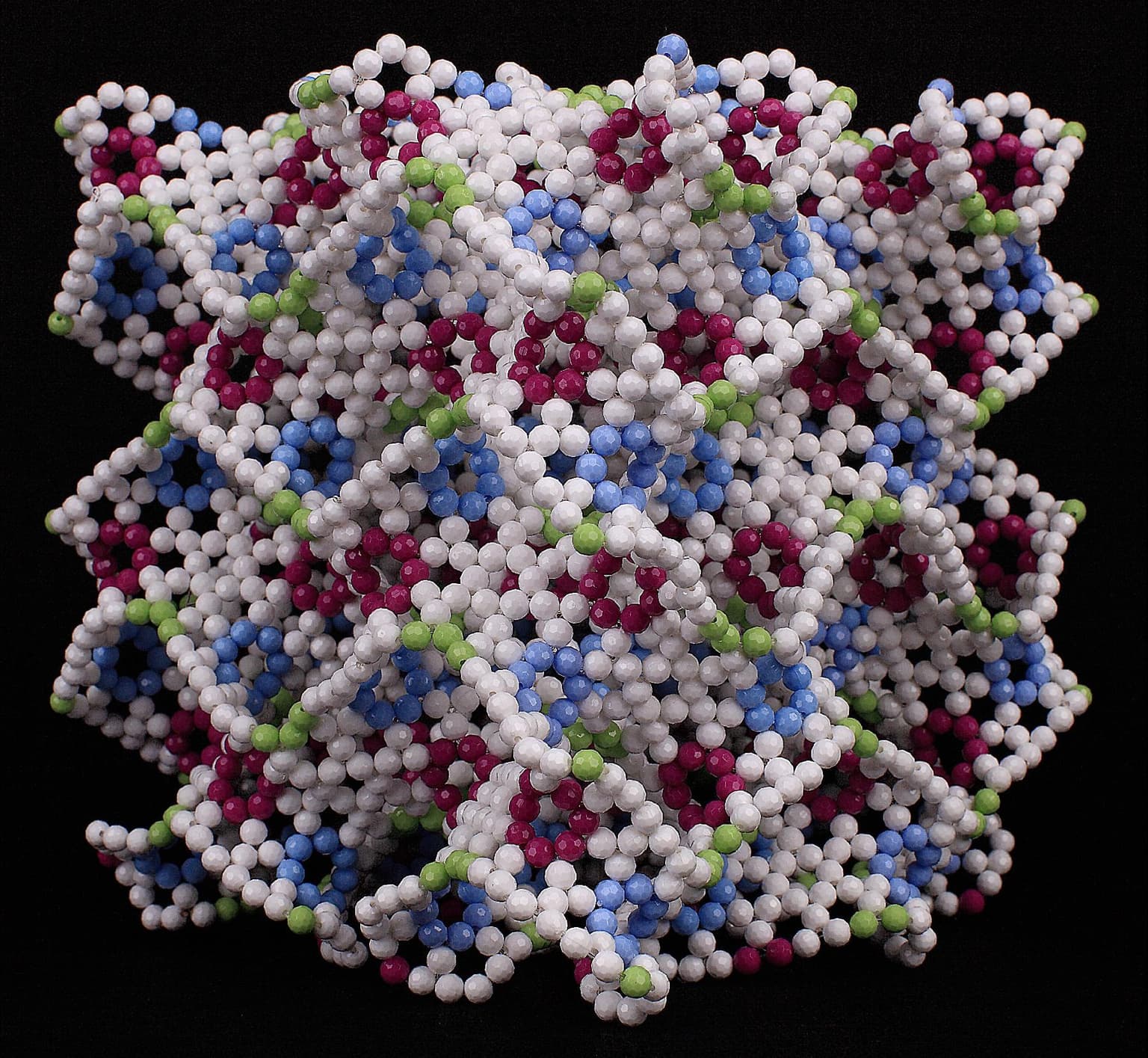
Beaded Fullerene of Schoen's G Surface
18.5cm x 18.5 cm x 20cm
Faceted plastic beads and fish thread
2011