2012 Joint Mathematics Meetings
Doug Dunham
Artists
Doug Dunham
Professor Emeritus of Computer Science
Dept. of Computer Science, University of Minnesota Duluth
Duluth, Minnesota, USA
Statement
The goal of my art is to create repeating patterns in the hyperbolic plane. These patterns are drawn in the Poincare circle model of hyperbolic geometry, which has two useful properties: (1) it shows the entire hyperbolic plane in a finite area, and (2) it is conformal, i.e. angles have their Euclidean measure, so that copies of a motif retain their same approximate shape as they get smaller toward the bounding circle. Most of the patterns I create exhibit characteristics of Escher's patterns: they tile the plane without gaps or overlaps, and if colored, they are colored symmetrically and adhere to the map-coloring principle that adjacent copies of the motif are different colors. My patterns are rendered by a color printer. Two challenges are to design appealing motifs and to write programs that facilitate such design and replicate the complete pattern.
Artworks
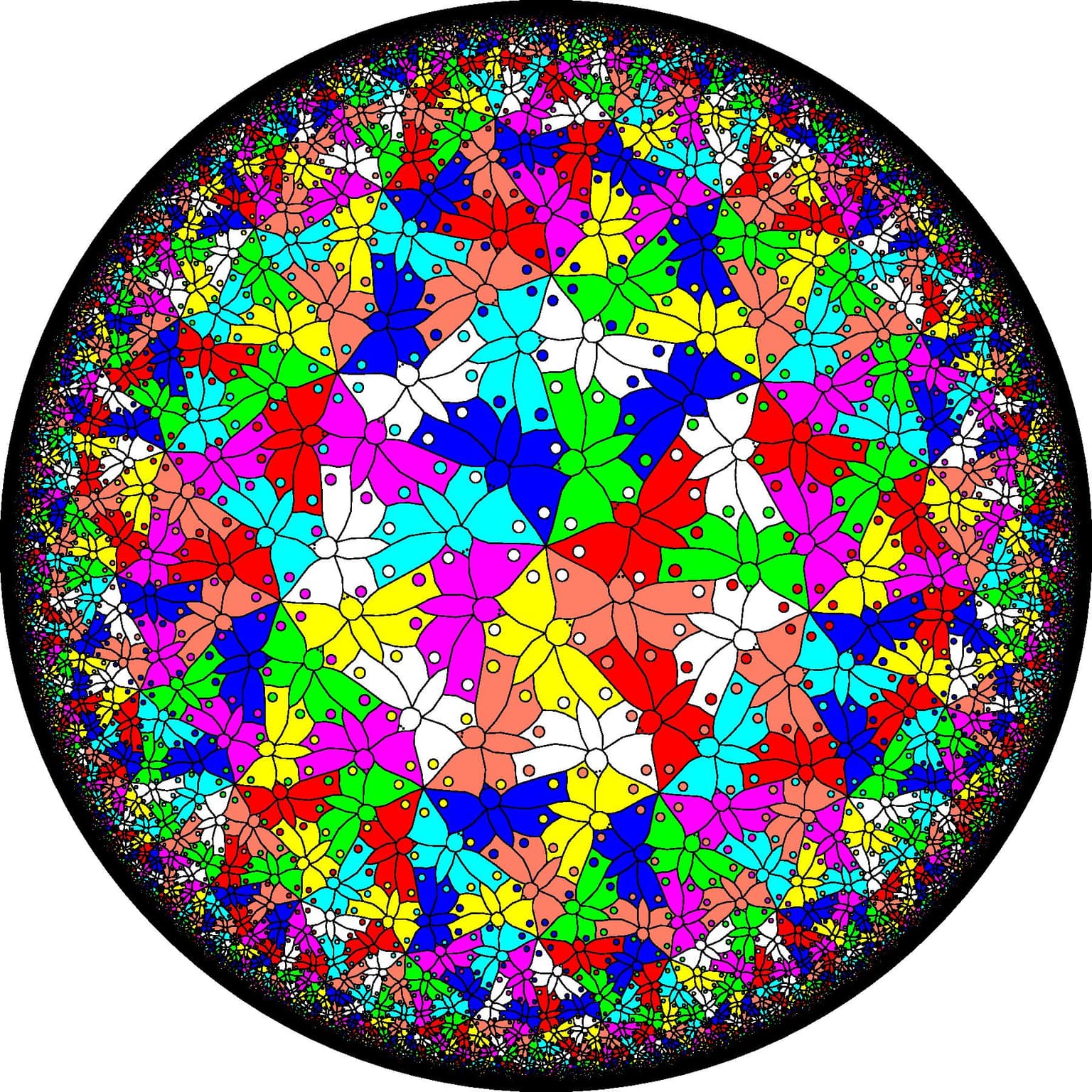
Butterflies 7-3
11 by 11 inches
Color printer
2009
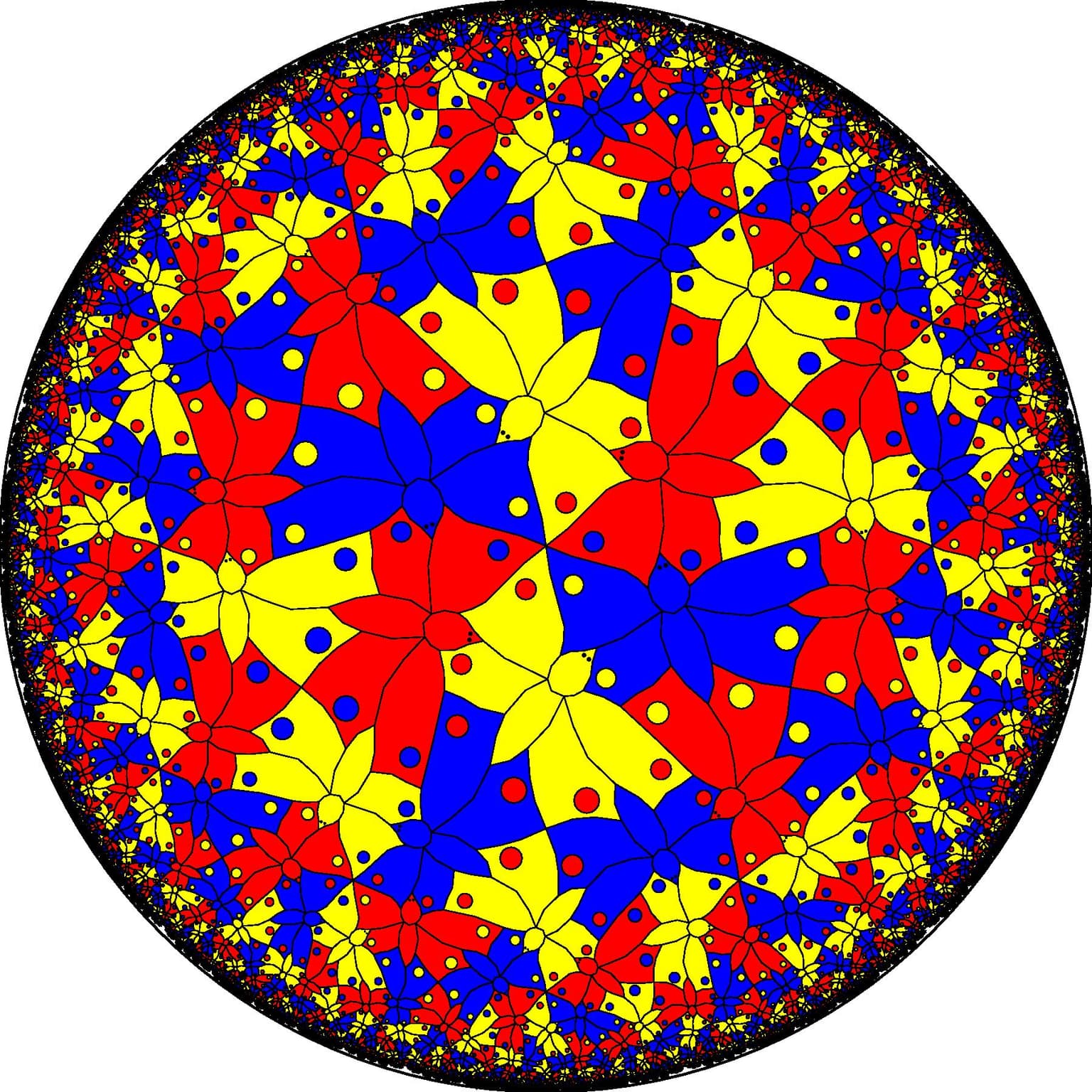
Butterflies 6-4
11 by 11 inches
Color printer
2009