2012 Joint Mathematics Meetings
Henry Segerman
Artists
Henry Segerman
Associate Professor of Mathematics
Oklahoma State University
Stillwater, Oklahoma, USA
Statement
I am a postdoctoral mathematician. My mathematical research is in 3-dimensional geometry and topology, and concepts from those areas often appear in my work. Other artistic interests involve procedural generation, self reference, ambigrams and puzzles. These sculptures were designed with the assistance of Saul Schleimer.
Artworks
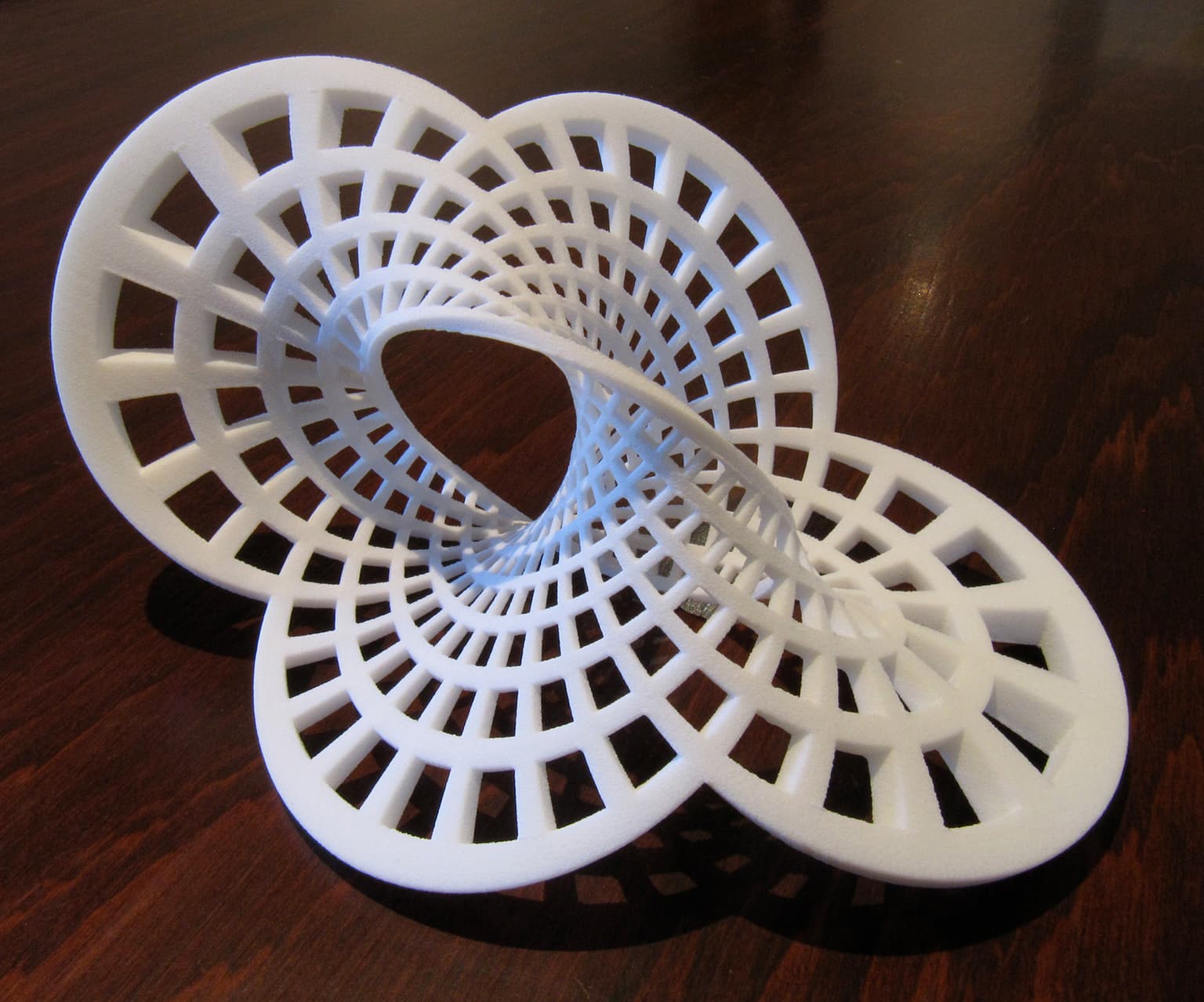
Round Möbius Strip
152 x 62 x 109 mm
PA 2200 Plastic, Selective-Laser-Sintered
2011
The usual version of a Möbius strip has as its single boundary curve an unknotted loop. This unknotted loop can be deformed into a round circle, with the strip deformed along with it. This shows a particularly symmetric result. The boundary of the strip is the circle in the middle, and the surface "goes through infinity", meaning that the grid pattern should continue arbitrarily far outwards. To save on costs, I have removed the grid lines that would require an infinite amount of plastic to print.

Round Klein Bottle
152 x 152 x 109 mm
PA 2200 Plastic, Selective-Laser-Sintered
2011
This is made by gluing two copies of the Round Möbius Strip along their boundaries. A Klein bottle in 3-dimensional space must intersect itself, and in this case it intersects itself along a straight line. These sculptures are based on parameterisations of the surfaces, given by trigonometric functions in the 3-dimensional sphere, viewed as the unit sphere in 4-dimensional Euclidean space. The designs are then mapped into 3-dimensional Euclidean space by stereographic projection, so that they can be printed.