2012 Joint Mathematics Meetings
john hiigli
Artists
john hiigli
artist/educator
Le Jardin a l'Ouest : Jardin Galerie
NYC, NY
Statement
I am a transparent/geometric painter and creator of math/art materials for the early childhood classroom. I am affiliated with the International Symmetry Association (Budapest, Hungary), the International Bridges Organization, the Experience Workshop (Pecs, Hungary) and the Synergetics Collaborative (USA).
Artworks

KALEIDOSCOPE - Derived from VIRUS XIX (Chrome 41)
10' X 10'
Opaque Oil Paint on Cotton Canvas
2007
Mac software CI Kaleidoscope Filter used to produce a kaleidoscopic image
from a source image (earlier painting : Chrome 41). By applying 24-way
symmetry the filter repeats a 15 degree wedge from the input image (center
Chrome 41). Kaleidoscope Painting exhibits rotational symmetry (12-fold
symmetry axis), reflection symmetry (12 symmetry axes at 30 degrees),
dodecagon bands as the repeating unit.
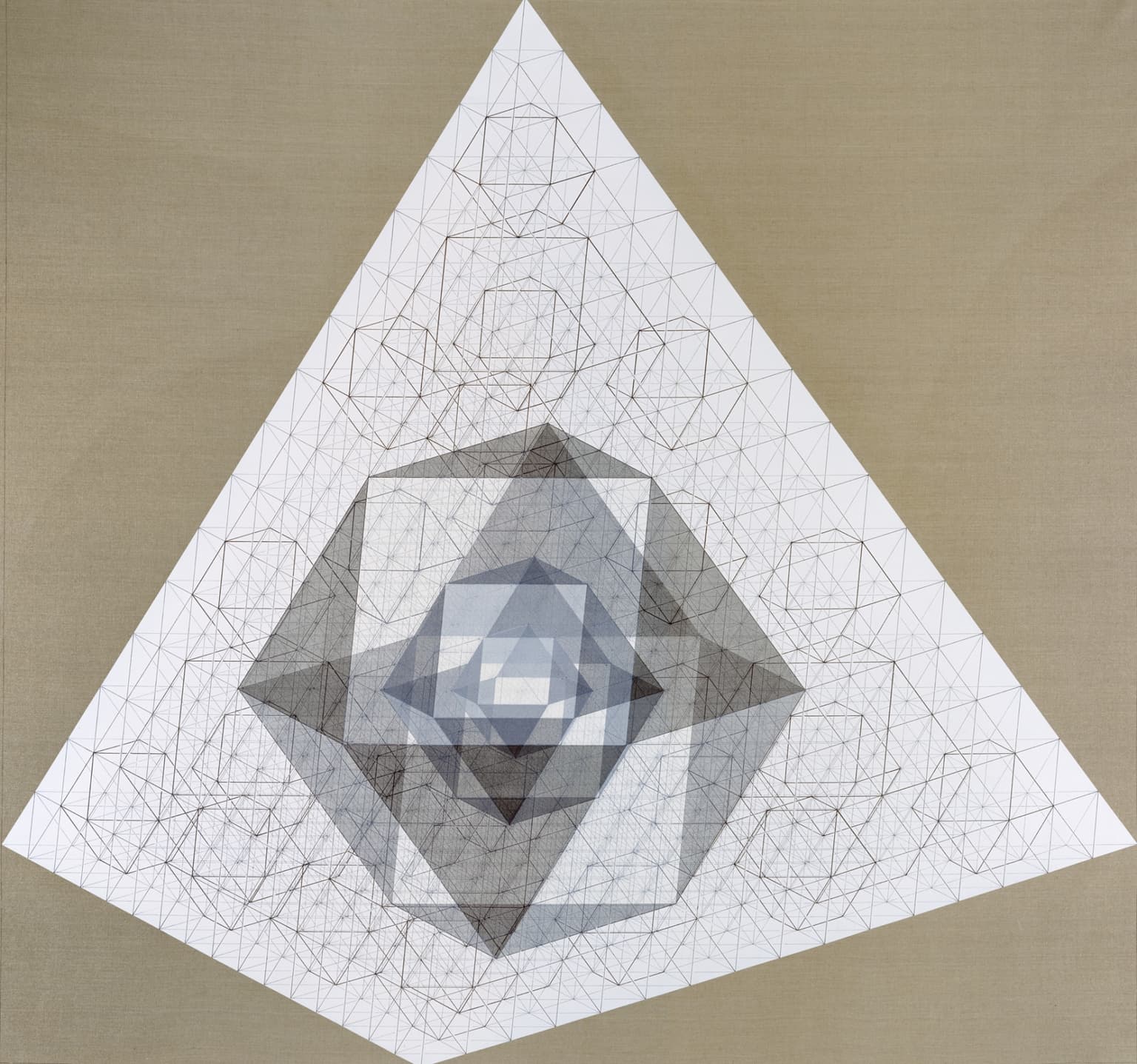
Chrome 194
60 X 64 in
Transparent Oil on Linen Canvas
2011
Through a process known as subdivision a tetrahedron can be broken down to infinity. Subdivision produces the cyclical growth of other structures, such as the cube octahedron (CUO). The lowest frequency tetrahedron capable of “growing” a cube octahedron is the four-frequency tetrahedron. The eight-frequency tetrahedron produces a two-frequency cube octahedron as well. The sixteen-frequency tetrahedron produces a nuclear CUO, a nuclear two-frequency CUO and a nuclear four-frequency CUO. Thus we can say that the sub-division of polyhedra manifests a natural space characterized by transformation and change of scale.
Scale change involves systems in which the size of individual forms vary incessantly while the proportions and characteristic shapes of these forms remain constant. These forms and their proportions can be grouped in a regular sequence in which the elements of the sequence constitute an infinite series. Such "scale-invariant” sequences occur in nature (the leaf's nervure, the laceration of the fern, the widening or narrowing line of the snail shell, the shoreline of the continents, etc). This "invariability" is also a characteristic of fractal geometry. In the Isotropic Vector Matrix the potential for scale change is related to infinite transformation. With every doubling (or halving) of edge-length the volume increases (or decreases) by eight (8), equivalent to the octave in music. The artistic strategy of using transparent oil paint makes it possible to explore and communicate this world of higher dimensions.
Scale shifting, or scale change, as well as the repetitious logic enabling it, is known in mathematics as “iteration,” hence the term “iterative mathematics/geometry”. It is a particular feature of IVM that is a useful tool in both mathematics and art education. In particular scale change provides a tool of measurement with which to evaluate angle & distance information.