2012 Joint Mathematics Meetings
Karen M. Strom
Artists
Karen M. Strom
Artist/Photographer
none - retired
Sonoita, AZ
Statement
As a scientist, my eyes and mind have been trained to respond to patterns in the natural world. These patterns result from the response of complex systems to physical laws, whose ultimate expression is cast in mathematical form. Mathematical forms -- be they equations or geometrical constructs -- evoke a sense of beauty, much like a poem or symphony. But the world of nature, while shaped by physical laws, is inherently chaotic. My goal in this series of images is to use the worlds of mathematical perfection and beauty to show the equally profound beauty of the natural world. Tiles are constructed perfection. Unlike the strictly periodic tiling patterns used to construct flooring and wall tiles, these tile patterns are aperiodic, constructed using only two shapes of tiles (e.g. kites and darts). Coherent blocks of these tiles may reappear throughout a large pattern but do not do so in a periodic manner. Tiling theory can be used to study crystal structure and methods to most efficiently fill a surface or a volume. In these images tile patterns generated by mathematical algorithms are overlaid on complex natural systems operating in the real world. These systems, both biological and chemical, adjust to efficiently grow using the space they have available. What I hope to evoke is a new, constructed world in which disordered nature is re-imagined through the eyes of mathematical order and perfection -- creating in the mindʼs eye a new beauty that is neither order nor chaos.
Artworks
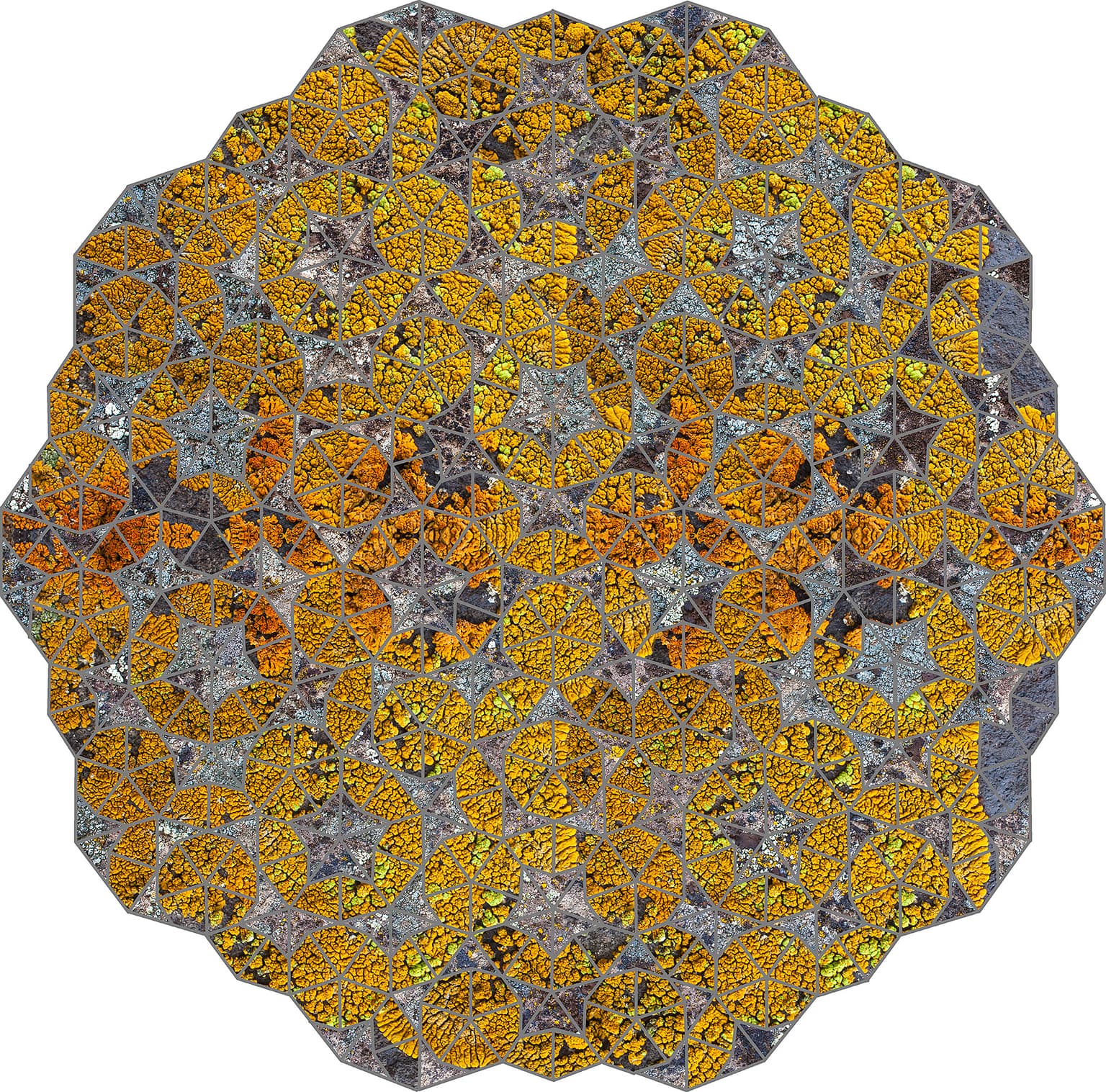
Lichen - Smith Rock State Park, OR
24" x 24"
archival pigment print
2010
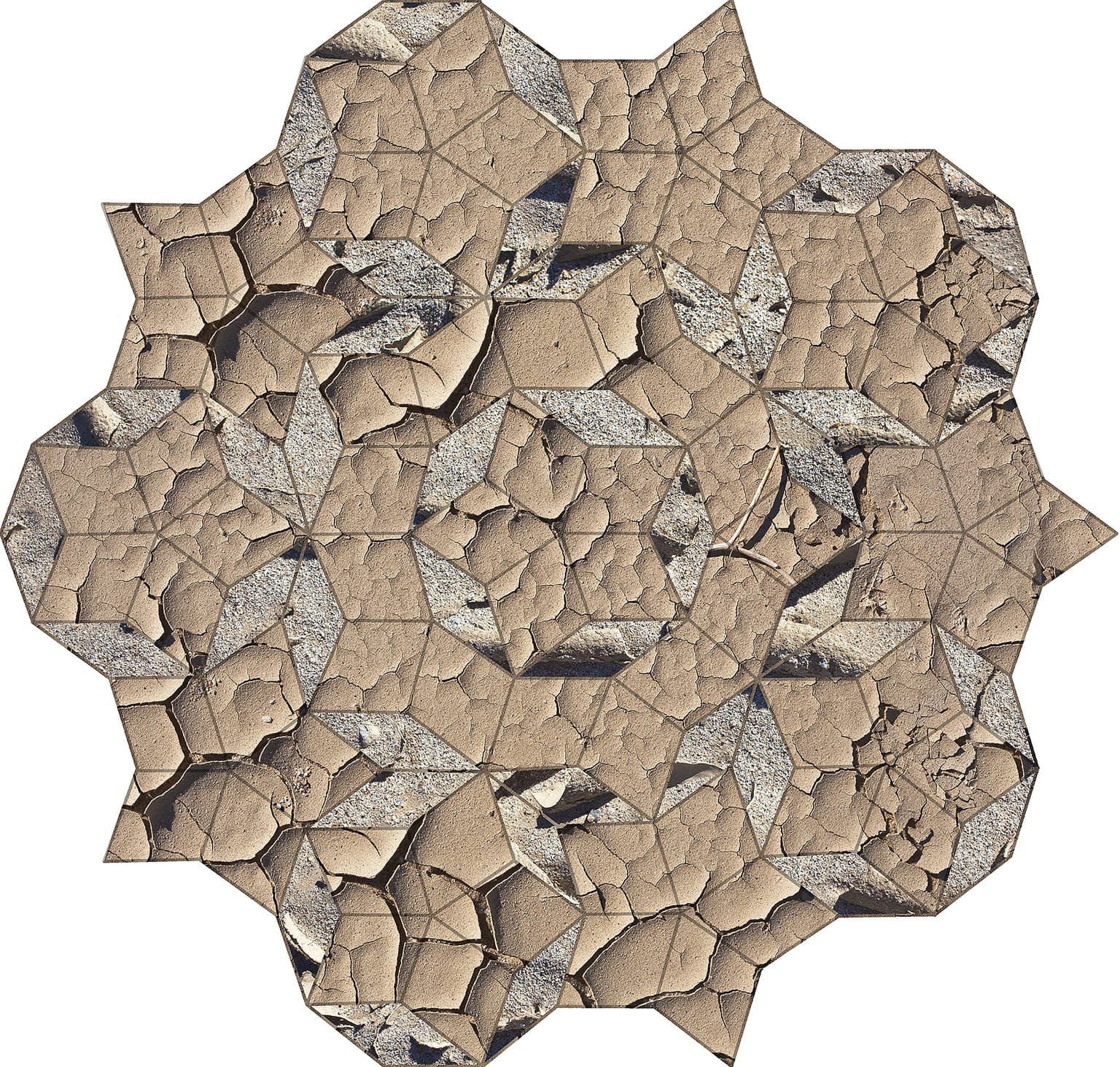
Fish Creek Wash 1
24" x 24"
archival pigment print
2011