2012 Joint Mathematics Meetings
Larry Riddle
Artists
Larry Riddle
Professor Emeritus of Mathematics
Agnes Scott College
Decatur, GA
Statement
I am a mathematician interested in the fractal geometry of iterated function systems. I enjoy using a software program I wrote called IFS Construction Kit to create mathematical designs (both digital images and counted cross-stitch pieces) that can be used to illustrate the fascinating fractals associated with these systems.
Artworks
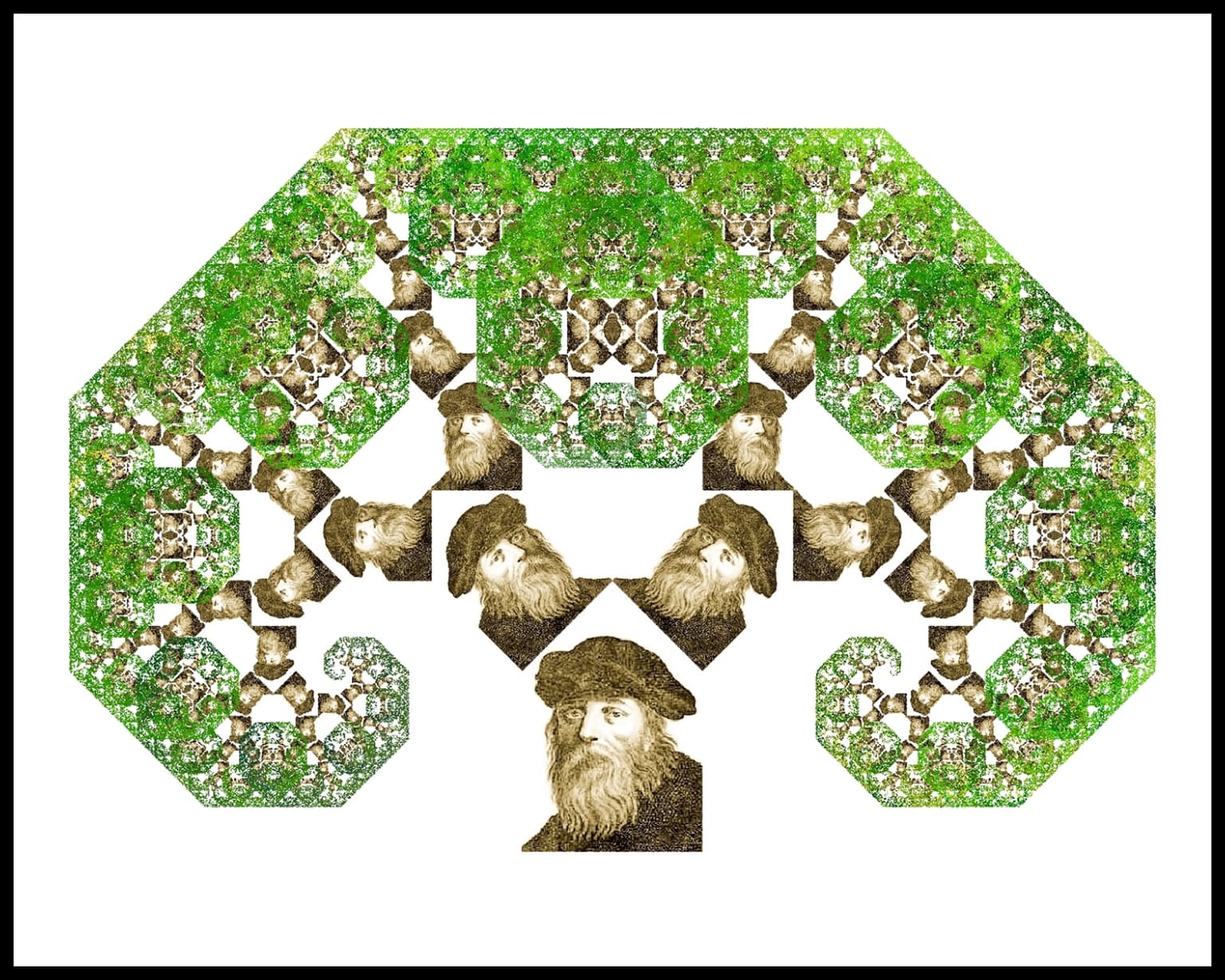
Pythagorean Tree
16" X 20"
Digital print
2011
The traditional Pythagorean Tree is constructed by starting with a square and constructing two smaller squares such that the corners of the squares coincide pairwise (thus enclosing a right triangle), then iterating the construction on each of the two smaller squares. When viewed as an iterated function system, however, one can start the iteration with any initial set. For this image I began with a common picture of Pythagoras as the initial set. The trunk of the tree was constructed using 10 iterations of a deterministic algorithm based on an iterated function system with three functions - the identity function, a scaling and rotation by 45 degrees, and a scaling and rotation by 45 degrees with a reflection. This gives a reflective symmetry for the trunk. The leaves consist of 500,000 points plotted using a random chaos game algorithm and colored based on a "color stealing" algorithm for iterated function systems described by Michael Barnsley in a 2003 paper. To give the leaves a realistic shading, the colors were stolen from a digital photograph of a field of green and yellow grass.