2012 Joint Mathematics Meetings

Thomas Hull, Robert Lang, and Ray Schamp

Artists

Thomas Hull and Robby Kraft

Associate Professor of Mathematics

Western New England University

Tom: Springfield, Massachusetts, USA. Robby: Brooklyn, New York, USA.

thull@wne.edu

Statement

Thomas Hull's statement: I've been practicing origami almost as long as I've been doing math.  Part of the charm of paper folding is its capacity for simple, elegant beauty as well as stunning complexity, all within the same set of constraints.  This mirrors the appeal of mathematics quite well.  Geometric origami, which is where most of my artwork lives, strives to express in physical form the inherent beauty of mathematical concepts in geometry, algebra, and combinatorics.  The constraints that origami provides (only folding, no cutting, and either one sheet of paper or further constraints if more than one sheet is allowed) challenges the artist in a way similar to being challenged be a mathematical problem.

Artworks

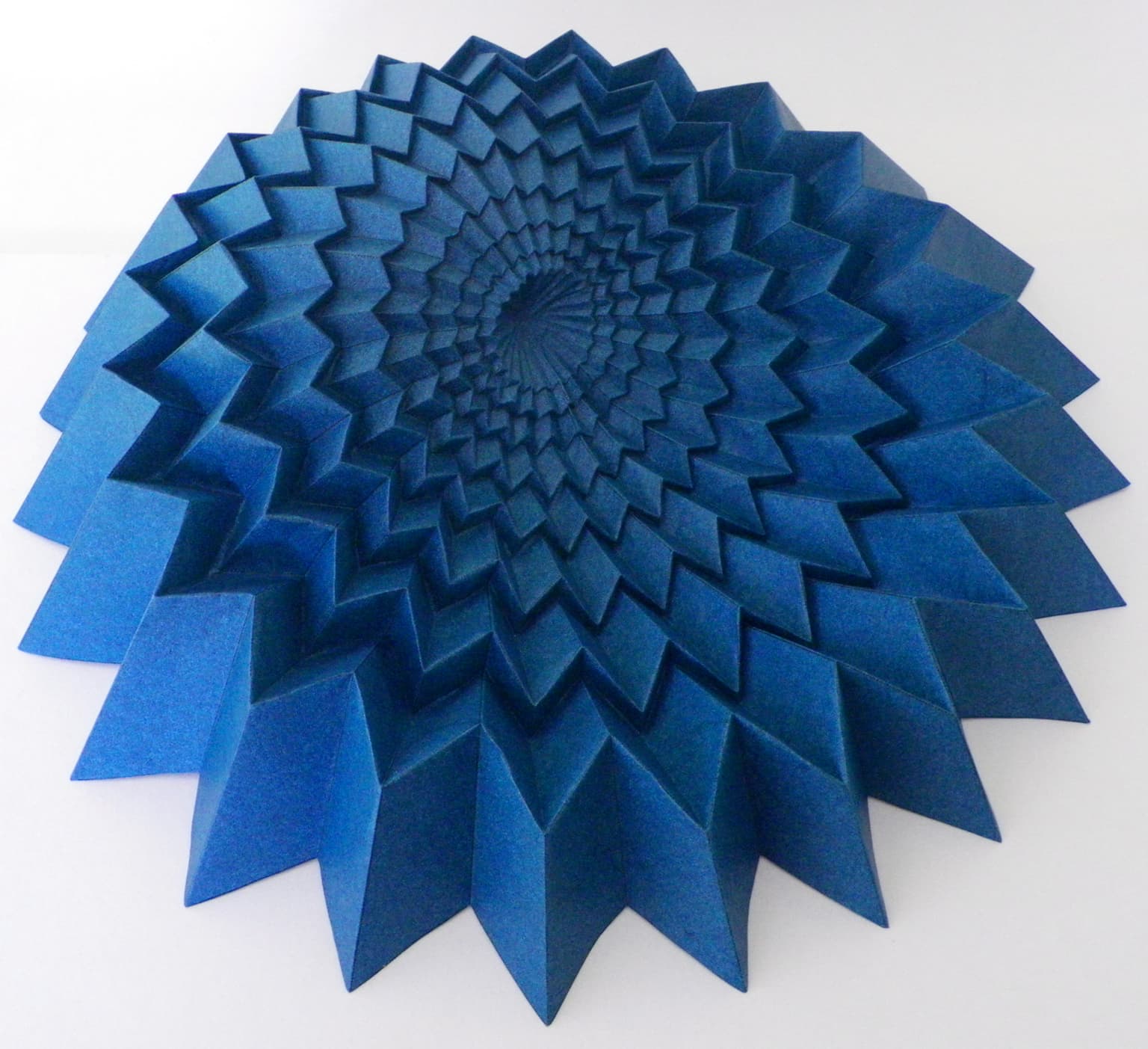
Image for entry 'Pleated Multi-sliced Cone'

Pleated Multi-sliced Cone

16 inches x 16 inches by 5 inches

elephant hide paper

2011

Imagine a long paper cone that is pleated with alternating mountain and valley creases so that its cross-section is star-shaped. Now slice the cone with a plane and imagine reflecting the top part of the cone through this plane. The result is exactly what one would get if we folded the pleated cone along creases made by the intersecting plane. Doing this repeatedly can result in interesting shapes, including the origami version presented here. This work is a collaboration. The concept and crease pattern for this work was devised and modeled in Mathematica by origami artist Robert Lang. The crease pattern was then printed onto elephant hide paper by artist Ray Schamp. The paper was then folded along the crease pattern by mathematician and origami artist Thomas Hull.