Gary Greenfield
Artists
Gary Greenfield
Professor Emeritas of Mathematics & Computer Science
University of Richmond
Richmond, Virginia, USA
Statement
Many of my computer generated algorithmic art works are based on visualizations resulting from simulating mathematical models of natural processes. Examples include cell morphogenesis, reaction-diffusion, and swarm behavior. By experimenting with parameter settings and drawing attributes, I try to focus the viewer's attention on the complexity underlying such processes.
Artworks
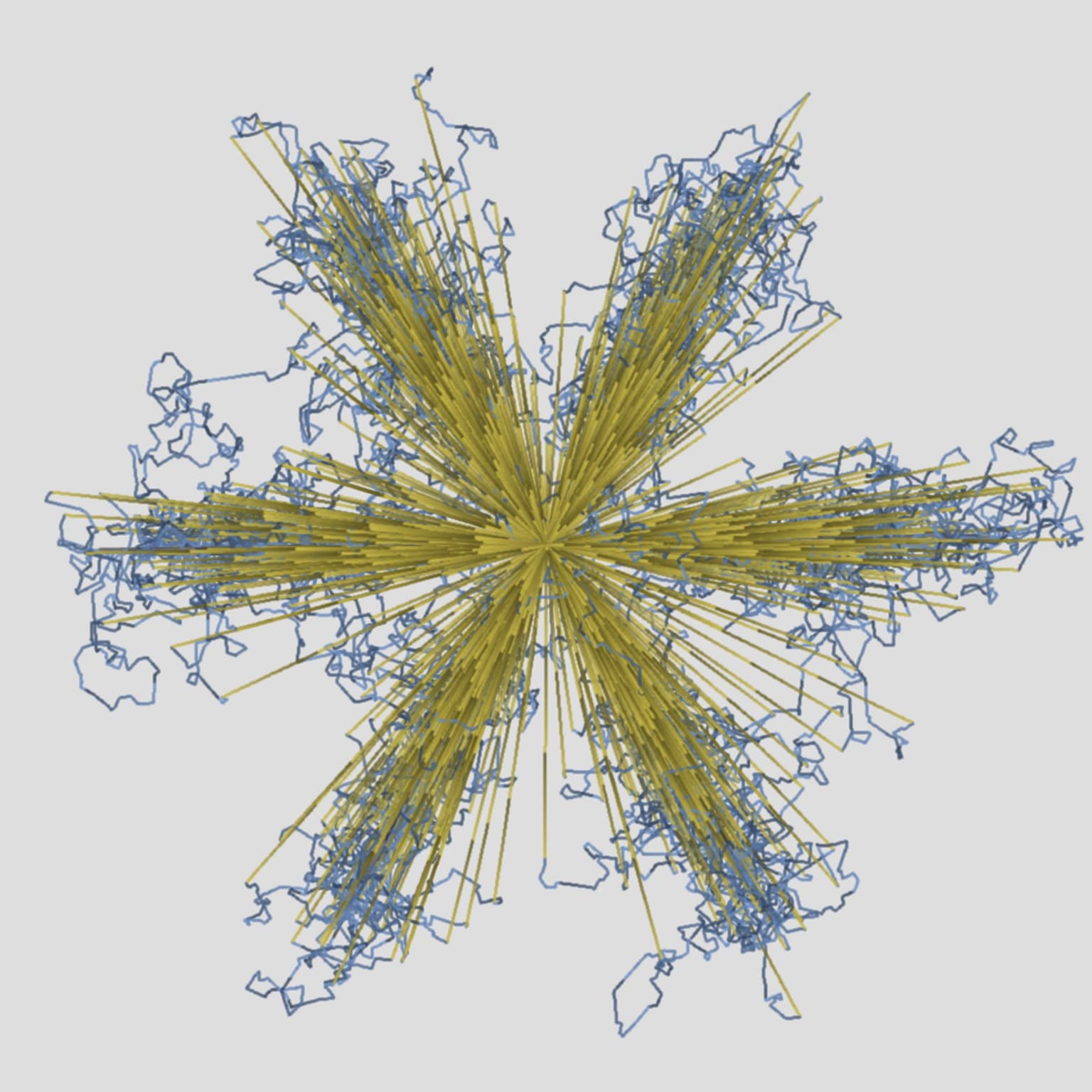
Untitled
10.5" x 10.5" (unframed)
Digital Print
2012
This visualization is based on the foraging behavior of the seed harvesting ant P. barbatus, a species which does not use pheromone trails for finding food. Here, 1000 ants stream out of the nest along six patroller trails and then intermittently break-off to initiate random searches for seeds. When seeds are encountered ants collect them and return directly to the nest. The simulation lasts for only 500 time steps. In nature, typically 1800 ants are foraging at any one time, the patroller trails are not uniform in length or uniformly separated, and foraging continues for several thousand time steps thus obscuring the foraging patterns and structure we are able to observe and recover using our more modest set-up. Color gradations are used to disambiguate the search phase from the return phase and reinforce the dynamic aspects of the process.