José L. Rodríguez
Artists
José L. Rodríguez, Diego Cangas
Associate Professor of Mathematics
Department of Mathematics, University of Almería
Almería
Statement
I like to make geometrical and topological models by hand, mainly thought for manipulation, so useful to know and understand their properties. My students at the unversity enjoy them a lot and they often colaborate with me in the construction. I also show these models in divulgative activities in primary and high schools.
Artworks
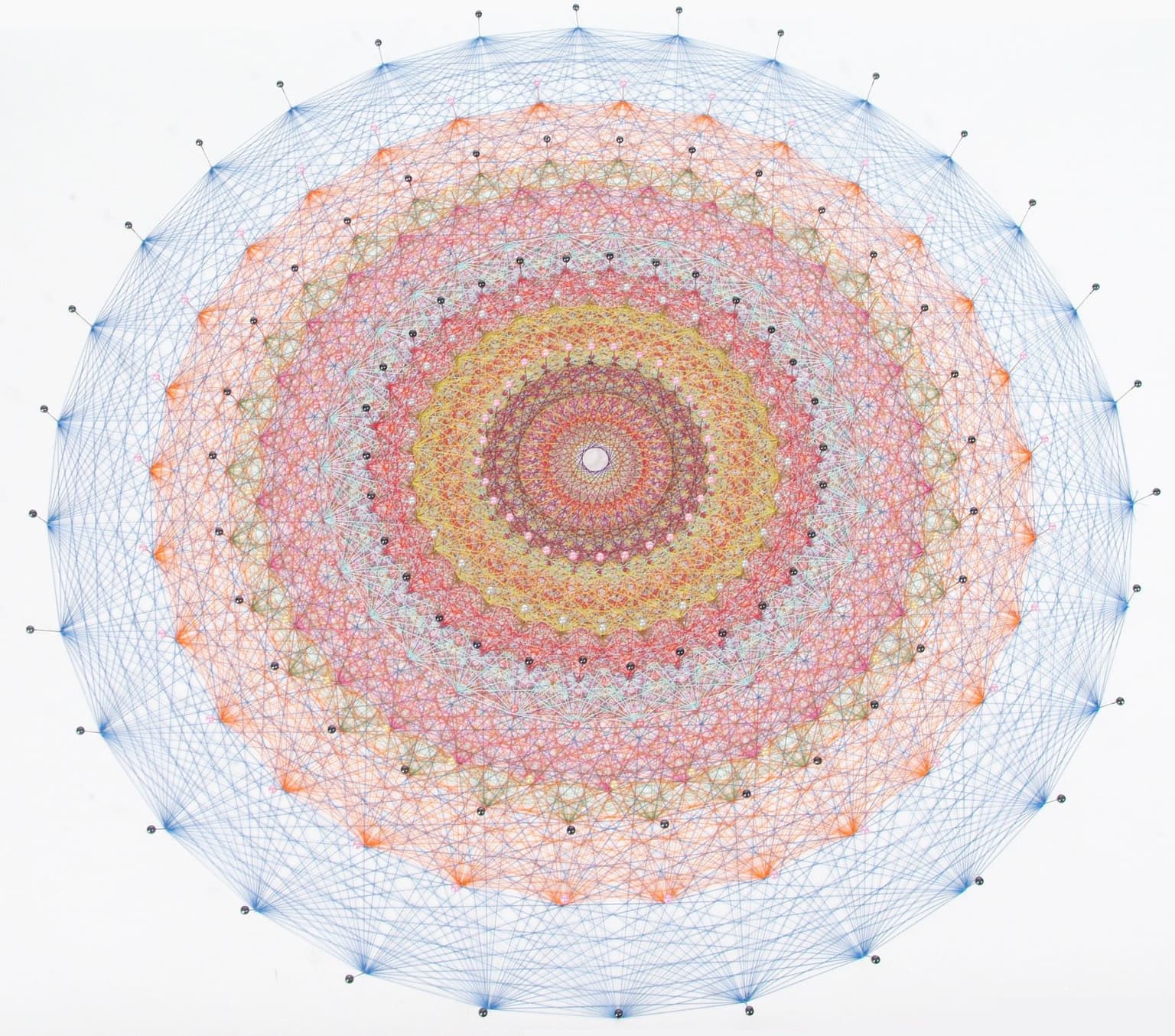
E8 polytope stringy art
100x60x10 cm
Pins and thread on cork
2010
In 1960, Peter McMullen draw by hand this wonderful representation of the Gosset polytope 4_21 (described in 1900). The set of vertices form the root system of the exceptional Lie group E8 (this is why it is also called the E8 polytope). More recently, John Stembridge draw a computer image, from which we have been inspired to make this hand made thread version.
This polytope lies in the 8-dimensional space. Its 1-skeleton consists of 240 vertices and 6720 edges. What we see here is the Petrie projection onto the Coxeter plane displaying a wonderful rotational symmetry of order 30. The 240 vertices are distributed in 8 concentric circles of 30 vertices each, where all vertices in the same circle are connected by straight lines, except the diametrically opposed.
The vertices provide a solution of the kissing number as well as best packing of 7-dimensional spheres. The Lie group E8 occurs in theoretical physics, more precisely in string theory and supergravity.