Elizabeth McTernan and Luke Wolcott
Artists
Elizabeth McTernan and Luke Wolcott
Artist (McTernan); Mathematics Postdoctoral Fellow (Wolcott)
University of Western Ontario (Wolcott)
Berlin, Germany (McTernan); Lisbon, Portugal (Wolcott)
Statement
Elizabeth McTernan, an artist, and Luke Wolcott, a mathematician, have been working together on math, and art, and math-art, for over a decade. Their projects have taken them through the fjords of Norway, across the US, deep into the wilderness of Washington State, along the Trans-Siberian railway in Russia, and out into the Gobi desert in Mongolia. They have brainstormed over cups of coffee in Seattle, NYC, Lisbon, Berlin, Stockholm, Irkutsk, Beijing, and Sendai. Sometimes the artist contributes insight into the creative and non-rational aspects of the process of the mathematician. Sometimes the mathematician provides mathematical consultation on the art pieces. In the last few years, however, they have begun to aim for honest math-art collaborations -- works that engage both contemporary math and contemporary art, in an integration that seeks to transcend and include both.
Artworks
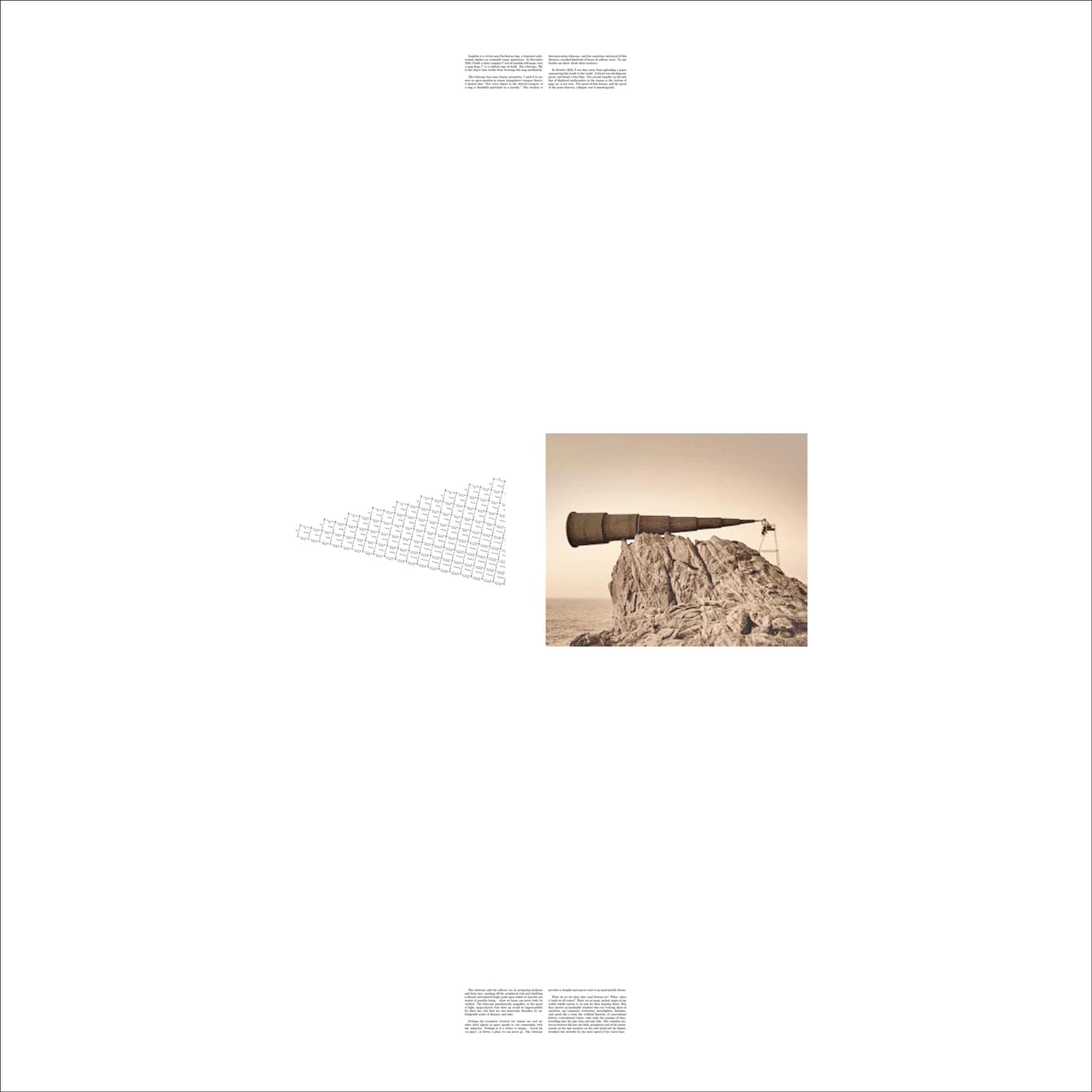
Telescope
24" x 24"
Ink on paper, plexiglass, magnifying dome lens.
2013