Martin Levin
Artists
Martin Levin
Portland, Oregon, USA
Statement
I received a Ph.D. in mathematics from Johns Hopkins. For most of my career I taught high school mathematics in Waldorf schools, where the pedagogy encourages the bridging of mathematics and art. I'm now retired. The Platonic solids are, in a way, quite simple geometric forms, and yet, as one contemplates them and builds up and holds the forms in one’s imagination, they become quite captivating. One can view a cube, for instance, as a bounded solid, but it is more than that. The center point of the figure has a dual (in the sense of projective geometry), which is the plane at infinity. Opposite vertices have a common line that lies on the center point, while opposite faces have a common line that lies on the plane at infinity. One can imagine the form carved out by planes and lines coming in from the infinitely distant periphery. The model shown here is designed to suggest shapes that are not solid blocks, but rather created by lines and planes coming from the periphery.
Artworks
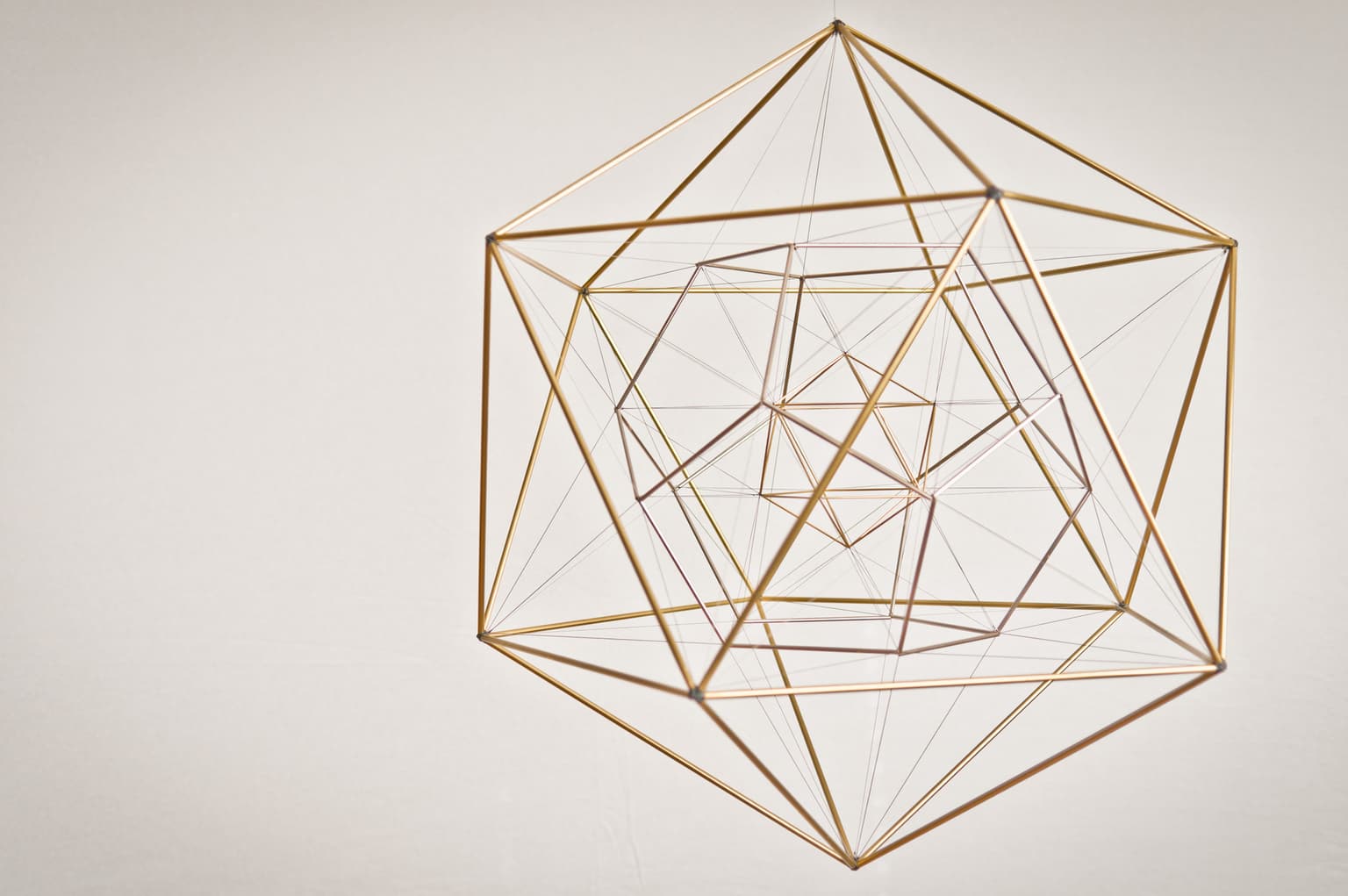
As Above So Below
17" x 17" x 17"
brass and aluminum tubing, beading wire and string, and 3D printed nylon joints
2013