Robbin Juris
Artists
Statement
Although I am not a mathematician or a scientist, I find that motifs that come from these disciplines bring me closest to expressing something meaningful about the unanswerable mysteries of our universe. I am particularly interested in the concept of infinity as it is expressed in the complex patterns and dynamic processes of the natural world.
Artworks
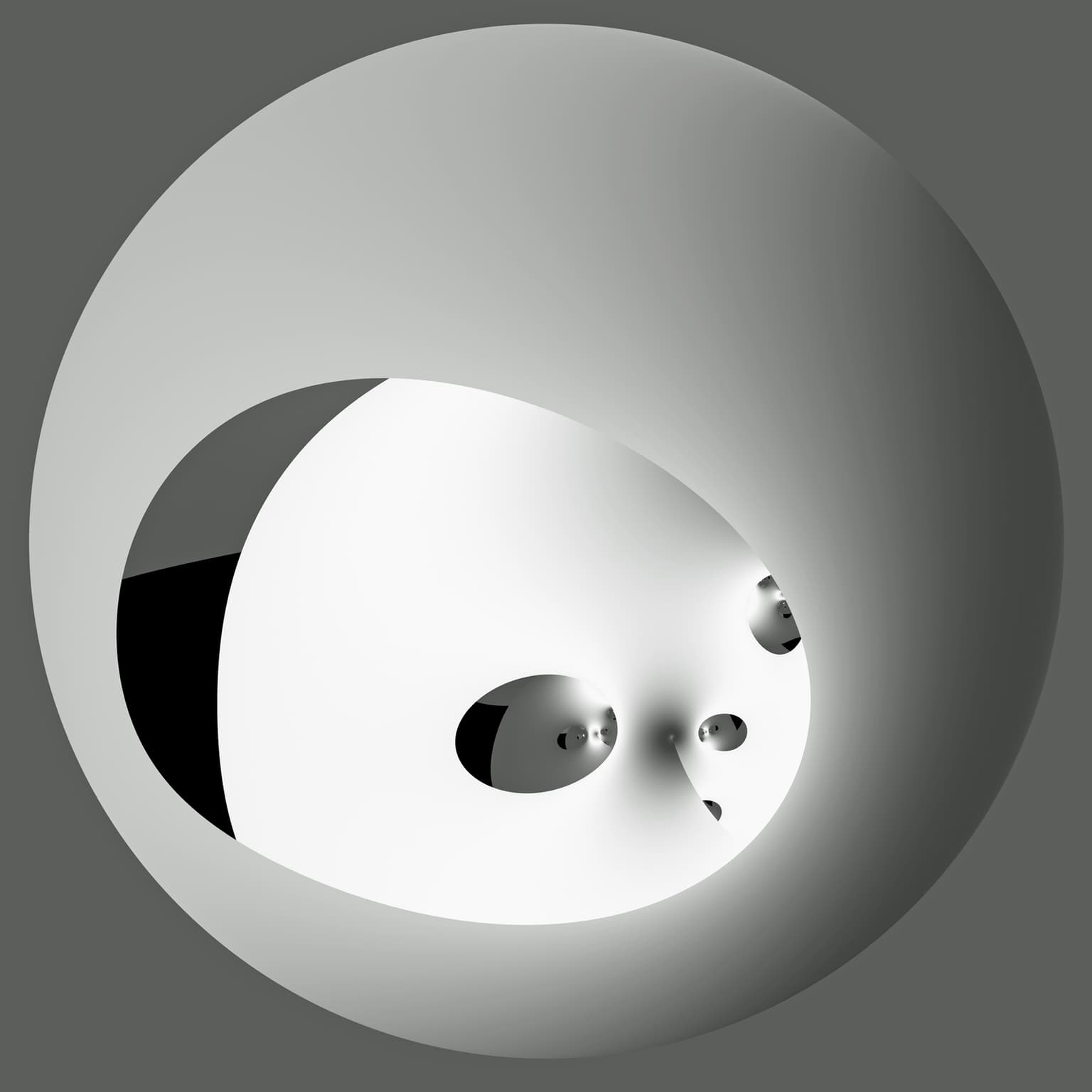
You Are Here, You Are Here, You Are Here,... #FI76I-1a
12 inches by 12 inches
Digital pigment print
2011
In this series of prints, which was inspired by the myth of Daedalus and was exhibited in conjunction with a concert by the Daedalus String Quartet, I use the infinitely recursive properties of fractal geometry as source material to create projective spheres that explore the idea of the world as an infinite labyrinth. The prints share in common shapes that preserve their detailed structure in all scales. Thus, what viewers see on the surface of the print is also what they might see if they could zoom into the print in real time. Paradoxically, the surface smoothness of the spheres belies their inherent roughness and irregularity, which may become apparent at other angles of observation.

You Are Here, You Are Here, You Are Here,... #FI57a-3a
12 inches by 12 inches
Digital pigment print
2011
In this series of prints, which was inspired by the myth of Daedalus and was exhibited in conjunction with a concert by the Daedalus String Quartet, I use the infinitely recursive properties of fractal geometry as source material to create projective spheres that explore the idea of the world as an infinite labyrinth. The prints share in common shapes that preserve their detailed structure in all scales. Thus, what viewers see on the surface of the print is also what they might see if they could zoom into the print in real time. Paradoxically, the surface smoothness of the spheres belies their inherent roughness and irregularity, which may become apparent at other angles of observation.
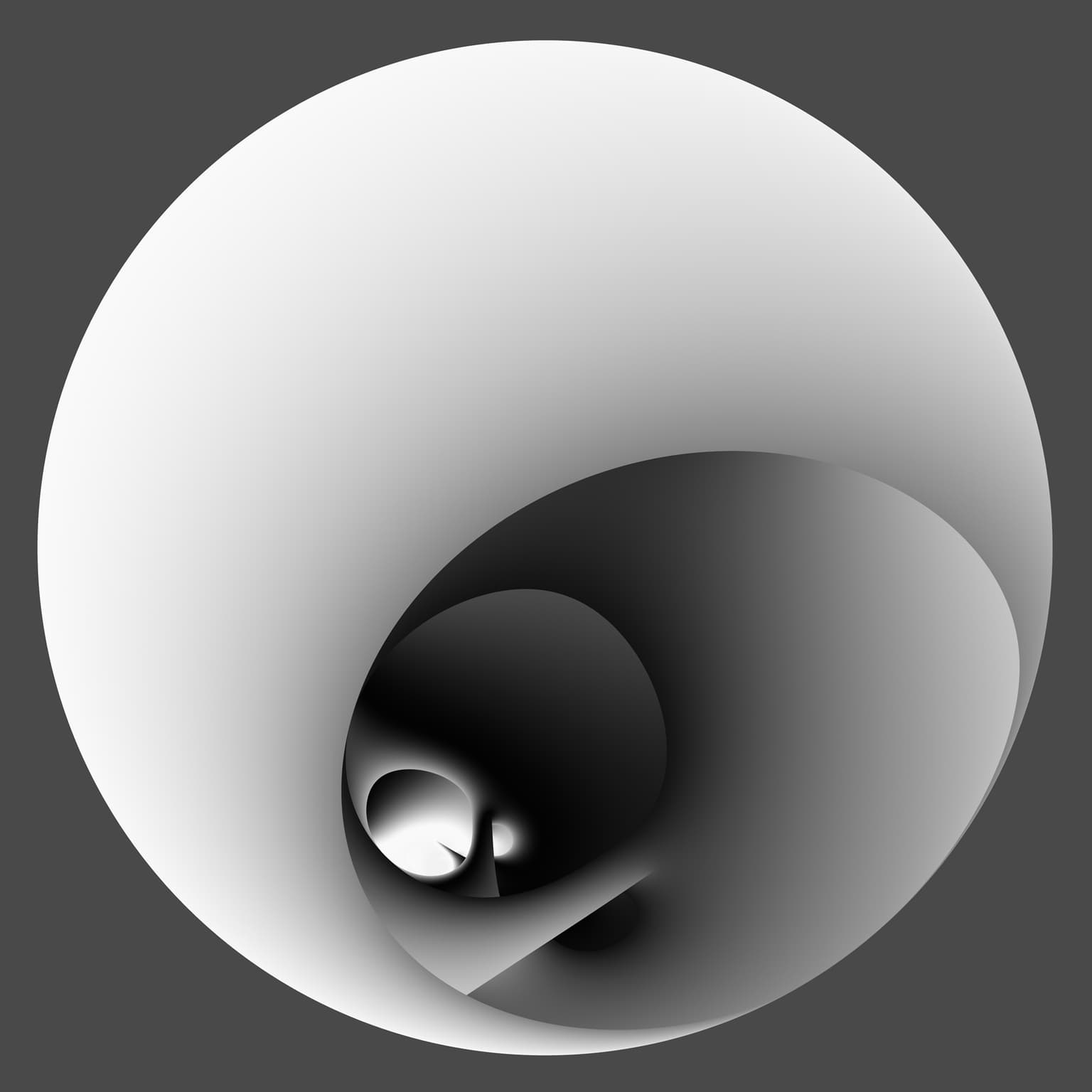
You Are Here, You Are Here, You Are Here,... #FI110a4
12 inches by 12 inches
Digital pigment print
2011
In this series of prints, which was inspired by the myth of Daedalus and was exhibited in conjunction with a concert by the Daedalus String Quartet, I use the infinitely recursive properties of fractal geometry as source material to create projective spheres that explore the idea of the world as an infinite labyrinth. The prints share in common shapes that preserve their detailed structure in all scales. Thus, what viewers see on the surface of the print is also what they might see if they could zoom into the print in real time. Paradoxically, the surface smoothness of the spheres belies their inherent roughness and irregularity, which may become apparent at other angles of observation.