2013 Joint Mathematics Meetings
Horst Schaefer
Artists
Statement
I am trying to apply formal concepts from mathematics, logic or science in my work. One of my goals is to reach a balance between these formal aspects, artistic freedom and the resulting aesthetic appearance. My previous submissions to Bridges consisted of variations of the classical tangram. A Tangram consists of seven pieces: a square, a parallelogram and 5 rectangular, isosceles triangles forming a square. My non-standard tangrams also consists of seven pieces. Every piece is either a square, a parallelogram or a rectangular, isosceles triangle but with different numbers for each piece. Putting them together, they fit into a square. Using these constraints I found 16 non-standard tangrams. One can identify them in the art work by searching for a square consisting of seven pieces.
Artworks
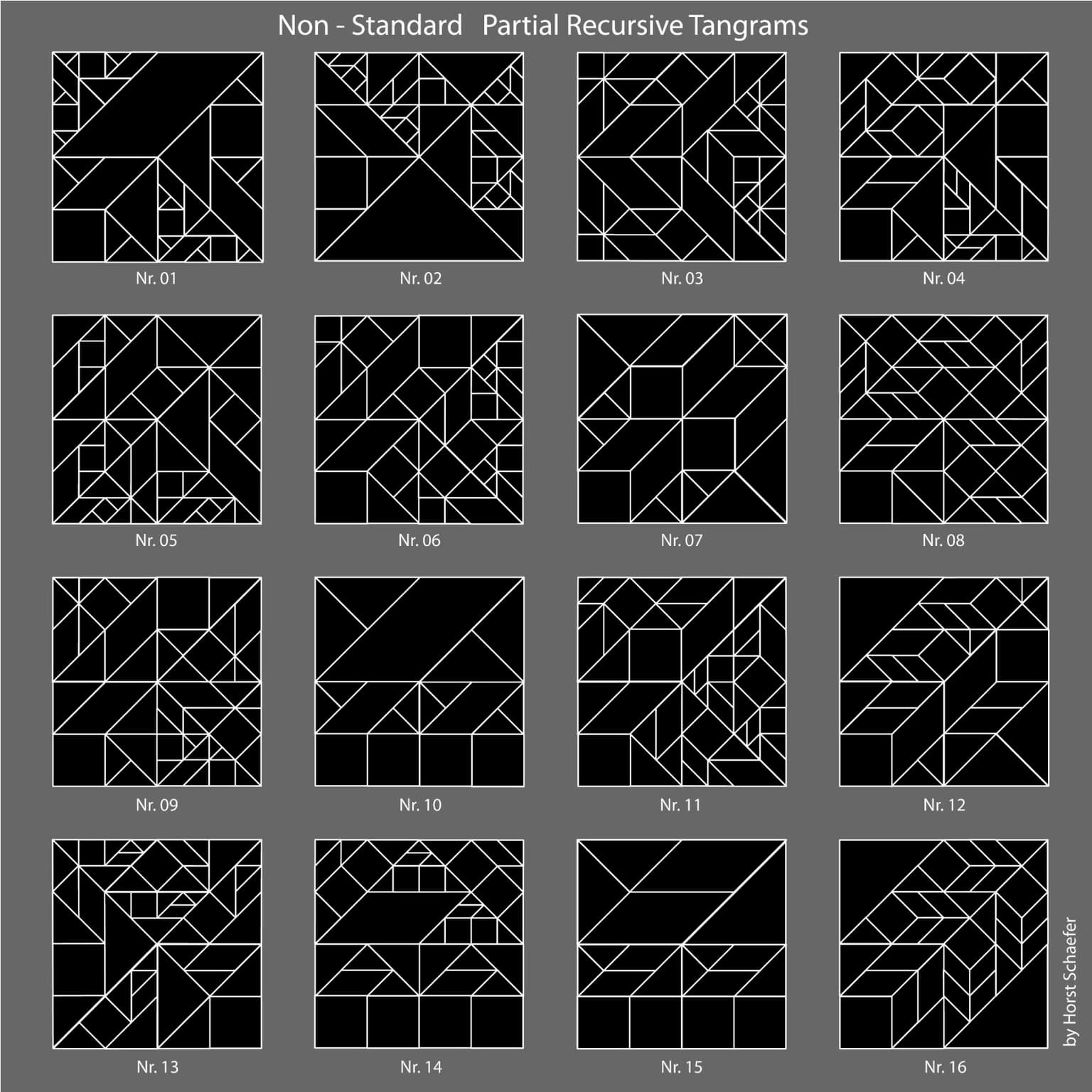
Non-Standard Partial Recursive Tangrams
40 cm x 40 cm
Digital Print
2012