Axel Voigt
Artists
Axel Voigt
Professor of Mathematics
Mathematics Department, TU Dresden
Dresden, Germany
Statement
My artwork is inspired by evolution principles in nature and the richness of the generated nano- and micromorphology. Mathematically modeling such structures using differential equations and visualizing them not only is a reasonable approach in science but also provides the basis for my artwork which is realized using a variity of techniques and materials, e.g. layered printing and 3D printing technologies.
Artworks
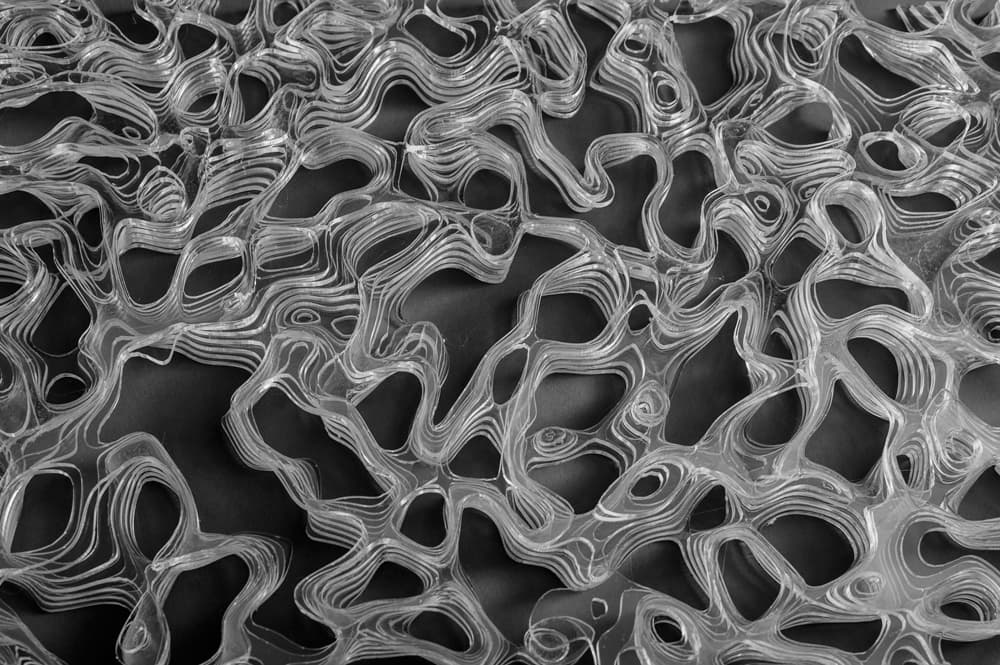
Flow
10 x 80 x 60 in cm
acrylic glass
2014
The evolutionary process of phase separation in a binary fluid is mathematically modeled and numerically solved. Slices of the computed solution are extracted and the interface between the two fluid phases cutted in acrylic glass using a laser cutter. The slices are stacked together to provid a 3D illusion of a bicontinuous structure.
The underlying mathematical model is the Cahn-Hilliard equation, a non-linear partial differential equation, which is solved using the adaptive finite element software AMDiS on a high performance computer.
The art work is a cooperation with ruestungsschmie.de and Wolfram Neumann.

Evolving Bowl
10 x 80 x 20 in cm
3d printed plaster
2013
The driving force for phase separation in vinaigrette is mathematical modeled and numerically solved to generate a bowl, with a morphology governed by its potential use. The morphology evolves in time from finer to coarser structures. The art work shows three snapshots of the evolution, with the oil/water interface 3D printed.
The art work is a cooperation with Sebastian Aland and Florian Stenger.
The underlying mathematical model is the Cahn-Hilliard equation, a partial differential equation describing phase separation in binary systems, which is solved using a diffuse domain approach to account for the geometry of the bowl, and adaptive finite elements on a high performance computer.